2020 Joint Mathematics Meetings
Bernat Espigulé
Artists
Bernat Espigule
Mathematical Researcher & Visual Artist
Departament d'Informàtica, Matemàtica Aplicada i Estadística, Universitat de Girona
Sant Climent Sescebes, Catalonia, Spain
Statement
Bernat lives in Catalonia and works both as a tech consultant and a mathematical researcher at Universitat de Barcelona. This involves spreading his love of maths via research articles, animations, 3D-printed sculptures, workshops, and school visits. Currently, Bernat is interested in exploiting the notion of complex tree to tackle unsolved problems in the field of complex dynamics, and to help develop the theory of analysis on fractals. His research on the theory of complex trees is available at www.ComplexTrees.com
Artworks
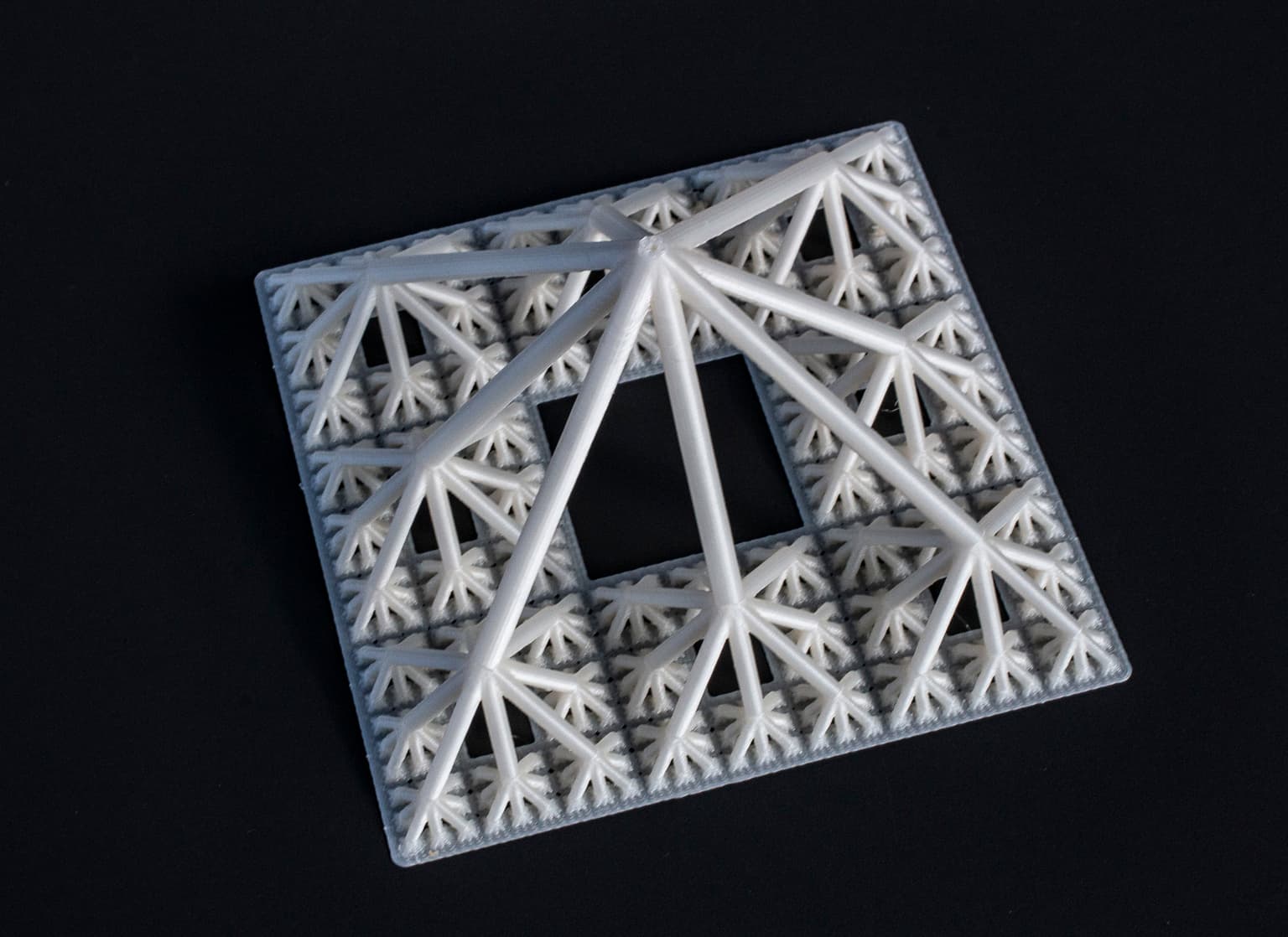
This mathematical sculpture, designed in Mathematica and 3D printed at ICERM's Ultimaker 3, illustrates how the Sierpinski carpet fractal is the limiting set of an octree (each node has exactly eight children). The Sierpinski carpet is a "universal plane continuum", any compact plane curve can be homeomorphically manipulated to fit inside the carpet. Robert L. Devaney has popularized its use as a toy model to study the topology of Julia sets for families of rational maps. The Sierpinski carpet has been also extensively used as a fractal antenna for mobile phones. The 3D tree version designed by the author can be assembled in six copies joint by their initial node to make the external faces of a Menger sponge.
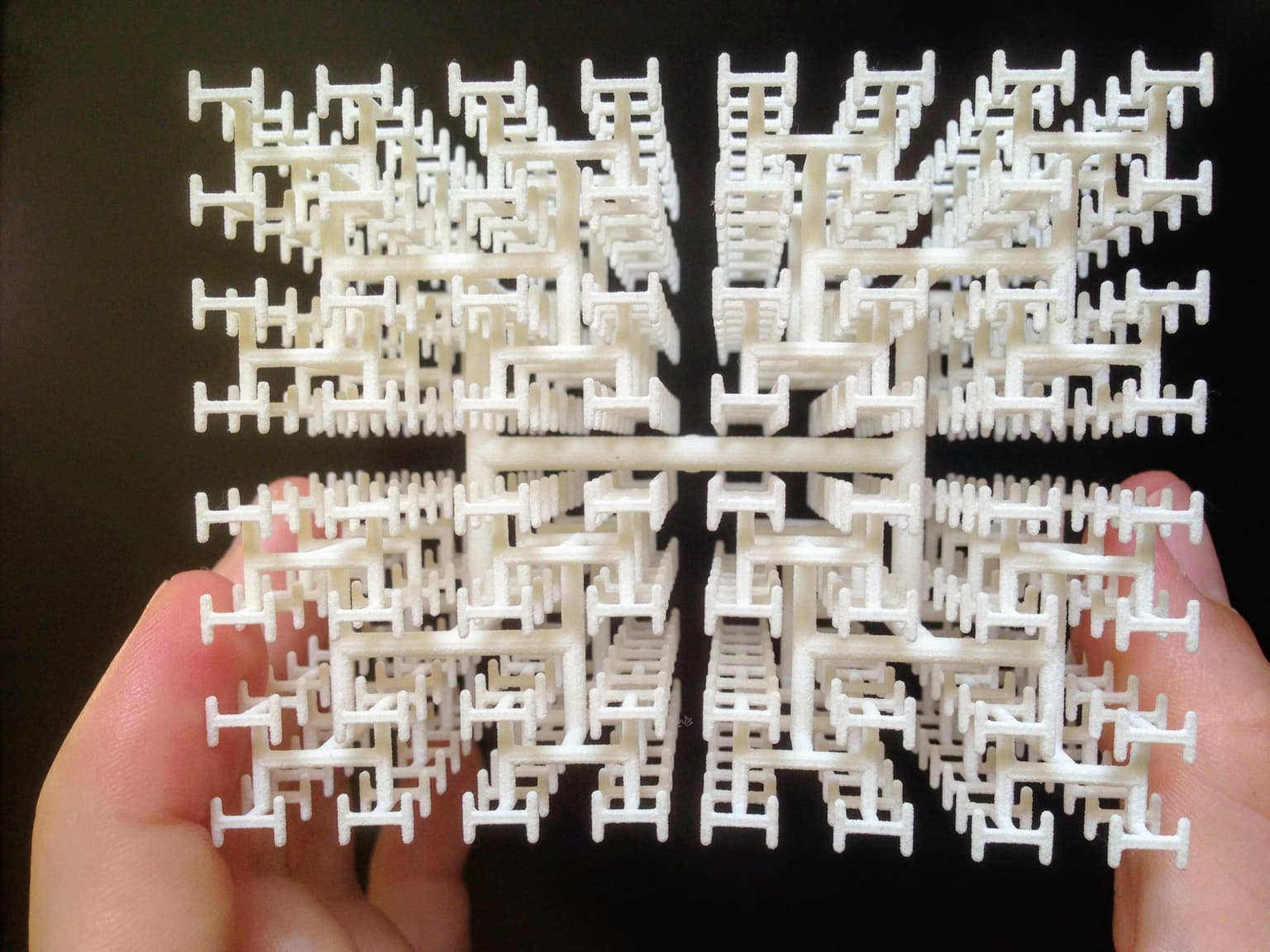
The H-tree in 3D is a space-filling binary tree that has a tipset with Hausdorff dimension D=log(1/2)/log(1/∛2)=3. As a fractal tree it is the three-dimensional equivalent of the plane-filling binary complex tree T{i/√2,-i/√2}, popularly known as the H-tree. This 3D model improves an older version made by the author in 2013. For this new design "Cylinders" were replaced by Mathematica's "Cuboids" following exactly Leonardo da Vinci's rule, i.e. the sum of the cross- sectional area of all tree branches following a branching point at any level is equal to the cross-sectional area of the branch prior that branching point.