2020 Joint Mathematics Meetings
Ian Sammis
Artists
Ian Sammis
Site Reliability Engineer
YouTube
San Bruno, California, USA
Statement
Creating mathematical art gives me a wonderful excuse to explore parts of mathematics well outside my specialty. The effort to make a mathematical idea aesthetically pleasing forces me to understand the underlying mathematics differently and more completely than I otherwise would.
Artworks
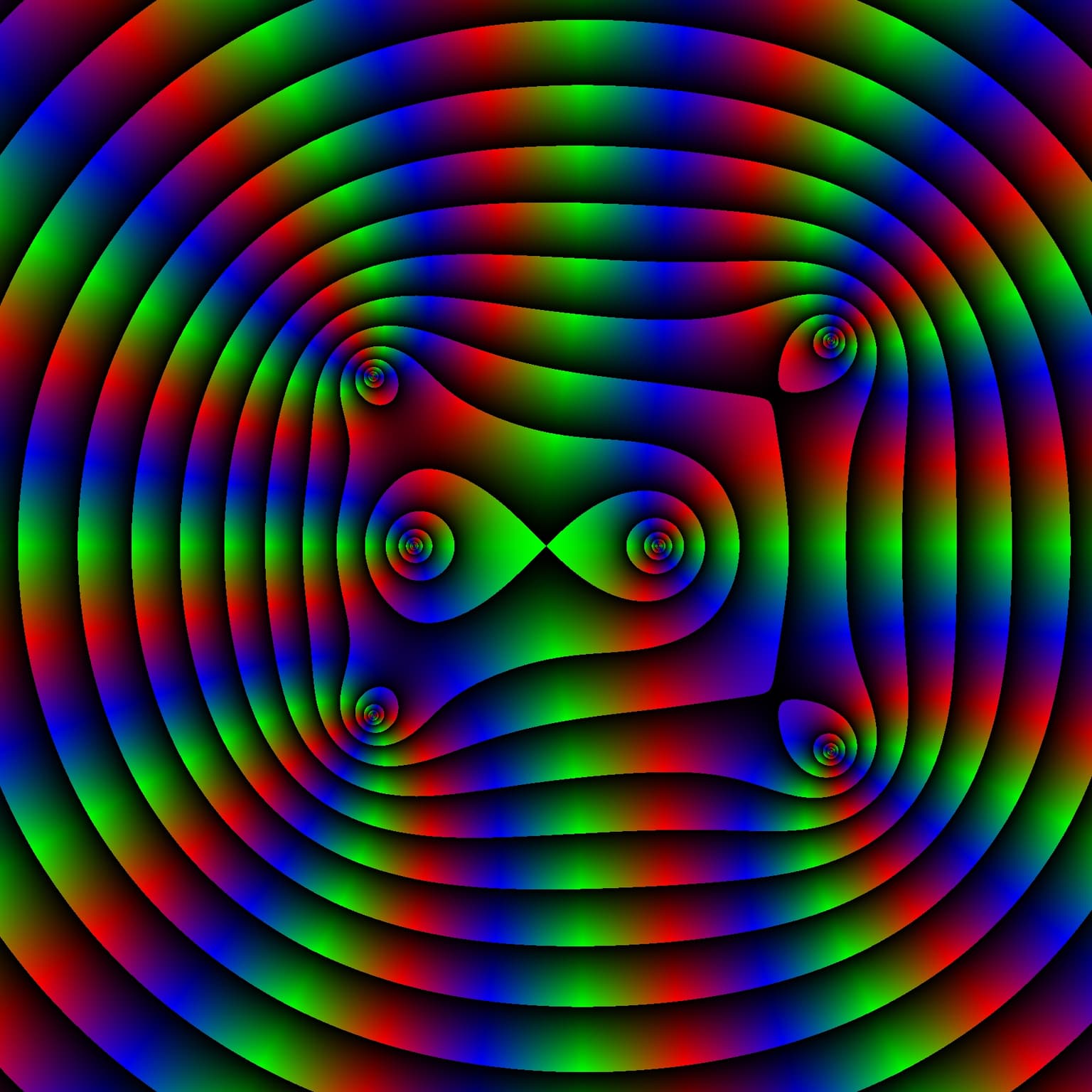
Lissajous Sextic Root Dance
26 x 26 cm
Digital Animation (on an iPad in an endless loop)
2019
Complex polynomials are often depicted simply by their roots, but there's a lot of structure beyond that that's frequently lost! I've always wanted to see the flow of the roots as the coefficients shift (particularly in the standard basis, where the relationship between the numbers and the structure is somewhat whimsical). This movie was a relatively successful attempt.
In this movie, brightness is computed by the fractional part of the base 2 logarithm of |P(z)|, where P(z)=z^6 + az^5 + bz^4 + cz^3 + dz^2 -1, where a, b, c, and d follow a 4-dimensional Lissajous curve in a region where P(z) switches between two and four real roots. The color represents complex phase. The animation loops after 60 seconds.