2020 Joint Mathematics Meetings
Jean Constant
Artists
Statement
Mathematics is based on sound reasoning and repeatable proof. On the opposite side of the spectrum art is considered by some as a spontaneous and subjective form of expression. Yet, both use images to communicate. My current research involves exploring the reasoning and visual aspect of abstract mathematics to investigate the connection between maths and art and provide a platform for people from all different backgrounds and cultures to explore ideas that encourages reflection and self reflection.
Artworks
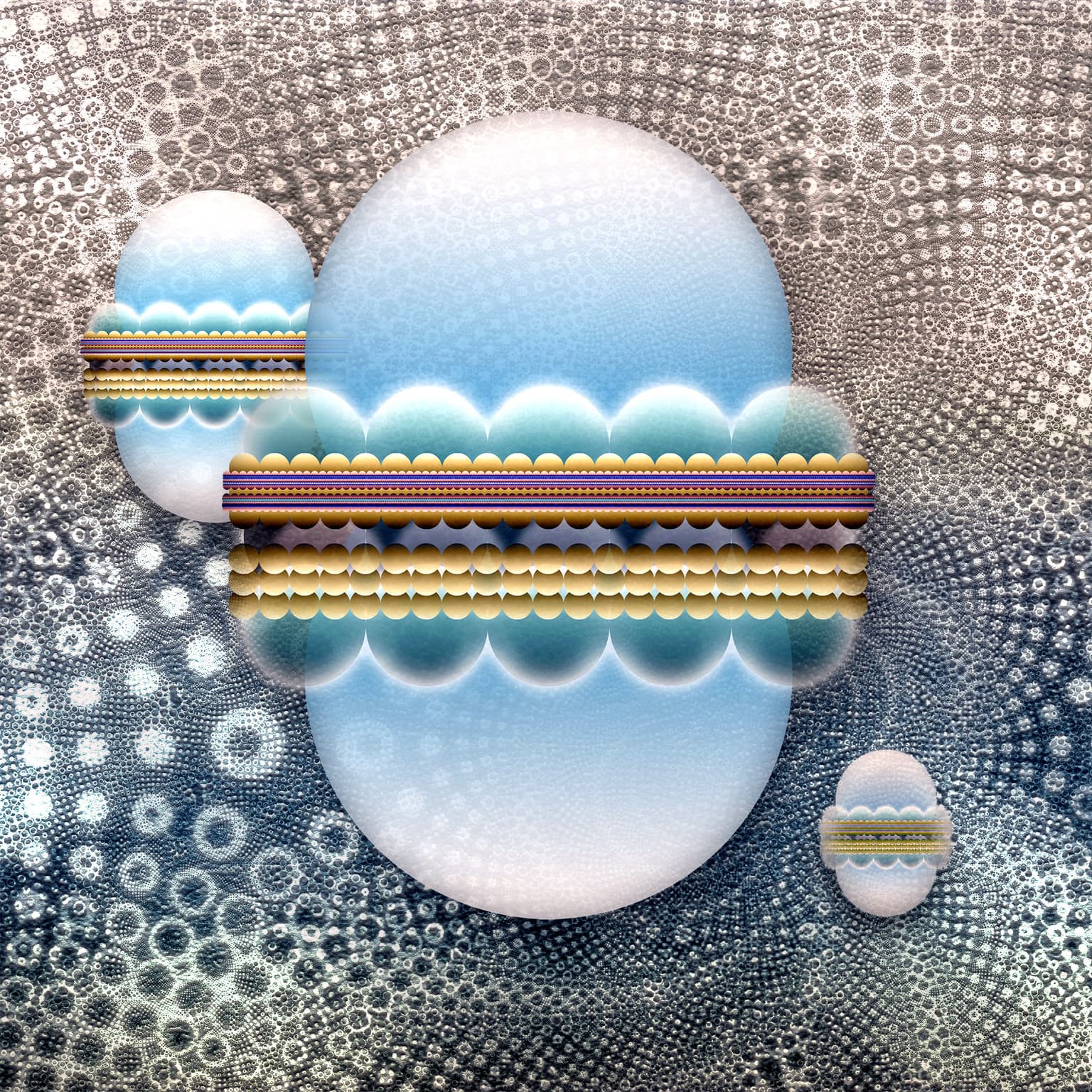
Congruent circles Sangaku
60 x 60 x 2 cm
Mixed media ink on Dibond-Aluminium
2019
Sangaku originating in a problem proposed by Shinohara in 1809 and developed at length by Okumura in the Sangaku journal of Mathematics (2017). The challenge consists in finding the ratio of the radii on the circles of the center line to construct several recursive configurations consisting of congruent circles on a line.
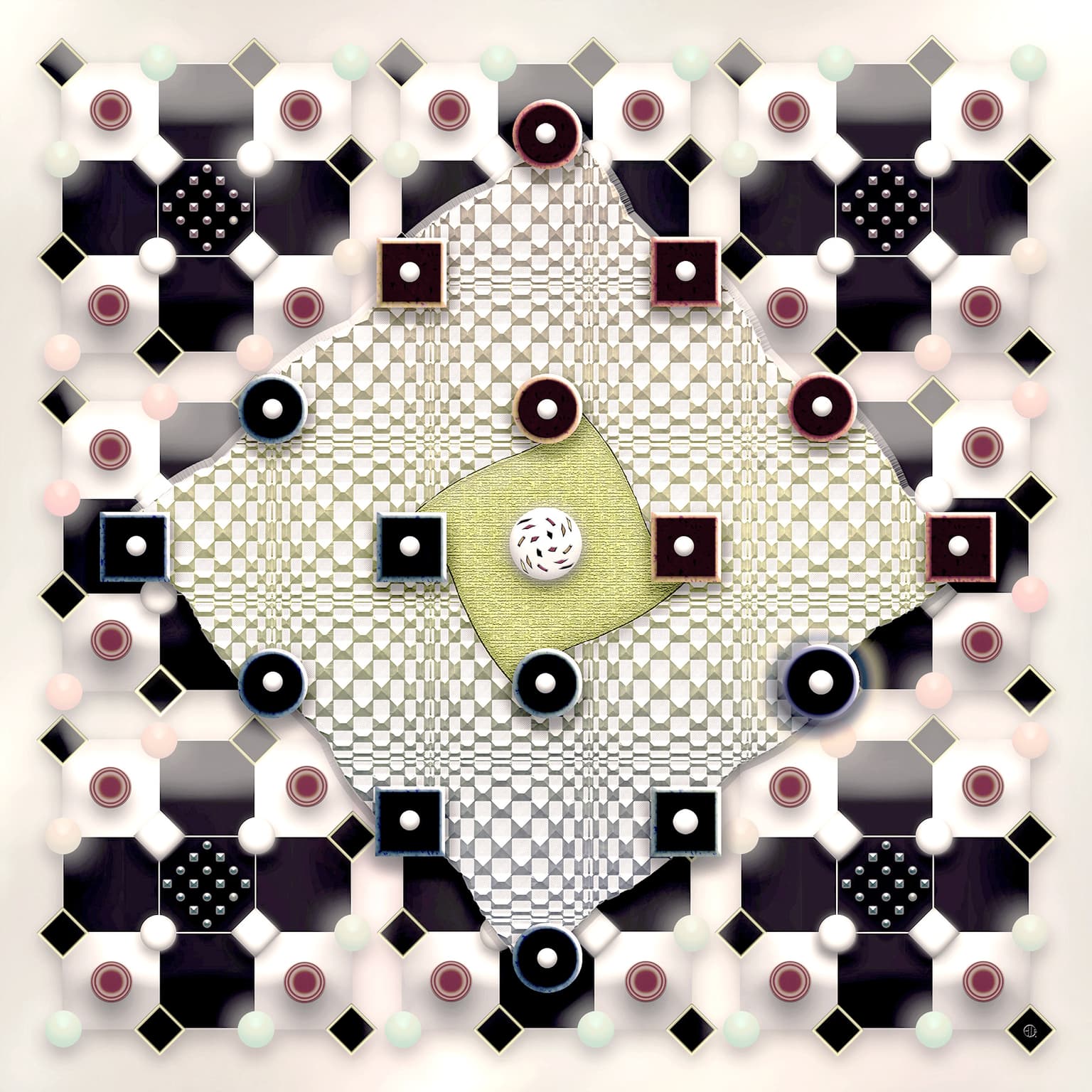
The shortest route problem
60 x 60 x 2 cm
Mixed media ink on Dibond-Aluminium
2019
Mathematicians and statisticians use graphs to study and understand randomness. However, graphs are seldom explored in the visual arts. They can be inspirational, fun, and a good conversation starter. This iteration of the shortest route problem was composed after Dr. Porteus textbook on stochastic processes (2002). The nodes on the central grid are replaced by numbers, 1 on the left, 16 on the right, 7 on top and 10 at the bottom. Using a recursive method, the shortest distance from node 1 to node 16 is 15, and the shortest route is along nodes l-3-6-10-13-15-16. I slightly enhanced the key position 15 to guide the viewer's eye toward the best possible solution.