2020 Joint Mathematics Meetings
Robert Spann
Artists
Statement
I have always been fascinated with the behavior of dynamic systems -- be they mathematical, physical, or financial. Where do they end up and how do they get there? Do they reach an equilibrium or do they continue moving randomly forever? Computer graphics allows one to see both the numerical and aesthetic properties of these systems. Recently I became interested in rational polynomials with one parabolic (or neutral) fixed point and n symmetrically arranged fixed points. I have been exploring their mathematical properties and using those properties to design images.
Artworks
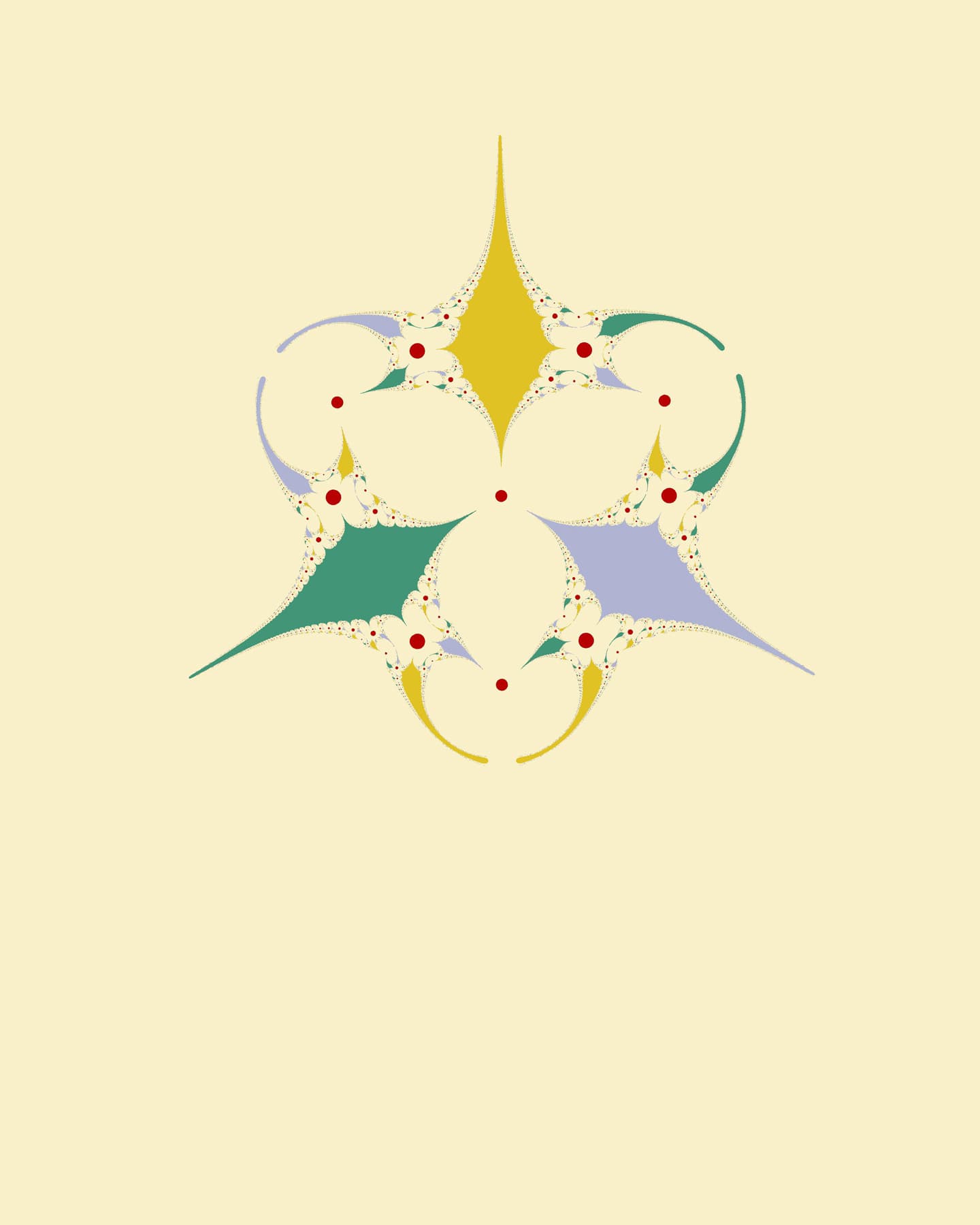
Kites and Darts
50 x 40 cm
Digital Print
2019
Kites and Darts is obtained by iterating the function f(z) = .5(z+z^4)/(.5+z^6) . Each point is assigned a color based on which fixed point that initial point converges to after 100 iterations. This function has one parabolic (or neutral) fixed point at the origin and three super attracting fixed points. The automorphisms of this function form a group that is isomorphic to the group of symmetries of an equilateral triangle. Another interesting property of this function is that infinity is not a fixed point. Thus it is not conjugate to a polynomial, nor is it conjugate to a Newton map.

Red and Black No. 137
50 x 40 cm
Digital Print
2019
Red and Black No. 137 is obtained by iterating the function f(z)=a(iz - z^4)/(a + z^6) with a = 4.016 - 4.003i. Each point is assigned a color based on which fixed point that initial point converges to after 100 iterations. This function has one parabolic (or neutral) fixed point at the origin and three attracting fixed points. The automorphisms of this function form a group that is isomorphic to the group of symmetries of an equilateral triangle. Another interesting property of this function is that infinity is not a fixed point. Thus it is not conjugate to a polynomial, nor is it conjugate to a Newton map.