2020 Joint Mathematics Meetings
Rodrigo Treviño
Artists
Rodrigo Treviño
Assistant professor
University of Maryland, College Park
College Park, MD
Statement
The study of aperiodic tilings lies at the intersection of several areas of mathematics such as dynamical systems, topology, discrete geometry, and operator algebras, to name a few. Their origins go back at least a thousand years, where they have appeared in artwork in medieval Islamic architecture in many countries throughout the world. The best known aperiodic tilings, such as the Penrose tiling, are ones which are self-similar. Aperiodic tilings which are constructed from random applications of different substitution rules have begun attracting serious attention recently. One of the tilings here is constructed from applying different Penrose-like substitutions at random.
Artworks
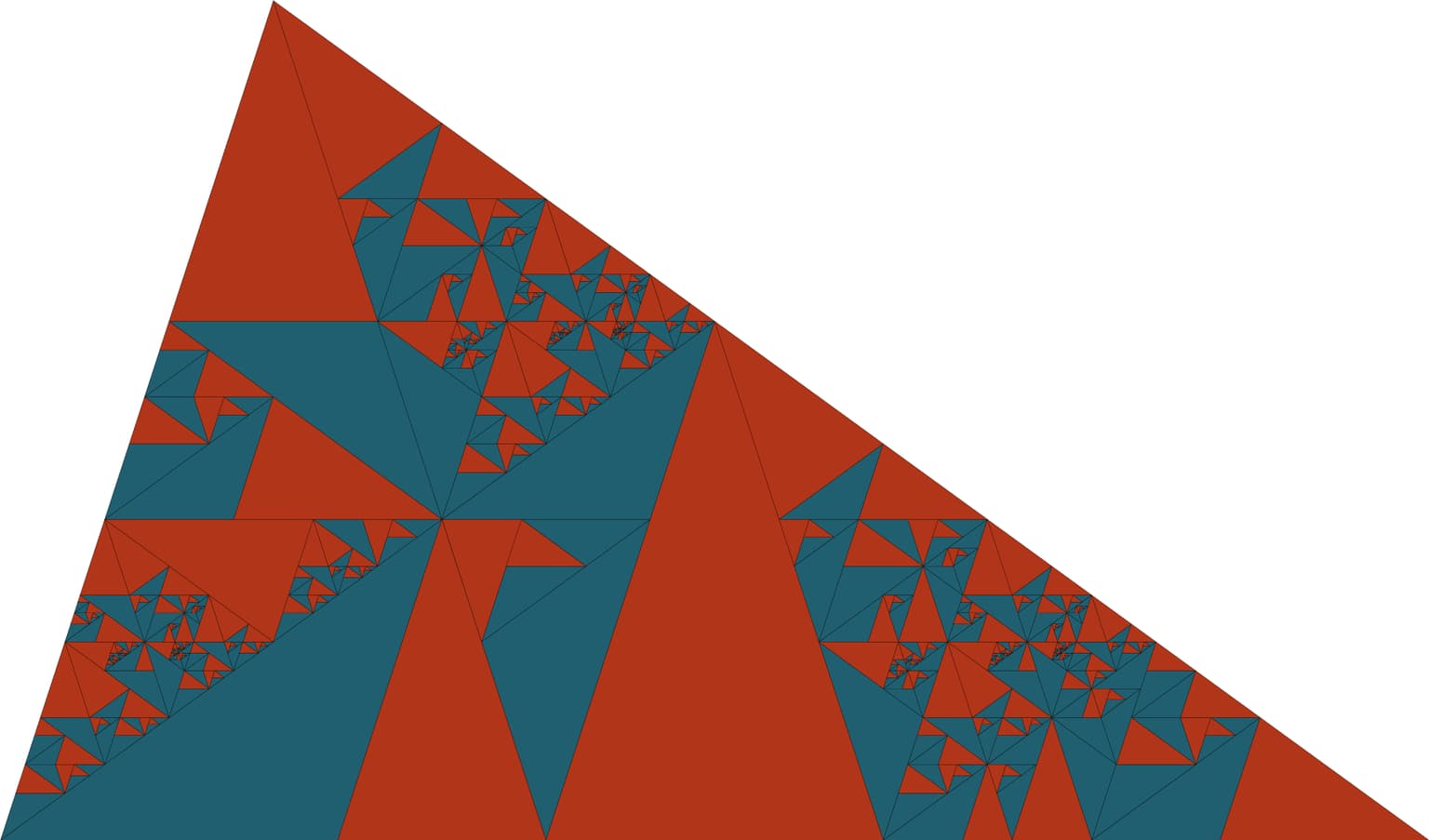
The March
45 x 70 cm
Archival paper print
2019