Winnie Leung

Statement

I am an origami artist specialising in tessellations. Since uniform-sized, straight-lined origami tessellations and fractals are now fairly common, I want to explore designs that are a bit different. While playing with star polygon tessellations from Grunbaum's tessellation book last year, I discovered a simple algorithm that creates star-based origami corrugations. The algorithm seems to work on any convex polygon tiling, although the end result may or may not have zero curvature. So far, I have applied the algorithm to a number of Archimedean and hyperbolic tilings, as well as the Hirschhorn Medallion. It would be interesting to see if there exists any pattern between the base tiling and the curvature of the end results.

Artworks

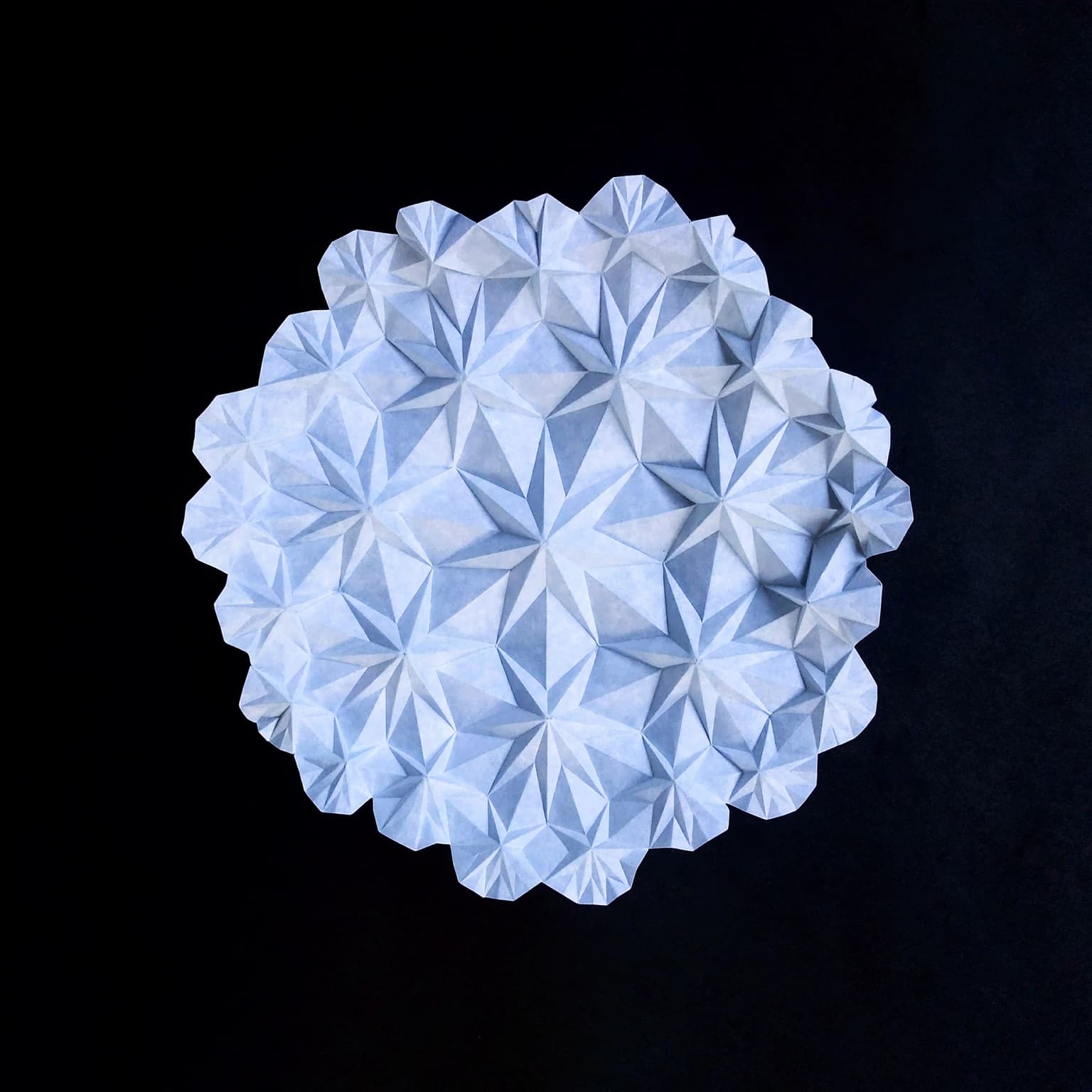
This work is inspired by Robert Lang's 3^7 Hyperbolic Limit model. Using the dual of the 3^7 hyperbolic tiling on a Poincare Disc as a base, the vertices of the tiles are joined together in straight lines to form irregular heptagons. Foldable star shapes are then created within each heptagon, and connected. A light coloured paper (Skytone) was chosen to accentuate the contrasting light and shadow of the design. The crease pattern is then machine scored on the paper and hand folded to form the final model.