Carlo Sequin
Artists
Carlo H. Séquin
Prof. Emeritus
EECS Department, University of California, Berkeley
Berkeley, California, USA
Statement
I am interested in mathematical knots as constructivist sculptures. Prime knots cannot exhibit mirror symmetry or any of the symmetries of the regular polyhedra. Still, it is possible to make tubular sculptures based on prime knots that overall display the structure of some Platonic or Archimedean solid. To do this, I run the knot strand along all the edges of such a polyhedron, forming a closed Eulerian circuit with as much symmetry as possible. Near the vertices, the knot strand is deformed slightly to avoid any direct strand intersections. These deformations, necessary to obtain a true knot, will further reduce the overall symmetry; but the overall shape of the knot will still represent the shape of the underlying polyhedron.
Artworks
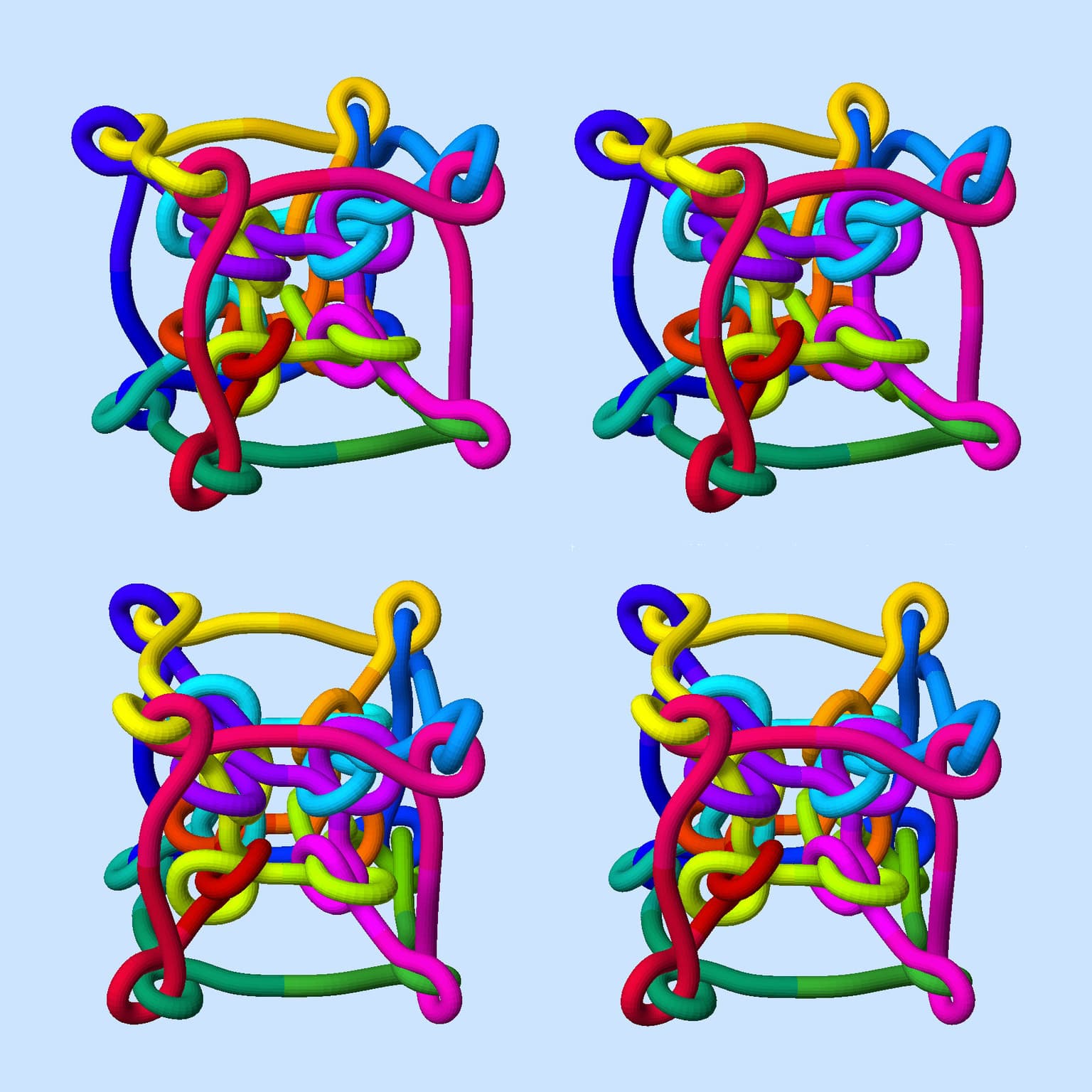
Hypercube Knot
18 x 18 cm
Computer Graphics
2021