Dan Bach

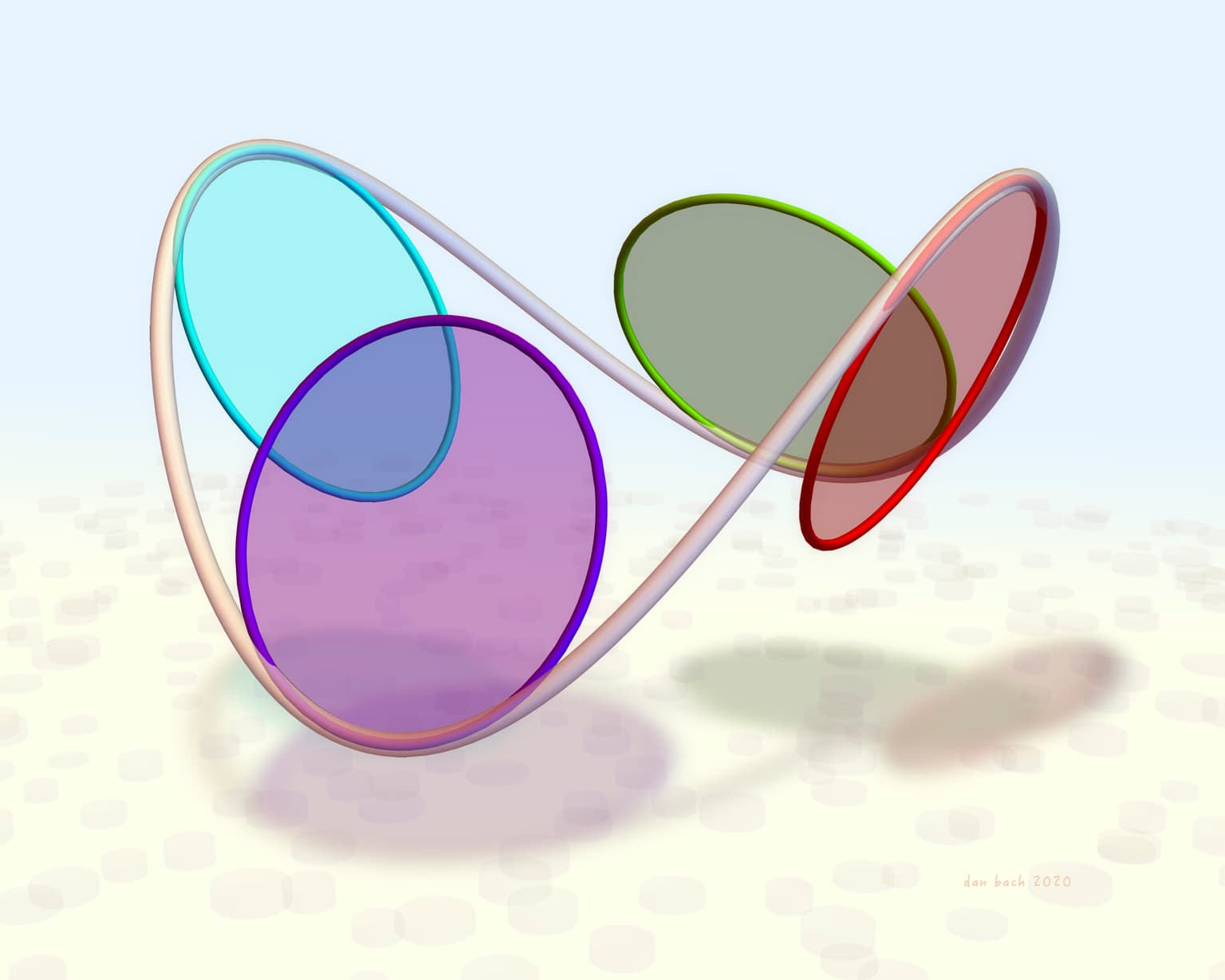
I love playing with space curves. Any smooth parametric curve has a unit tangent vector at each point, going in the same direction as the curve. The rate of change of this vector, with respect to arclength, gives the curvature at that point, and we can construct an 'osculating circle' tangent to the curve and having the same radius of curvature locally. The four circles shown here are the smallest ones along this saddle curve. Try to picture other osculating circles along this curve! (click the link for an interactive 3D experience!)

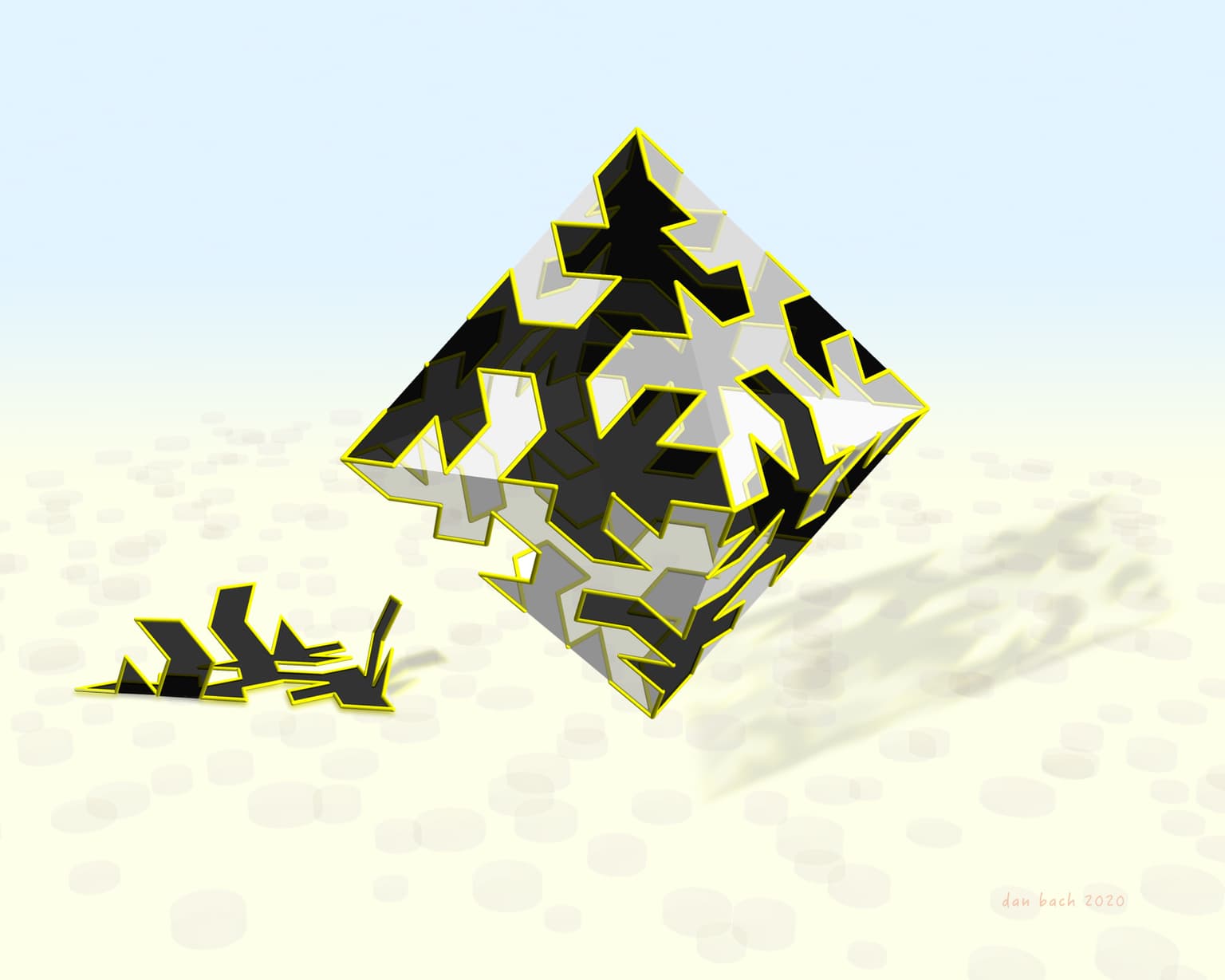
In my attempt to draw a path starting at the origin and going through every lattice point in space, I decided on nested octahedral layers, each defined by |x| + |y| + |z| = n, with n = 0, 1, 2, 3, ... An Eulerian circuit traces all edges of each octahedron once, and these can be filled out zig-zag style, to pass through all the points on the layer, as in the traveling salesman problem. The seventh layer is shown here; it turns out all the paths from one vertex to the next have the same shape, and this creates a 'zigsaw puzzle' with one piece for each face of the octahedron. The stray piece wants to be reattached to that hole in the side. Will it fit? (follow the link for a 3D version!)