2021 Joint Mathematics Meetings
Francesco Mancini
Artists
Statement
I'm an eclectic origami designer: I like to create modular polyhedra and stars but also animals, boxes, and multi-piece tessellations. Since I discovered origami I liked the possibilities it gave me to translate in a physical form lots of concepts and objects of mathematics. I work as a guide in a math museum and paper-folding is a very useful tool to show the beauty and fun of geometry (or arithmetics or algebra) to kids of all ages.
Artworks
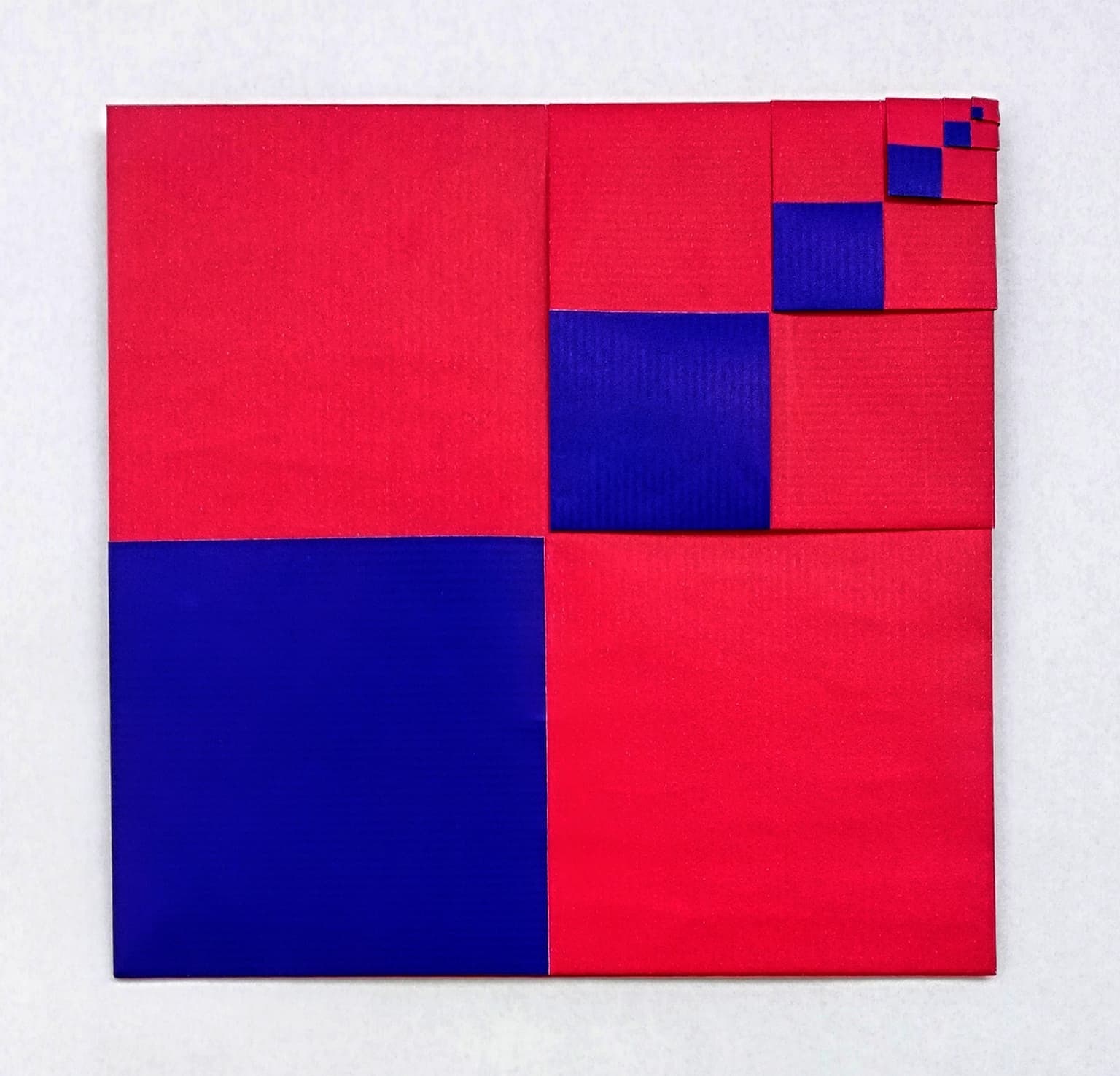
In proofs without words the emphasis is given to visual clues to stimulate mathematical thought. I made this origami version with 6 rectangles in the ratio 1:sqrt2. From the second piece I folded the others from a bicoloured sheet that was 1/4 of the previous one. The resulting model shows a sequence of decreasing blue squares. Each one is 1/4 of the previous and represents a term of a geometric series of the reciprocals of powers of 4. To the right and above of every blue square there is a red square with the same area. Together they are two more copies of the blue sequence. All three fill the whole square so the area of the blue sequence (and, in the limit, the sum of the series 1/4+1/16+1/64..) is 1/3. In the link a model with triangles.

This is a modular origami made from 12 units. I folded each unit from an uncut regular pentagon, and I assembled them without glue. The finished unit has the shape of a pentagonal antiprism, a polyhedron composed of two parallel pentagons joined by a ring of triangles. I named this model Anti-Dodecahedron because one of the pentagonal faces of the antiprism lies on one face of a regular dodecahedron. In the link, there is a 6 unit Anti-Cube starting from squares.