2021 Joint Mathematics Meetings
Ian Sammis
Artists
Ian Sammis
Site Reliability Engineer
YouTube
San Bruno, California, USA
Statement
Creating mathematical art gives me a wonderful excuse to explore parts of mathematics well outside my specialty. The effort to make a mathematical idea aesthetically pleasing forces me to understand the underlying mathematics differently and more completely than I otherwise would.
Artworks
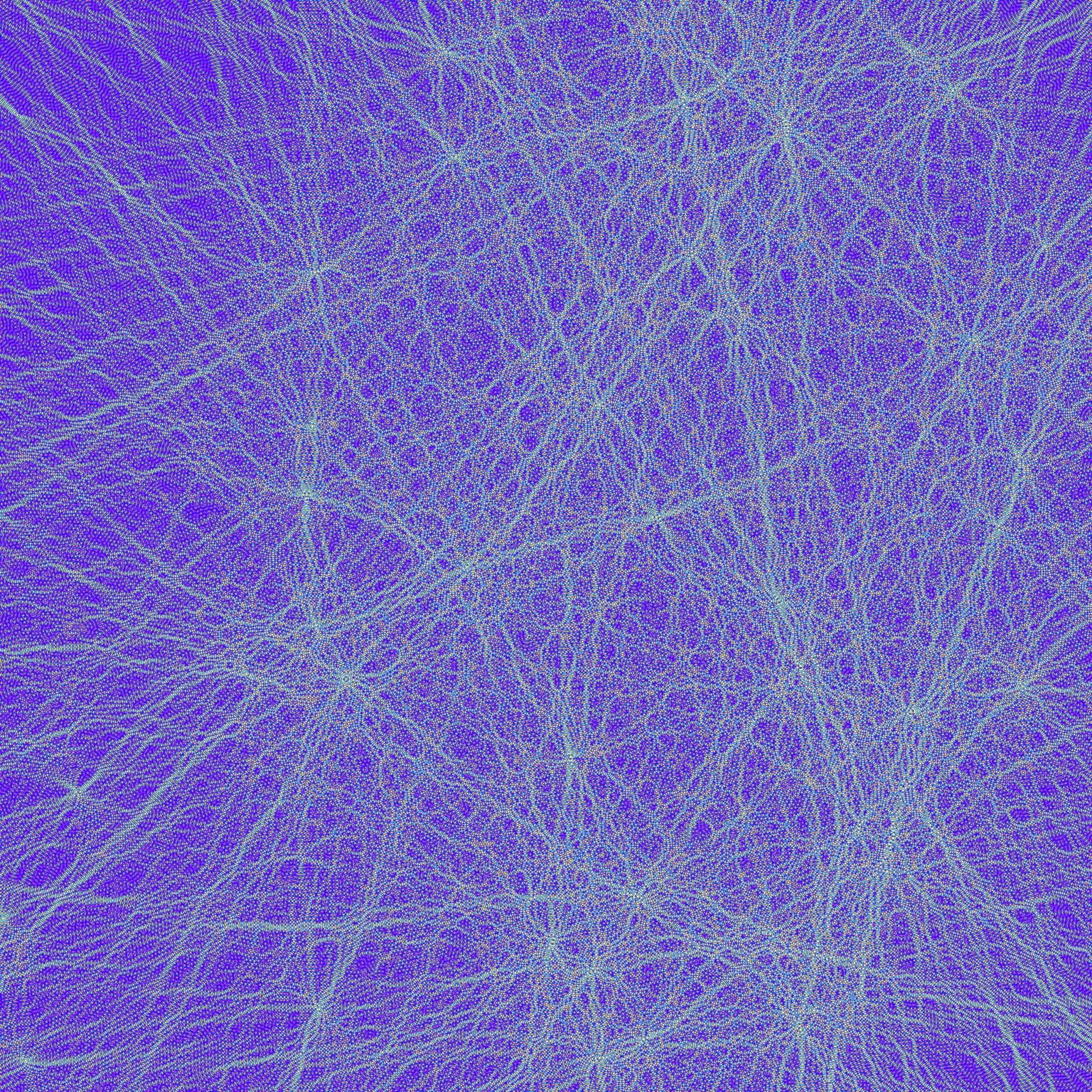
Bessel oil fringes
0 x 41 x 41 cm
Digital print on canvas
2020
50 radially symmetric bessel function perturbations were placed on a depth function representing a thin film of oil. The resulting interference patters in each wavelength were convolved against models of the response of the cones of the eye to create RGB values. Since the most visually striking aspect of the result was the interference fringes, the color balance was drastically altered to highlight them, resulting in a complex, web-like structure.
Interference pattens have always struck me as visually beautiful, and I've been fascinated for some time by the way a structured system tends to create visual beauty out of randomized inputs.