2021 Joint Mathematics Meetings
Regina Bittencourt
Artists
Regina Bittencourt
Software Developer and Mathematical Artist
MuArt; APECh; ESMA
Santiago, Chile
Statement
Even though our culture has two separate disciplines for mathematics and art, I feel that mathematics is of unique intrinsic beauty. And it is what I wanted to show when doing each artwork: to express the beauty of a curve, the game of algorithms and how the organization of numbers can form algebraic surfaces. I want to discover the beauty of mathematics.
Artworks
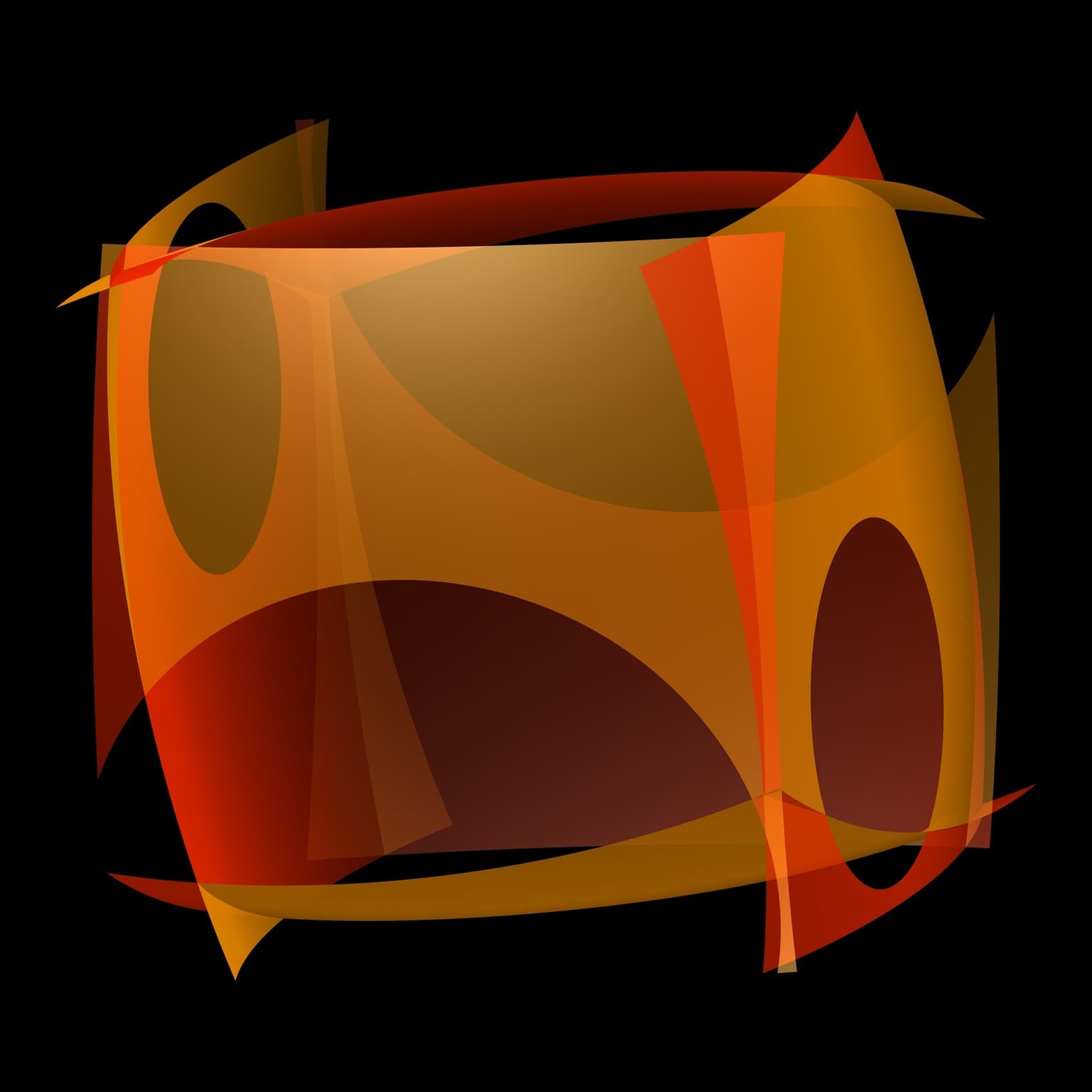
The surfaces inside an imaginary cube
100 x 100 cm
Giclée on museum canvas
2020
The image shows the interior of several algebraic surfaces and was generated from the following polynomial equations:
(0.2*x^2+0.2*y^2+z^2-1)*(0.2*x^2+0.2*z^2+y^2-1)*(0.2*z^2+0.2*y^2+x^2-1)=0
(z-2)*(z+3)*(z+2)*(z-4)*(x-2)*(x+3)*(x+2)*(x-4)*(y-2)*(y+3)*(y+2)*(y-4)=0
Once the algebraic surfaces were generated, they were cut on the outside to fit inside an imaginary cube. This allowed the image to show the interior of the surfaces.

The Beautiful Buildings of a Squared Square
50 x 50 cm
Acrylics on canvas
2020
This artwork presents a Simple Imperfect Squared Square of order 13.
It is a square that contains 13 squares; some of them are of the same sizes which makes it imperfect. And no subset of the squares forms a rectangle or a square; that characteristic makes it simple.