2022 Bridges Conference

Diana Davis and Samuel Lelièvre

Artists

Diana Davis and Samuel Lelièvre

Instructor in Mathematics / Maître de conférences

Phillips Exeter Academy / Laboratoire de mathématiques d'Orsay

Exeter, New Hampshire, USA / Orsay, France

https://dianadavis.github.io/

https://www.imo.universite-paris-saclay.fr/~lelievre/index-en.html

Statement

We are research mathematicians studying billiards and flat surfaces. Since 2014, we have been working together to study periodic billiard paths on regular polygons, starting with the regular pentagon. We wrote a program in Sage to draw pictures of the paths, which turn out to be really beautiful. We have made our pentagon pictures into jewelry and T-shirts, to bring the beauty of mathematics to more people. We just (in February 2022) added the ability to draw paths on tables with an even number of sides, such as this 32-gon, so we're especially excited about exploring the paths on such tables and understanding their properties.

Artworks

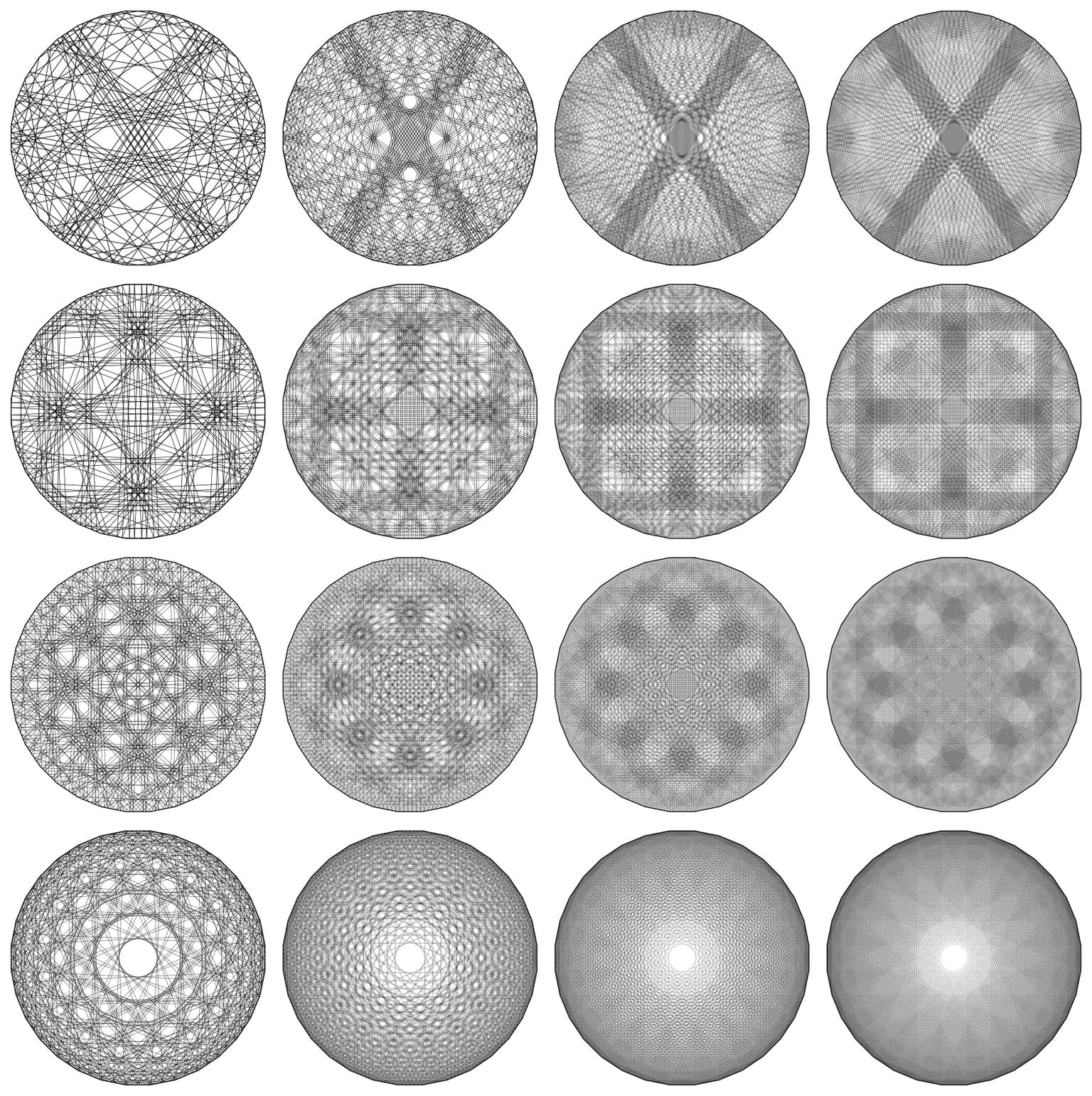
Image for entry 'Families of periodic billiards paths on the 32-gon'

Families of periodic billiards paths on the 32-gon

50 x 50 cm

Inkjet print of computer graphics

2022

Imagine that you have a billiard table in the shape of a regular polygon with 32 sides. The picture shows 16 different periodic (repeating) paths of a billiard ball on such a table. Each horizontal row has a different symmetry: dihedral symmetries of order 2, 4, 8 and 16, respectively. The paths in each row are members of what we call a "family" of periodic trajectories: They get longer and longer, but they make a similar pattern. We get subsequent family members by repeatedly "twisting" the surface associated to this billiard table. In the limit, you can't see the lines anymore; it has become a shading pattern. We have found that these families are visually appealing; people seem to like the contrast between light and dark regions.