David Plaxco
Artists
David Plaxco
Assistant Professor
Clayton State University
Morrow, Georgia, USA
Statement
David Plaxco is an assistant professor in the Mathematics Department at Clayton State University. He has been exploring alternative solutions to n x n x n Rubik's cubes for five years in an ongoing project called the Cubes Underscore Art project. With the project, David has identified several algorithms that can be used to precisely manipulate pieces of the n x n x n cube to generate pixel art.
Artworks
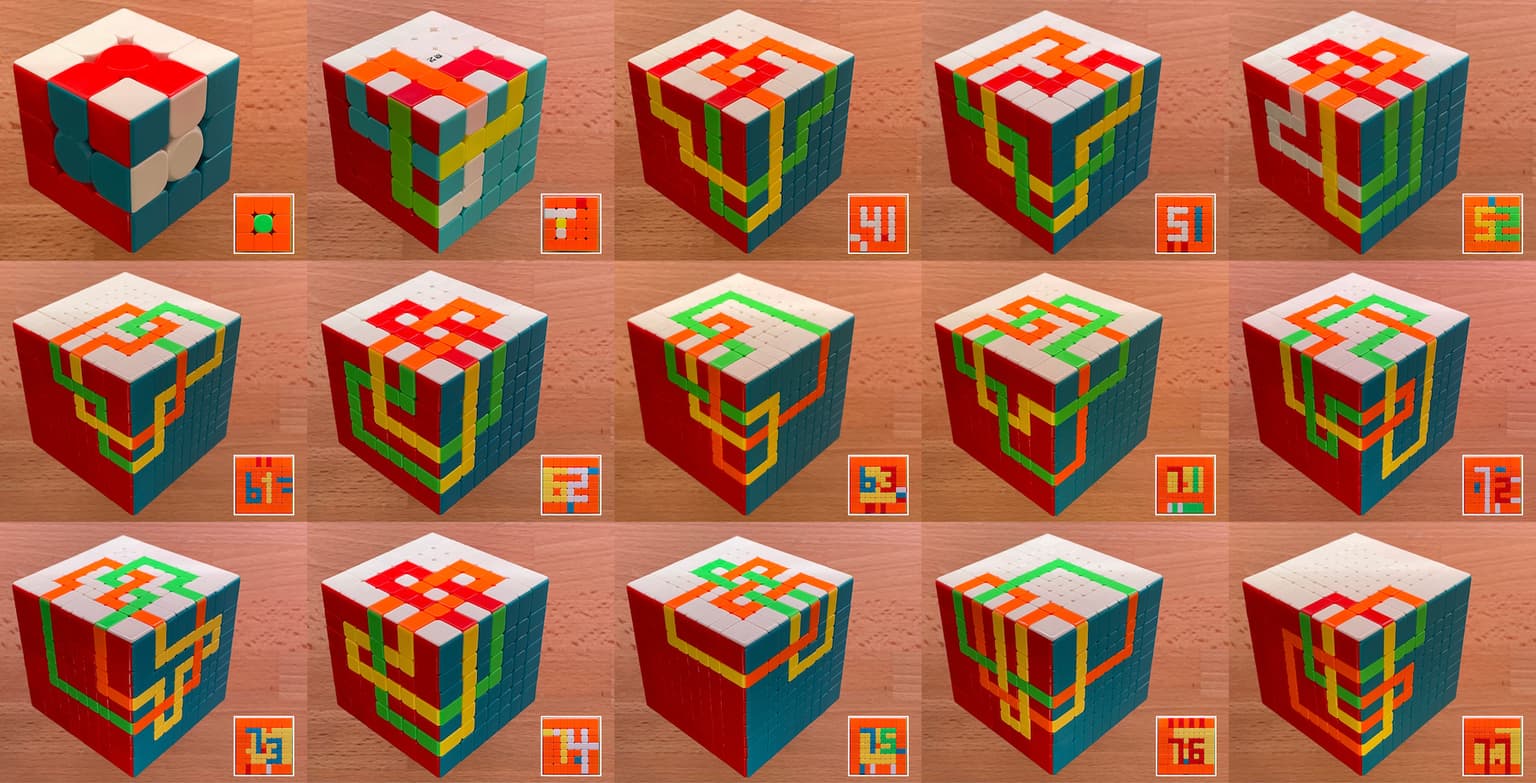
Knots on n×n×n Rubik’s cubes
7 x 50 x 50 cm
15 Rubik's cubes ranging in size from 3×3×3 to 9×9×9
2022
This piece is a collection of 15 Rubik's cubes that have been solved to alternative solution states. These states show all knots through 7 crossings. Each knot is shown on three faces of a cube so that the entire knot is visible from a single perspective (in this collection, the white/blue/red corner). I solved each cube's orange face (inset) to indicate the knot. For each knot, the number of layers of the cube is minimized and then the number of colors is minimized (as far as I have been able to achieve by submission). I call these pixelated projections of knots "photogenic" because they depict segments of the knot on each face as a solid color that contrasts with the background and at crossings and also leaves a gap between segments.