Peter Hilgers
Artists
Peter Hilgers
Artist
private
Ettlingen, Germany
Statement
I am a retired engineer, computer hobbyist, naive artist and have always been fascinated by geometry. Current computers and sophisticated programs make it possible to create, study and visualise complex structures. For me this is a constant source of challenges and delight.
Artworks
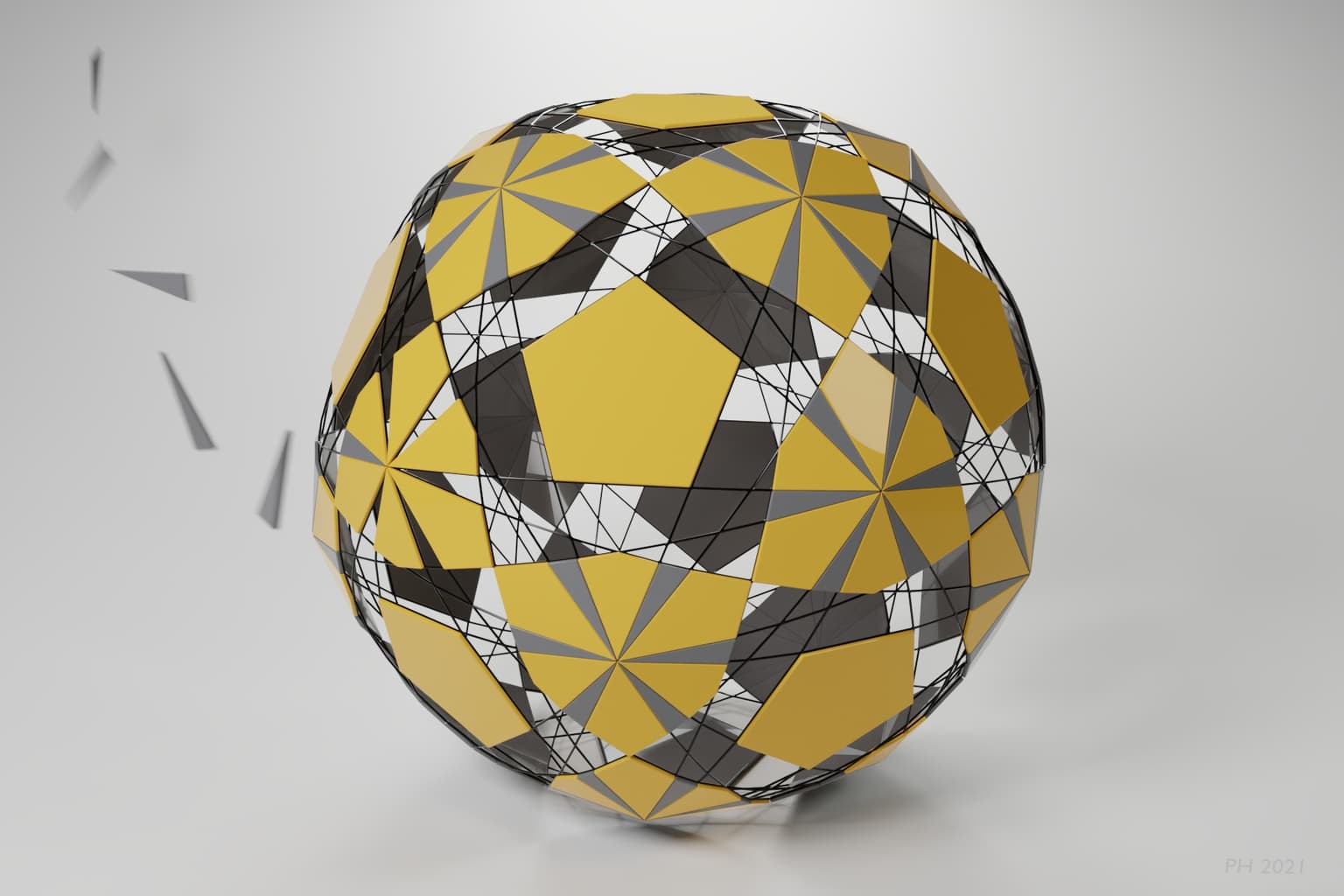
Late arrival
40 x 60 cm
Digital print, created with Mathematica and Blender
2021
The topic of this artwork is a playfully treated feature of non-periodic tilings called growth form. Growth forms are a kind of neighbourhood relation for 2D or 3D tilings: Let a patch P of a tiling be a finite set of tiles. Under recently found general conditions [1] the nth-generation "immediate neighbours" of P, scaled by 1/n, form a defined polygon or polyhedron in the limit.
Here the tiling is derived from 30 vectors related to an octahedron 5 compound and the growth form is a convex polyhedron with icosahedral symmetry, 590 vertices, 1260 edges and 672 faces.
[1] Demski, D., Hilgers, P. & Shutov, A.: Grow forms of grid tilings, Acta Crystallographica, Volume A78, Part 4, 1 July 2022, https://doi.org/10.1107/S2053273322003485