Carlo Séquin

Statement

For the last couple of years, I have been interested in taking a small domain of the 2D Gosper curve and transforming it into a corresponding 3D sculpture. Such a sculpture should be constructed from a set of equal-length tube segments that form a single circuit. Consecutive segments should form bending angles of 60° or 120° as found in the planar Gosper curve. Ideally, the construction should be based on a recursive procedure, where simple elements of the Gosper curve get replaced by a scaled-down version of a larger sub-assembly found in the curve. However, such an ideal procedure that fills all of 3D space has not been found yet.

Artworks

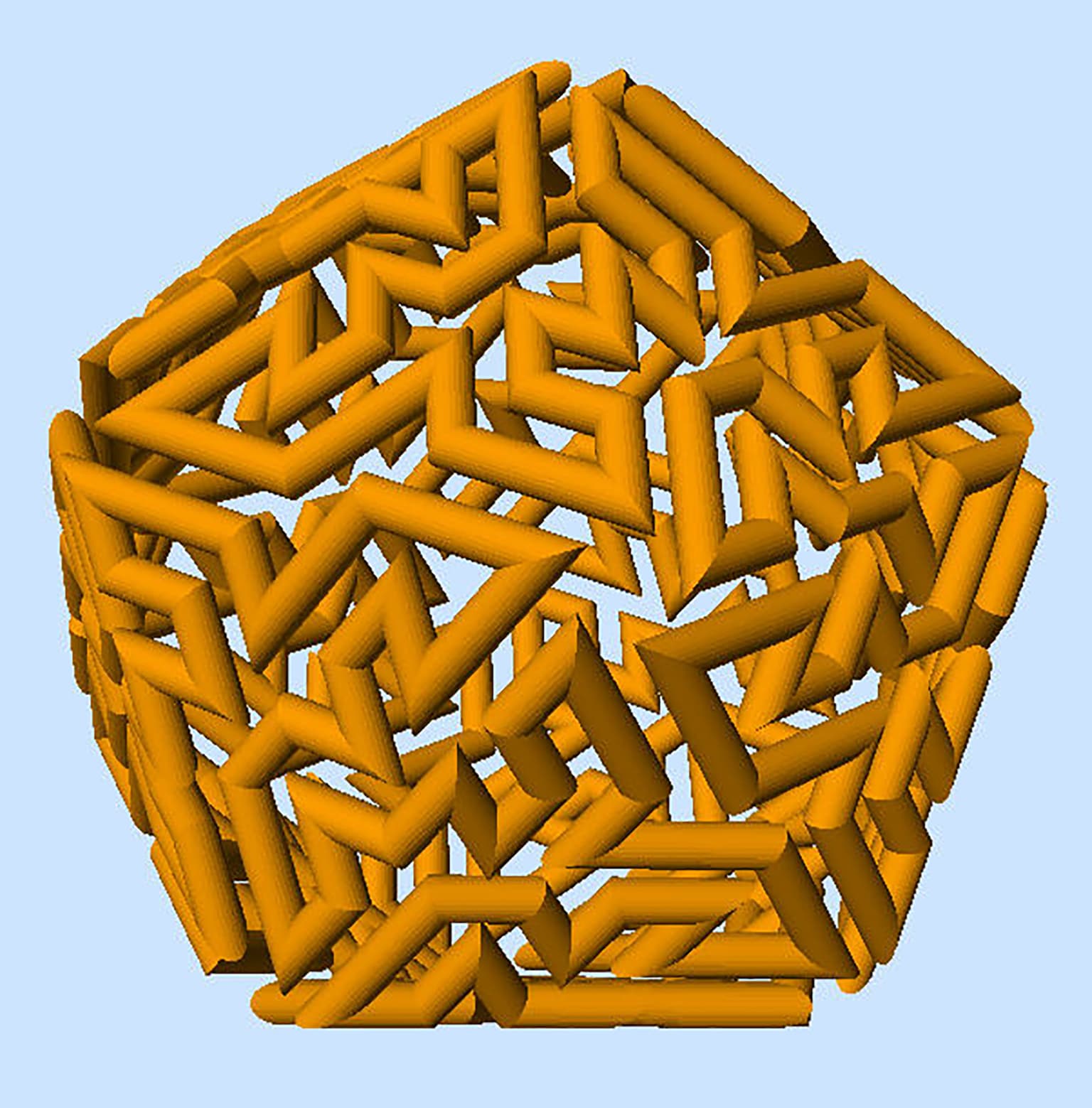
In “Gosper-Icosahedron_480” I am covering just a small amount of 3D space, and I am replacing the objective of a recursive procedure with an aim for high symmetry. First, I designed a Gosper-like pattern that fills an equilateral triangle, with entry- and exit-points of the Gosper path on two different sides of the triangle. Then I string together 20 of these elements to cover the whole surface of an icosahedron. The sequence in which the 20 triangles are visited is given by a Hamiltonian path on the dual of the icosahedron – the dodecahedron.