Christopher Hanusa

Statement

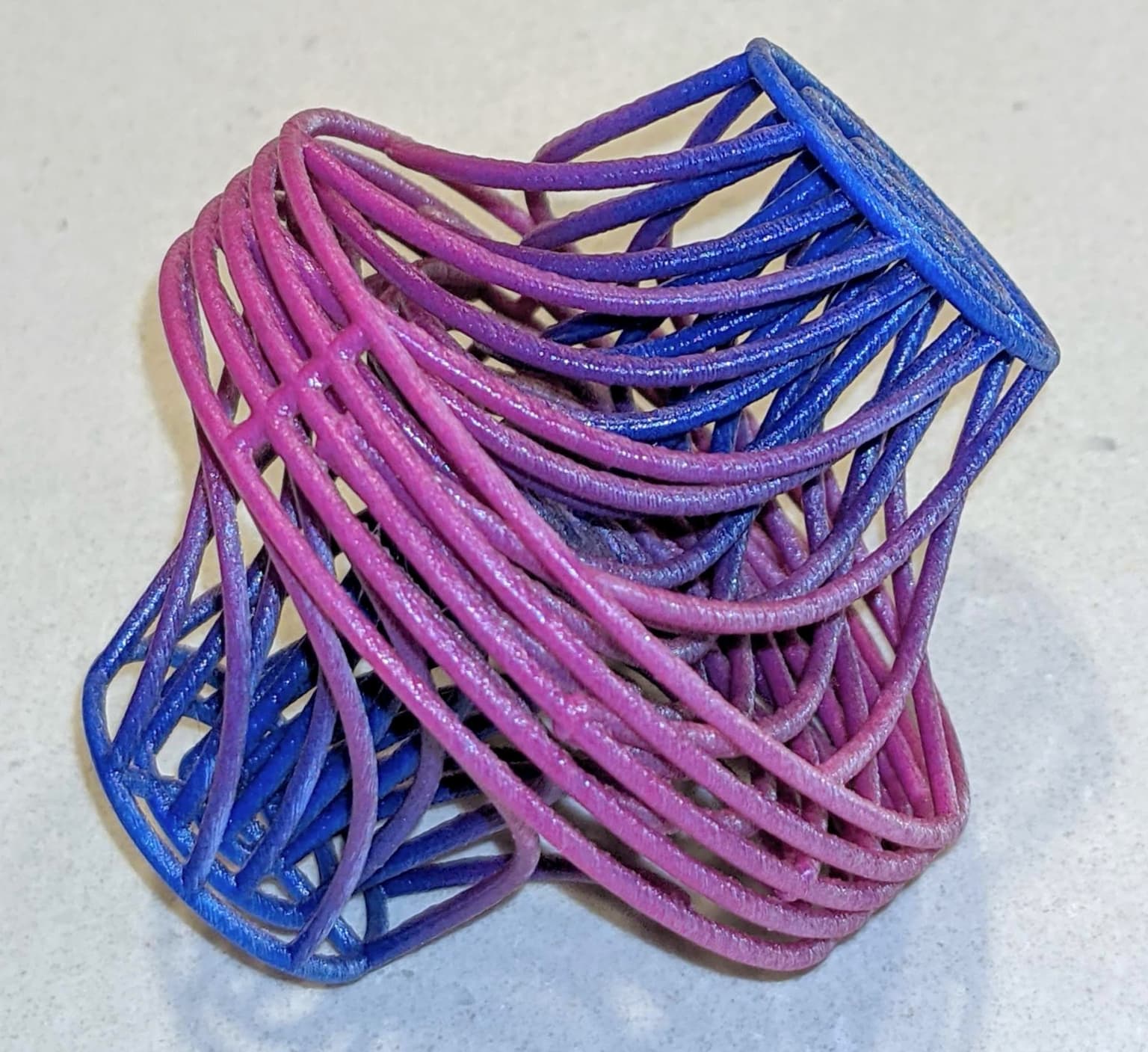
I use computational software to design images and sculpture inspired by the inherent beauty of mathematics. I am inspired by mathematical concepts, research topics, and found math. When I create art I work to understand the underlying theory, implementing it through algorithms, and honing the aesthetics to appeal to and reach a greater population. In these works I explore the streamlines of vector fields. A vector field is an assignment of a direction to every point in space. A streamline is the path that a particle in the vector field would follow if acted upon by the associated directions. An example of a vector field is the direction the wind is blowing; a plastic bag that is released at some location would follow a streamline.

Artworks

This is a model of the streamlines in the three-dimensional vector field $\langle 1/x^2,1/y^2,1/z^2\rangle$. The streamlines approaching either side of the coordinate planes have been connected to form continuous paths from points near $\langle 1,1,1\rangle$ to points near $\langle -1,-1,-1\rangle$. The dichotomous colors show that equally spaced streamlines at one end arrive in bunches near the other end.

This is a model of the streamlines in the three-dimensional vector field $\langle y,z,x\rangle$. Visible are the axis in the direction $\langle 1,1,1\rangle$ and spirals in the plane $x+y+z=0$. Outside these regions, the streamlines exhibit spiraling behavior around the axis. The magenta and blue circles you see on the side and top of this model were added for stability during the 3D printing process and are not themselves streamlines.