2022 Joint Mathematics Meetings

Francisco Lara-Dammer

Artists

Francisco Lara-Dammer

Professor of Mathematics

Mathematics Department, Escuela Politécnica Nacional

Quito-Ecuador

jose.larad@epn.edu.ec

Statement

One of my passions is centered around mathematical imagery and visualization because they bring clarity, simplicity, and elegance to understand concepts and ideas that at first sight look too abstract. However, when the images come with artistic touch, they give stronger understanding and a sense of awe as mathematics itself is art. The Theory of Groups is one of those fields in mathematics that in the past appeared to be at the extreme opposite of imagery. However, mathematicians such as Klein and Cayley have showed us the opposite. A group can indeed be drawn and result in spectacular gems.

Artworks

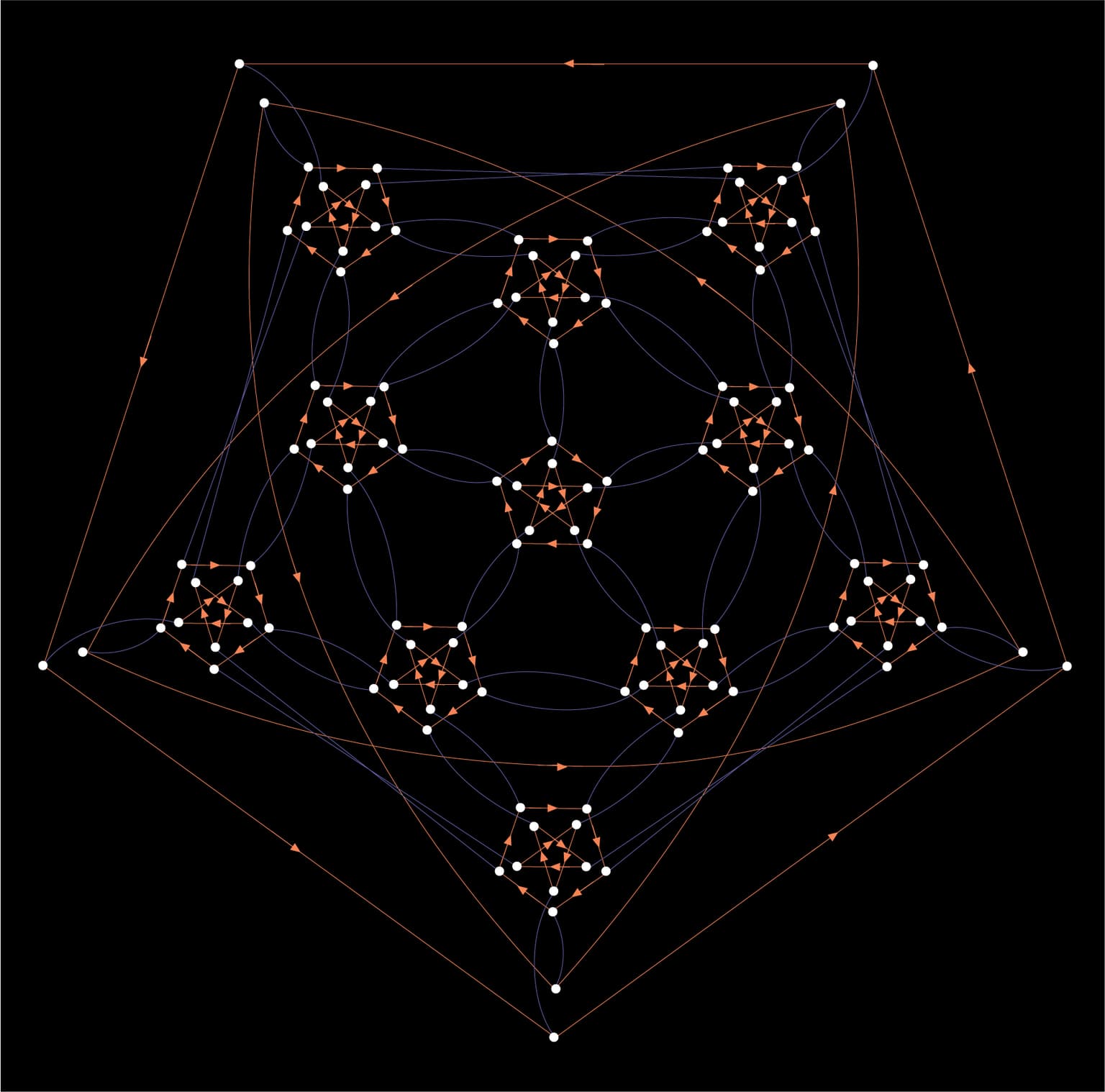
Image for entry 'S5 with pentagons'

S5 with pentagons

20 x 20 cm

The Geometer's Sketchpad, Illustrator, paper

2021

This is a Cayley diagram (named after the British mathematician Arthur Cayley, famous for his contributions in Algebra) of the symmetric group S5. This diagram is generated by two elements: one of period two and another one of period five. The period-5 generator creates the main theme of the diagram (pentagons of various sizes and shapes), whereas the period-2 generator links the pentagons. This diagram was inspired by the need to visualize its subgroup A5 (the alternating group of five elements) as a part of S5. The group S5 can be seen as made up of two cosets with respect to A5. One coset (A5 itself) can be interpreted by the dots in the convex pentagons, whereas the other coset can be interpreted as the dots in the starred pentagons.