2022 Joint Mathematics Meetings
Henry Segerman
Artists
Henry Segerman
Associate Professor of Mathematics
Oklahoma State University
Stillwater, Oklahoma, USA
Statement
Henry Segerman is an associate professor in the department of mathematics at Oklahoma State University. His research interests are in three-dimensional geometry and topology, and in mathematical art and visualization. In visualization he works in 3D printing, spherical video, virtual, and augmented reality. He is the author of the book "Visualizing Mathematics with 3D Printing".
Artworks
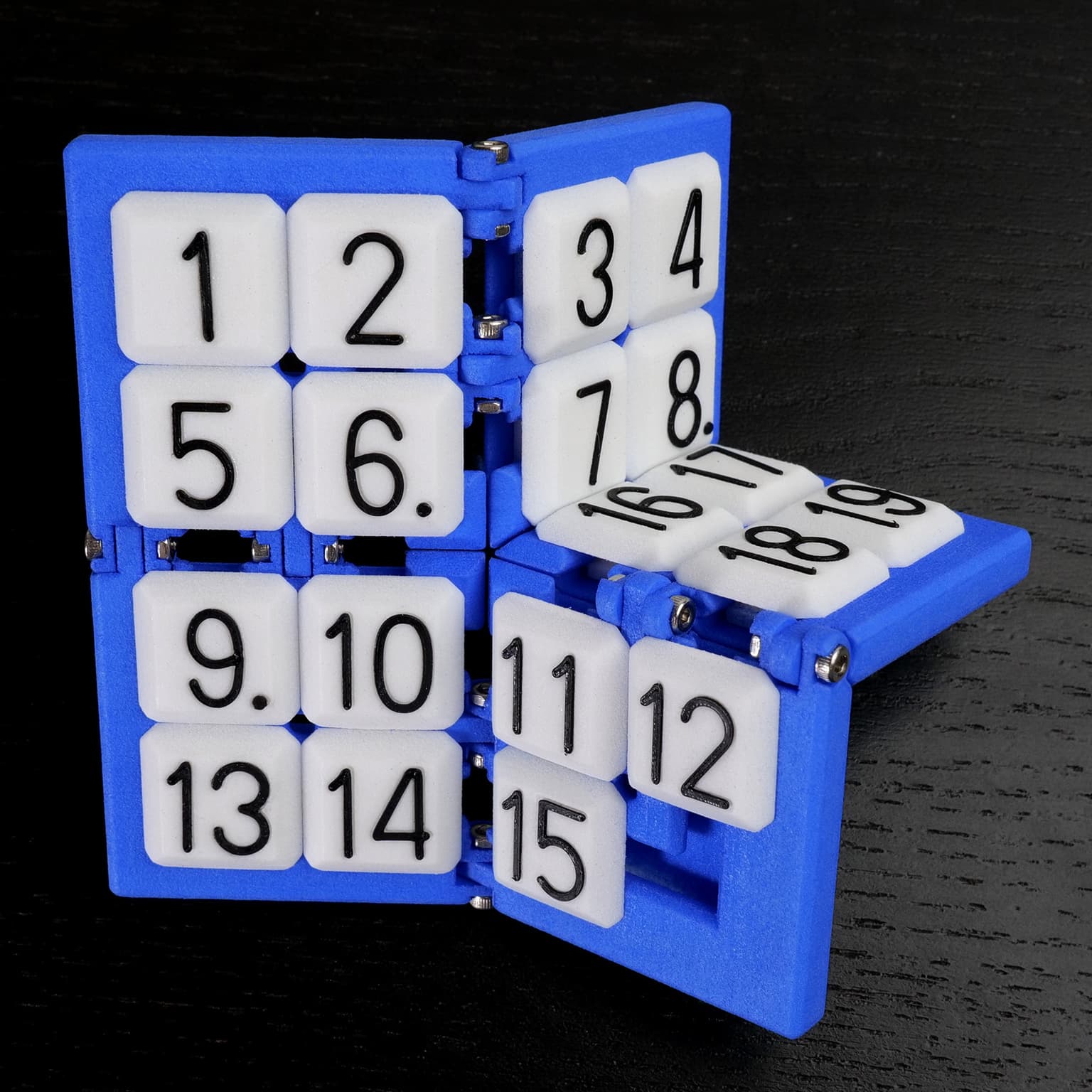
15 + 4 puzzle
5 x 9 x 9 cm
3D printed plastic, dye, nuts and bolts
2021
This variant of the classic $15$ puzzle differs in the addition of four extra tiles, jammed into the puzzle by replacing the usual $4\times 4$ frame with five $2\times 2$ squares, hinged around a central vertex. A tile can slide across the hinge between two squares when there is no angle between them. The puzzle has a cone point with angle $5\pi/2$ in the center. A consequence of this point of negative curvature is that the puzzle has non-trivial holonomy: a tile that travels around the central point comes back rotated by a quarter turn. Thus the orientation of the tiles is important.

Hyperbolic 29 puzzle
3 x 10 x 10 cm
3D printed plastic, dye, nuts and bolts
2021
In this sequel to the 15 + 4 puzzle, every vertex has five squares around it. This makes the puzzle considerably harder to solve. Even sliding a tile from one square of the frame to a neighbour can be difficult: the whole frame must be manipulated to get the two squares to be flat, possibly requiring many preparatory moves. There are no neat rows to put the numbers in for the solved state: instead the numbers spiral out from the center, each pointing to the number following it.