2022 Joint Mathematics Meetings
Robert Spann
Artists
Statement
The behavior of dynamic systems -- be they mathematical, physical, or financial—fascinates me. Where do they end up and how do they get there? Do they reach an equilibrium or do they continue moving randomly forever? Computer graphics allows one to see both the numerical and aesthetic properties of these systems. For some time, I have been interested in rational polynomials in the complex plane. I have been exploring their mathematical properties and using those properties to design images.
Artworks
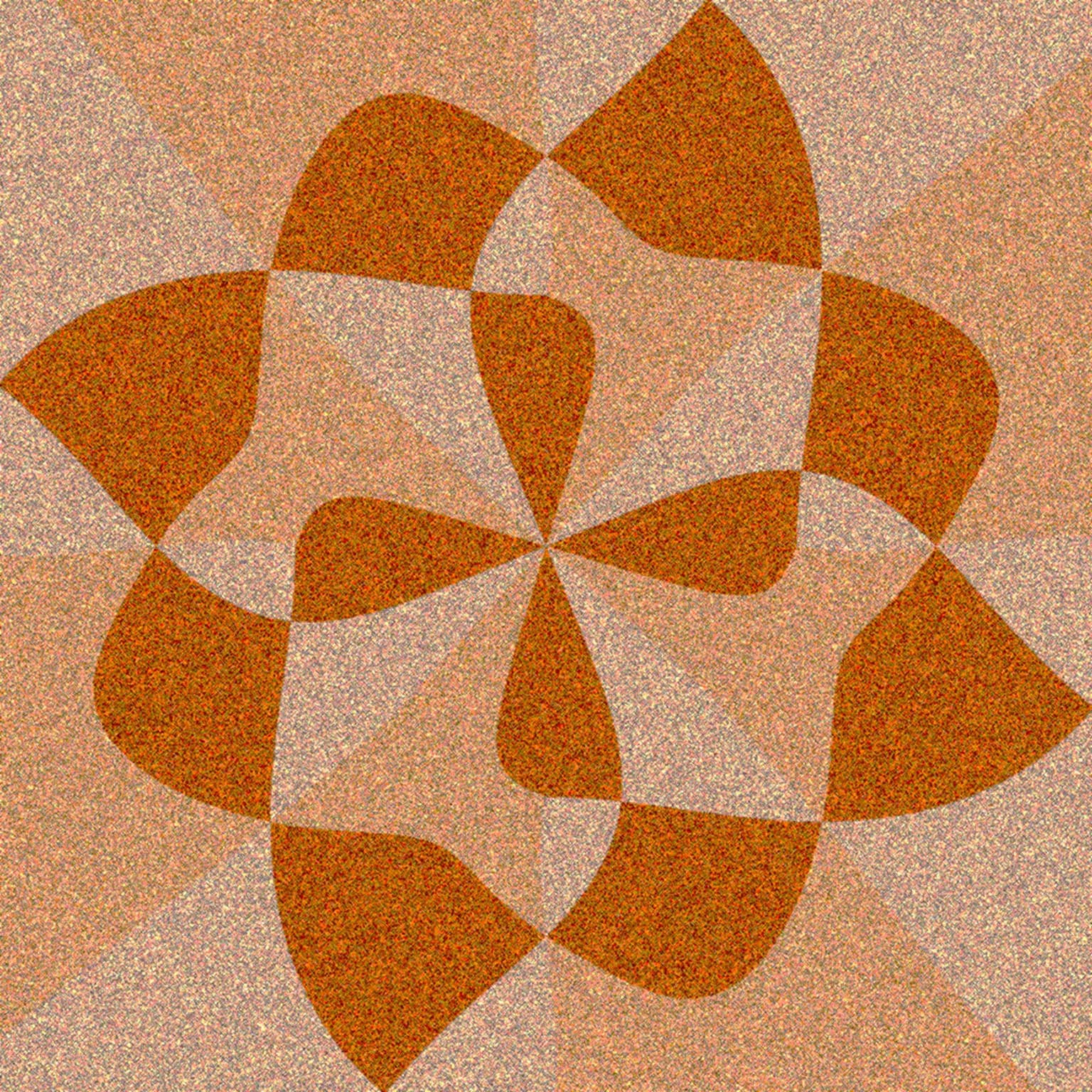
Sand Dollar
50 x 40 cm
Digital Print
2021
Sand Dollar is also produced by iterating the function $f(z)=z^2/w(1-z^4)$ twice with $w=-.5i$. Similarly, I compute $arg(z_{f})$ and use these values to color the image. The differences between the two images result from using three colors in Sand Dollar and nine colors in Chariot Wheel. In addition, I apply a pixelation filter from Photoshop to Sand Dollar to create the grain effect in that image.
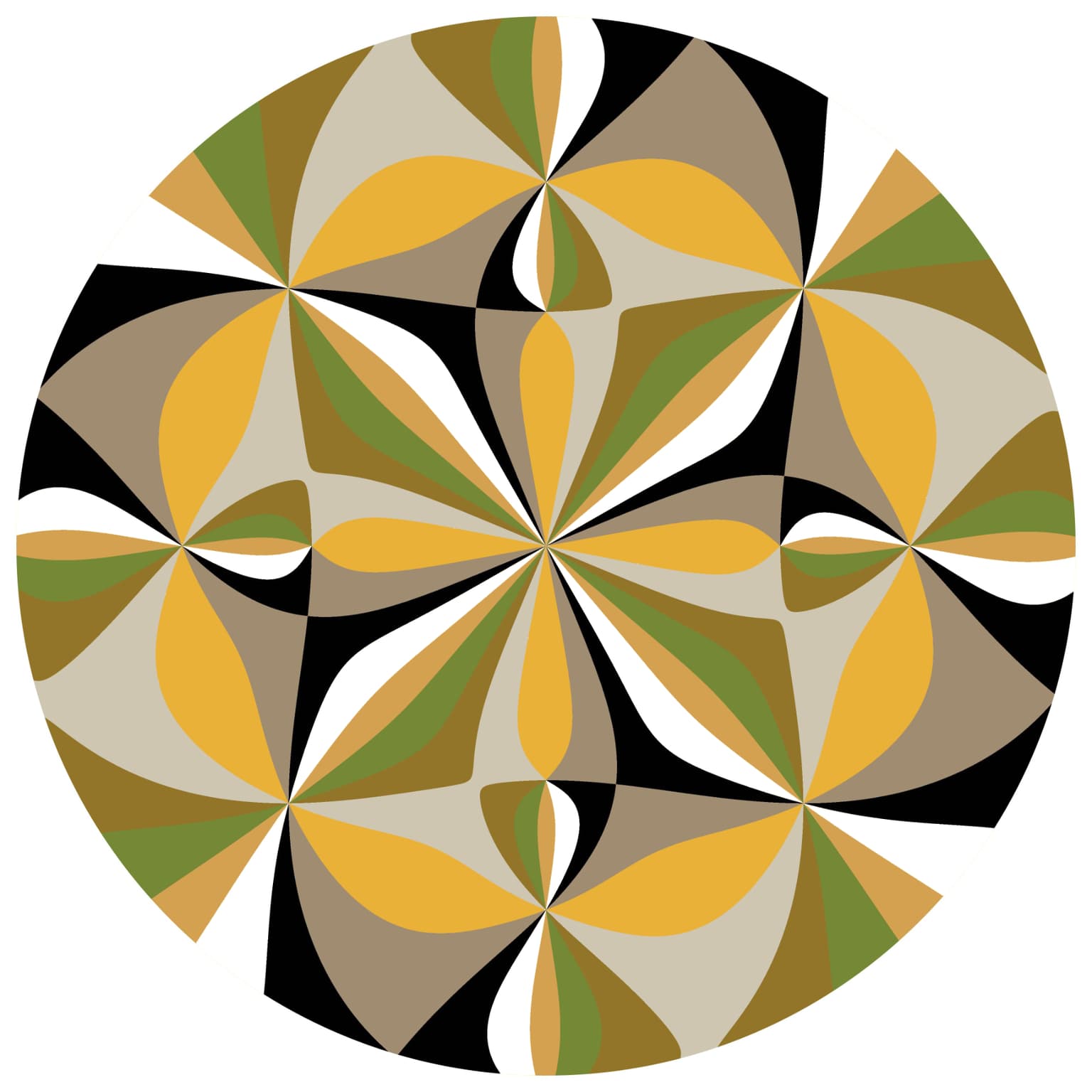
Chariot Wheel
50 x 40 cm
Digital print
2020
Chariot Wheel is produced by iterating the function $f(z)=z^2/w(1-z^4)$ twice with $w=-.5i$. This function has no attracting fixed or periodic points other than a super attracting fixed point at the origin. It does have the property that $f(z) = f(-z)$. As such, it can be used to produce images that are symmetric with respect to a 180 degree rotation. Letting $z_{f}$ be the value of $f(z)$ after two iterations, I compute $arg(z_{f})$ and use these values to color the image. I use commercial software (Circle Crop) to crop the image from a square to a circle.