Rashmi Sunder-Raj
I seek patterns to make sense of my world. Some of these I choose to
interpret as visual images. Sometimes this involves tessellations,
sometimes crochet...and sometimes other things.
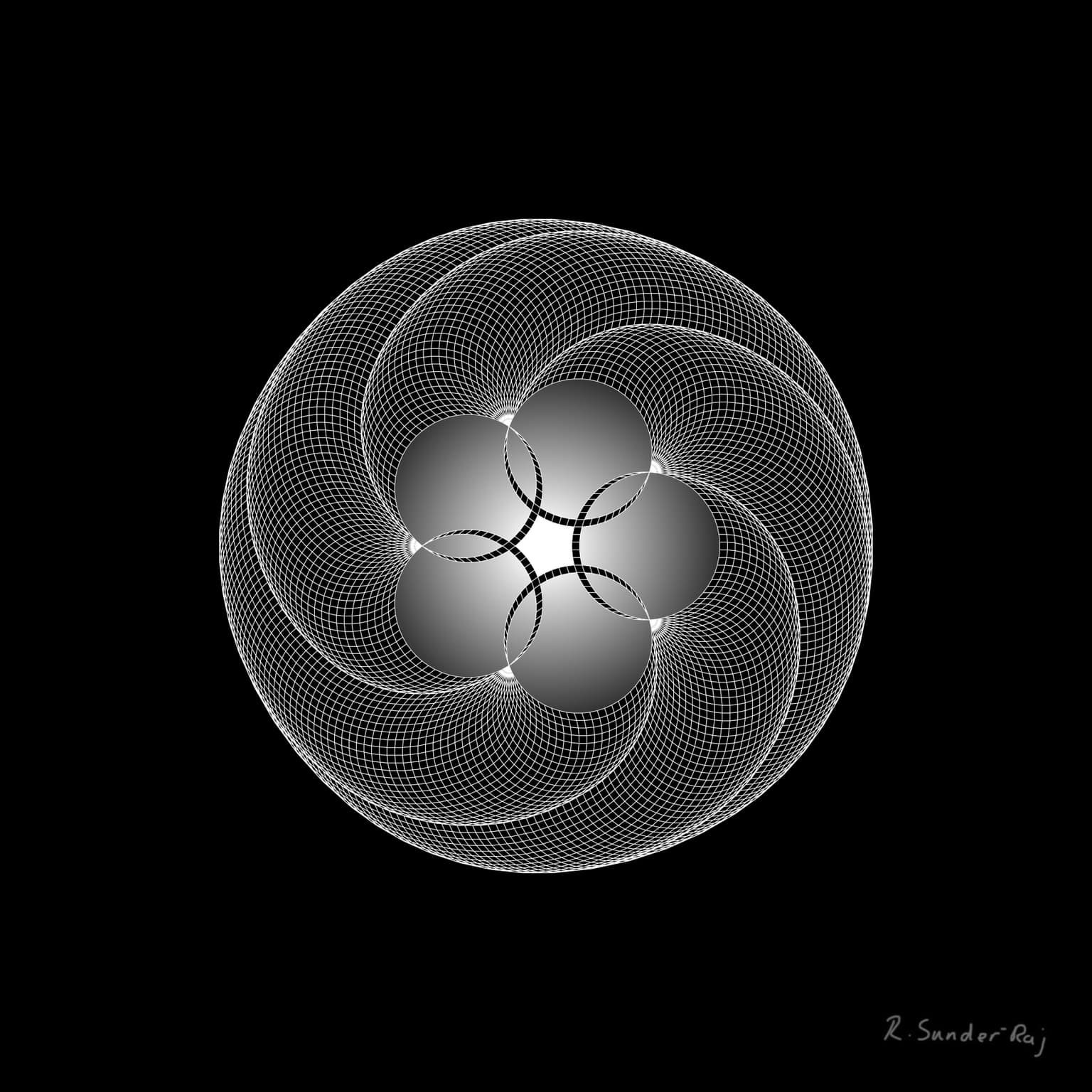
This piece came about from an investigation of rhombus patterns
which can be created from portions of regular n-fold rhombus
rosettes. In particular this 5-fold pattern is constructed using
the shapes which appear in a 60-fold rosette. The central pattern
is made of what I would call 5 intersecting curved “2π/60-rhombus
worms”. Given an angle θ (here I am only exploring those angles
which divide nicely into 2π), a θ-rhombus worm consists of a chain
of rhombi with angles (θ,π-θ), (2θ, π-2θ), (3θ,π-3θ),...,ending in
either (π-θ,θ) or (π-θ/2,θ/2) depending on whether or not θ
divides π. The rhombi are hinged so that all lie on one side of a
very bendy spine. If we let n=2π/θ, the curved form can be rotated
to form an n-fold rhombus rosette.
This is a pattern of edge-touching regular dodecagons arranged to
suggest irregular pentagonal tiles forming a Cairo Tiling. I have
been exploring how various regular polygons can give rise to the
formation of irregular pentagons with different angles. Here, each
“tile” approximates a 120°-90°-120°-90°-120° pentagon, giving the
dual of the snub square tiling (3.3.4.3.4). The arrangement and
colouring of the dodecagons hint at the location of the vertices
of the triangles and squares in 3.3.4.3.4. I hope to eventually
convert this pattern to physical form using either a type of bead
crochet, or linked jump rings.