2023 Bridges Conference Art Exhibition
Cruz Godar
Artists
Cruz Godar
Graduate Student
Mathematics Department, University of Oregon
Eugene, Oregon, USA
Statement
My first two college courses were in the departments of math and computer science, and since then, I’ve been fascinated by where the two subjects meet. Over the past few years, I’ve written dozens of web applets for creating and exploring fractals and similar objects, and eventually I developed a JavaScript library to streamline the process for myself and others. The kind of generative art these applets produce is a great way to counter the stereotypical presentation of math as a completely obscure subject, and my hope is for them to be both useful to mathematicians and appreciable by anyone, mathematician or not.
Artworks
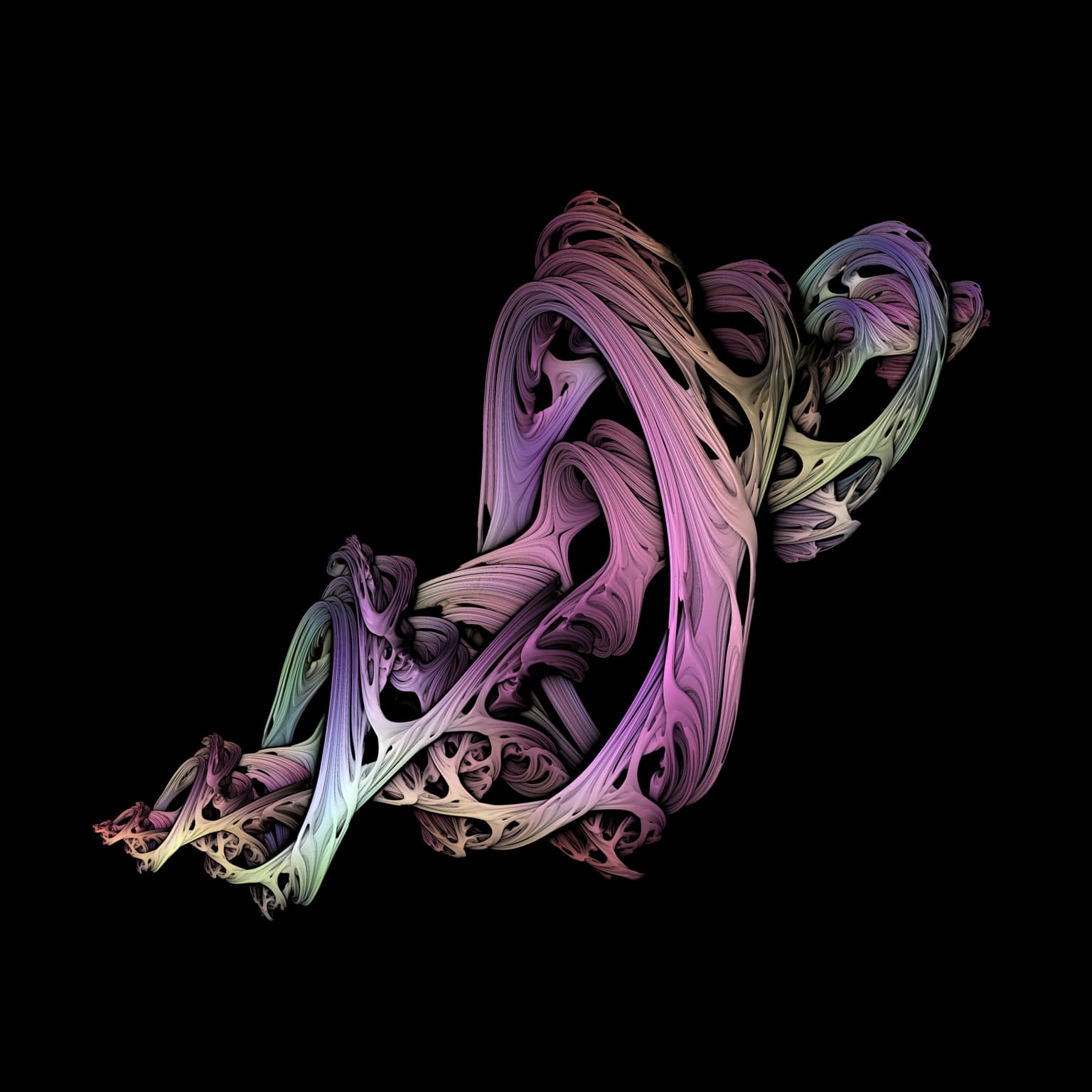
Julia sets are among the most famous fractals ever discovered. They are defined over the complex numbers, or ℂ, which is a 2D number system, but since there is no analogous 3D system, creating three-dimensional Julia sets is not so simple. But there is a 4D number system — the quaternions, written ℍ — that is similar to ℂ in all the right ways. While ℂ has a single value i with i² = -1, ℍ has i, j, and k, all of which square to -1. We may not be able to render all four dimensions of a quaternionic Julia set at once, but we can take a 3D slice, just like a 2D cross-section of a 3D object. The beautiful objects that result have all the intricacy and texture we would hope for, and the resemblance to 2D Julia sets couldn’t be clearer.