2023 Bridges Conference Art Exhibition
Laird Robert Hocking
Artists
Laird Robert Hocking
Mathematician and Artist
Taiwan
Statement
As a kid, I grew up exploring coastal British Columbia, sometimes bringing home treasure that I found. I would sometimes feel an intense need to find out what the view is like from a mountain visible in the distance. Similarly, as an adult I find myself intensely curious about, for example, "What do 3D cross-sections of 4D Menger sponges look like?". In both cases, an answer exists - I just have to go out and find it (either by climbing the mountain, or by doing the math). In this spirit, I see mathematical art less as a creative process and more one of exploration and discovery. Just like when I was a kid, I rarely find anything interesting. However, I occasionally stumble up a treasure which I want to take home (with a 3D printer).
Artworks
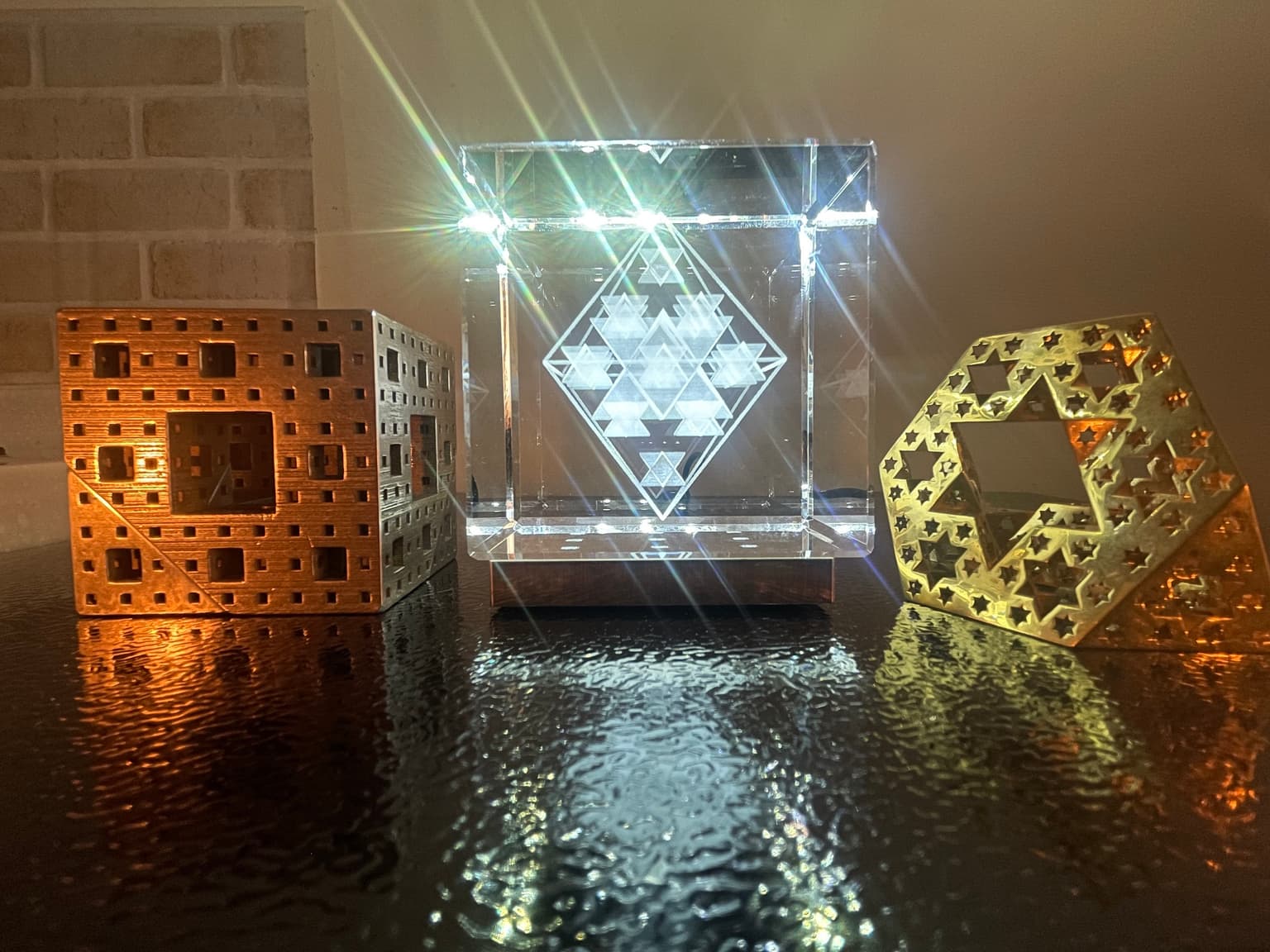
Menger sponges and their sections
10.0 x 25.0 x 25.0 cm
Steel, brass, glass, gold
2023