2023 Bridges Conference Art Exhibition
Paul Zorn, Barry Cipra
Artists
Statement
We are interested in exploring the sometimes surprising aesthetic effects that arise from simple mathematical rules. The tricoloring rule for knots is one such simple rule: At each overpass, the three strands of a knot (or link) are either all the same color or three different colors. By applying this rule to a square weave of an infinite “carpet" with prespecified colors along the fringe on two adjacent sides, we’ve found some fascinating and unexpected patterns.
Artworks
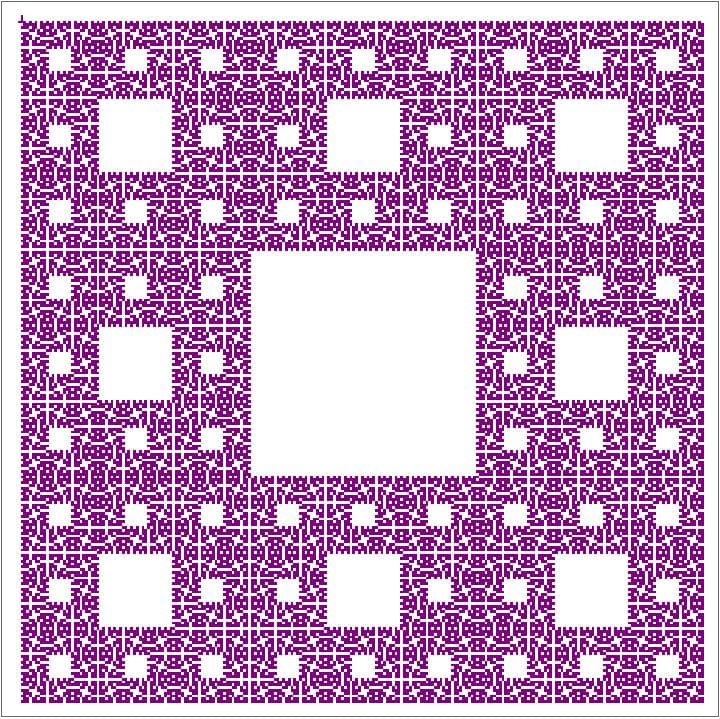
Try a Different Color
45.0 x 45.0 cm
digital print
2023
Starting from an all White “carpet,” changing the color of the first “warp” and “weft” threads then applying the tricoloring rule from knot theory to the rest of the weave, we find an unexpected fractal pattern in the way that some squares stay White while others change color. Here we show a 243x243 portion of a potentially infinite carpet, with Purple indicating squares that change to one of the other two colors. The pattern is strongly reminiscent of the famous "Sierpinski carpet.” Mathematical questions abound, including many we are still trying to answer, such as What can be said precisely about connections to the Sierpinski carpet, and How are symmetry properties of the fringes reflected in the carpet’s interior?