2023 Joint Mathematics Meetings
Aiman Soliman
Artists
Aiman Soliman
Research Scientist
NCSA - University of Illinois Urbana-Champaign
Champaign, Illinois, USA
Statement
My graphic art features tessellations, symmetry, and visual illusions. In my art, I explore approaches to the visual harmony of forms and colors rooted in mathematical concepts such as symmetry, tessellations, and group theory. My biggest inspiration came from the music of JS Bach, particularly those compositions written in the style of counterpoint. Bach's contrapuntal compositions depend heavily on mathematics. However, his music does not only evoke a sense of order but in addition a wide range of human emotions.
Artworks
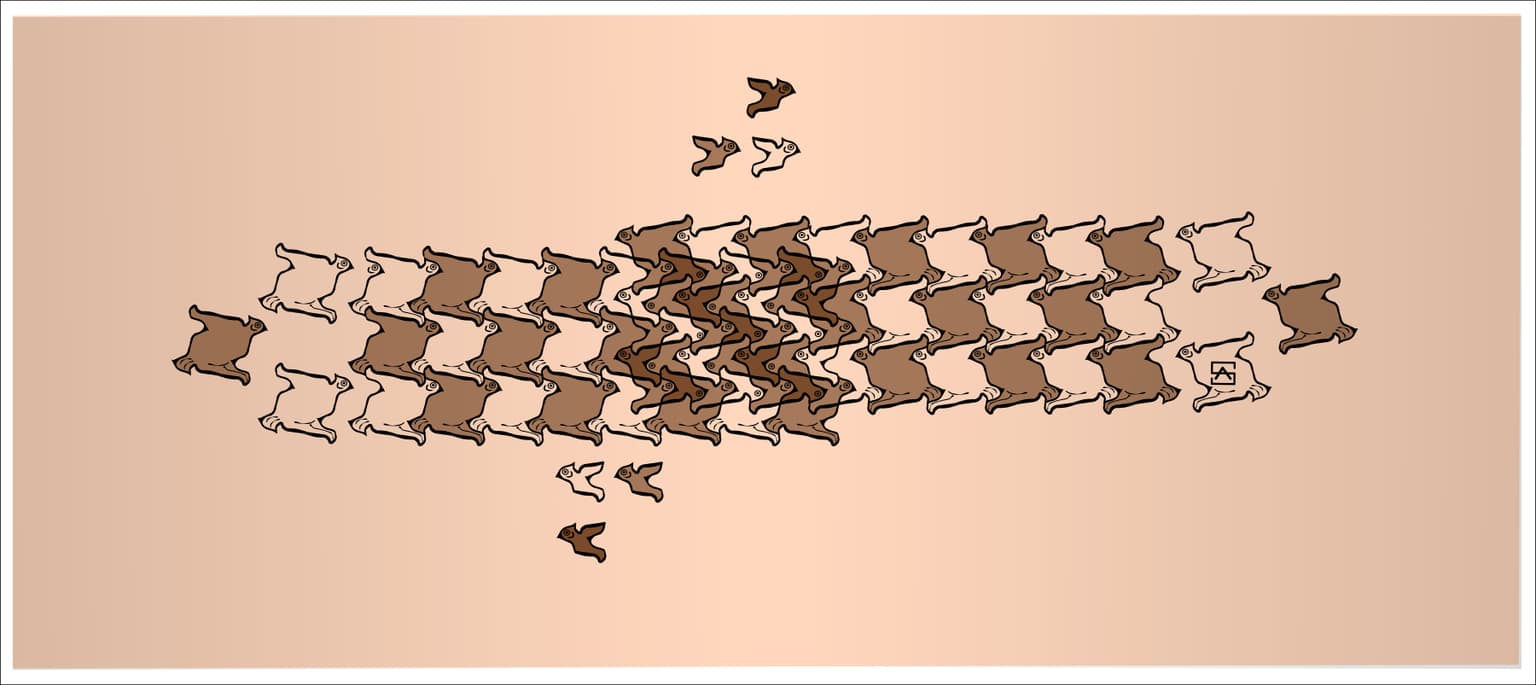
In this artwork, I tried to develop the visual equivalent of a musical composition with a two-voice counterpoint. Two flocks of tessellated quails fly into each other, and when they intersect, they reveal a new motif (songbirds) with a different plane symmetry. The quail tessellation is combined harmonically with a copy of itself, which is reflected and shifted half a unit in both x and y directions. Combining the two tessellations introduces glide reflection axes inside the original quail motifs and transforms the quail plane symmetry group from (p2) to the songbird symmetry group of (pgg). The shown transformation is in agreement with theoretical wallpaper subgroup relationships, which were studied by mathematicians, including Coxeter.