2023 Joint Mathematics Meetings

Robert Hearn

Artists

Robert Hearn

Self

Self

Portola Valley, California, USA

bob@hearn.to

Statement

In the process of investigating two-dimensional twisty puzzles, I stumbled onto a new kind of geometric symmetry, generalizing the traditional notion of symmetry group. A "compound symmetry group" is a group generated by multiple isometries whose domains overlap. A simple example is the group generated by $n$-fold rotations within one disk, and $m$-fold rotations within an overlapping disk. In developing this notion and investigating its properties, I have created software which lets me explore the space of compound symmetries, and create mathematical artwork based on it. This artwork can also be thought of as images from a new kind of kaleidoscope, which renders symmetries that are traditionally forbidden.

Artworks

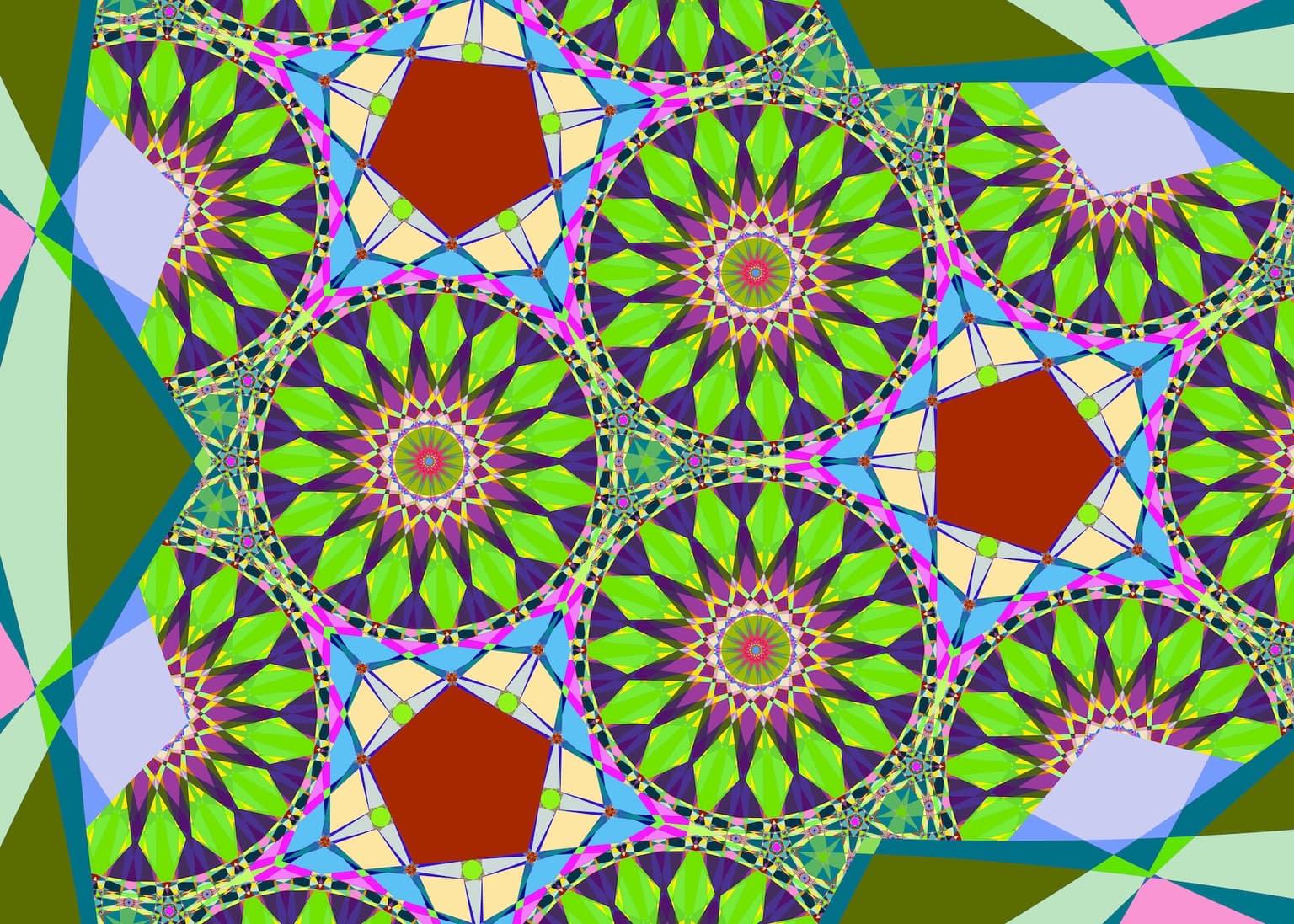
Image for entry 'Compound Symmetry Group #3'

Compound Symmetry Group #3

51 x 71 cm

Canvas

2022

This piece shows a portion of the image of a compound symmetry group combining three-fold and five-fold symmetries. Both symmetries are present here, as well as some multiples (e.g 15-fold symmetries). Necessarily, the local symmetries become broken at larger scales — there are no repeating patterns in the plane with these symmetries. The parameters here are chosen to be just short of the transition to a fractal, displaying a large but finite complexity. The colors are chosen to complement the structure, in this case suggesting a floral pattern. The individual regions may also be thought of as puzzle pieces in an immensely complex two-dimensional twisty puzzle. Pieces with the same colors may be permuted by this puzzle.