2023 Joint Mathematics Meetings
Bruce Torrence
Artists
Bruce Torrence
Professor Emeritus of Mathematics
Randolph-Macon College
Ashland, Virginia, USA
Statement
As someone with a passion for the visual arts, I've long been fascinated by the ways humans process and interpret visual information. As a mathematician, I relish the joy and satisfaction that come from solving a difficult problem and understanding its underlying ideas. I strive in my own work to present viewers with patterns and symmetry, to convey a sense of wonder in its intricacy and scale, and to challenge viewers to make sense of what they see.
Artworks
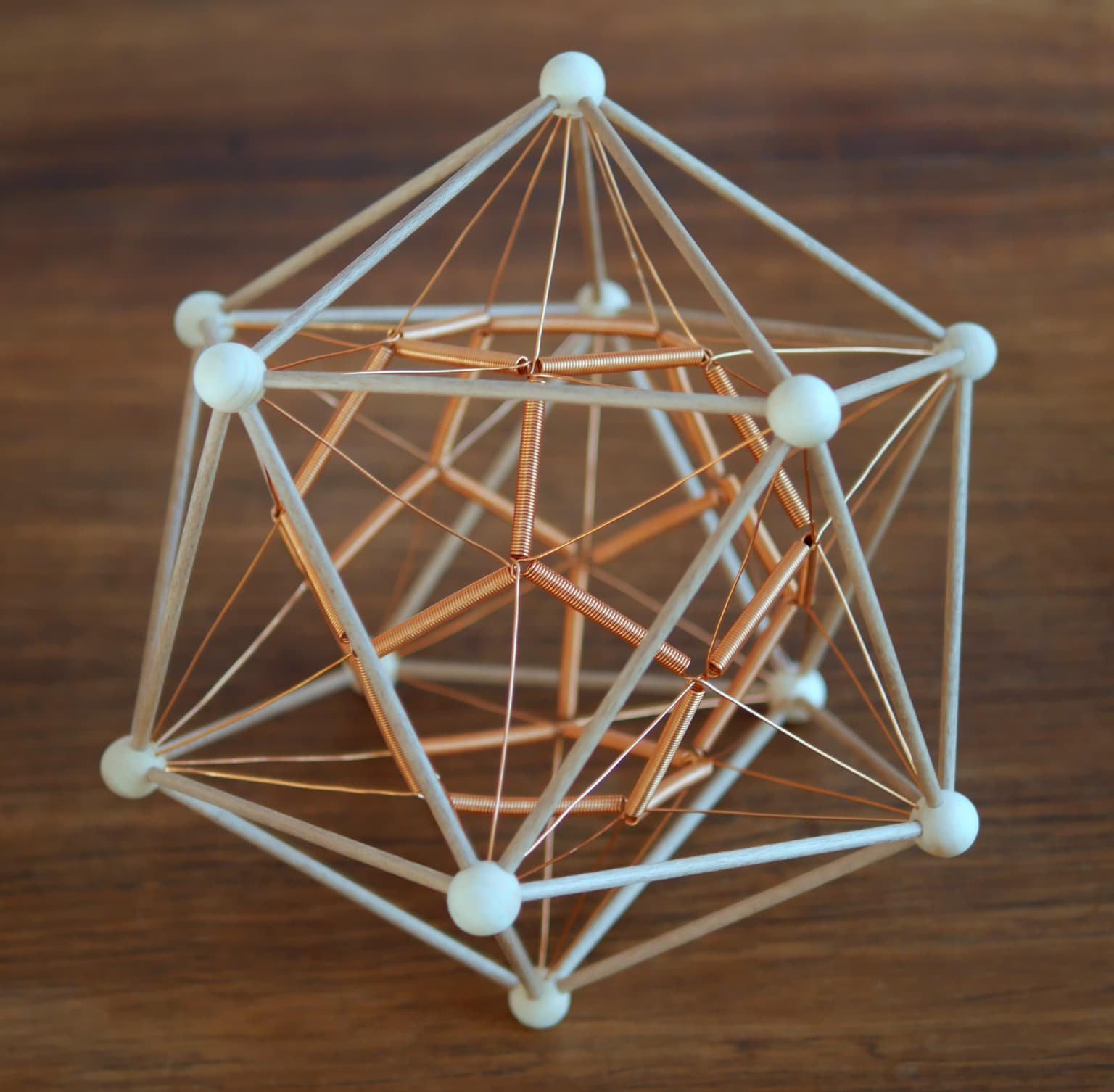
Highlighted Hexacross
25 x 25 x 25 cm
Wood and Aluminum Wire
2022
A wood edge model of the icosahedron is supplemented with internal wire edges which join all pairs of non-adjacent vertices except those that are antipodal. The center section of each wire edge is wound in a tight spiral to highlight it. These highlighted edge sections outline a suspended dodecahedron, and the full wire structure forms its first stellation. The entire work may be thought of as an edge model of the projection into 3-space of the 6-dimensional cross-polytope, where the six coordinate axes map to the six 5-fold symmetry axes of the Icosahedron. Each pair of antipodal vertices on the icosahedron represent the numbers 1 and −1 on a coordinate axis. Simplicity and purity of form drives the aesthetic. Natural wood balls were drilled using a custom 3D-printed drill jig.
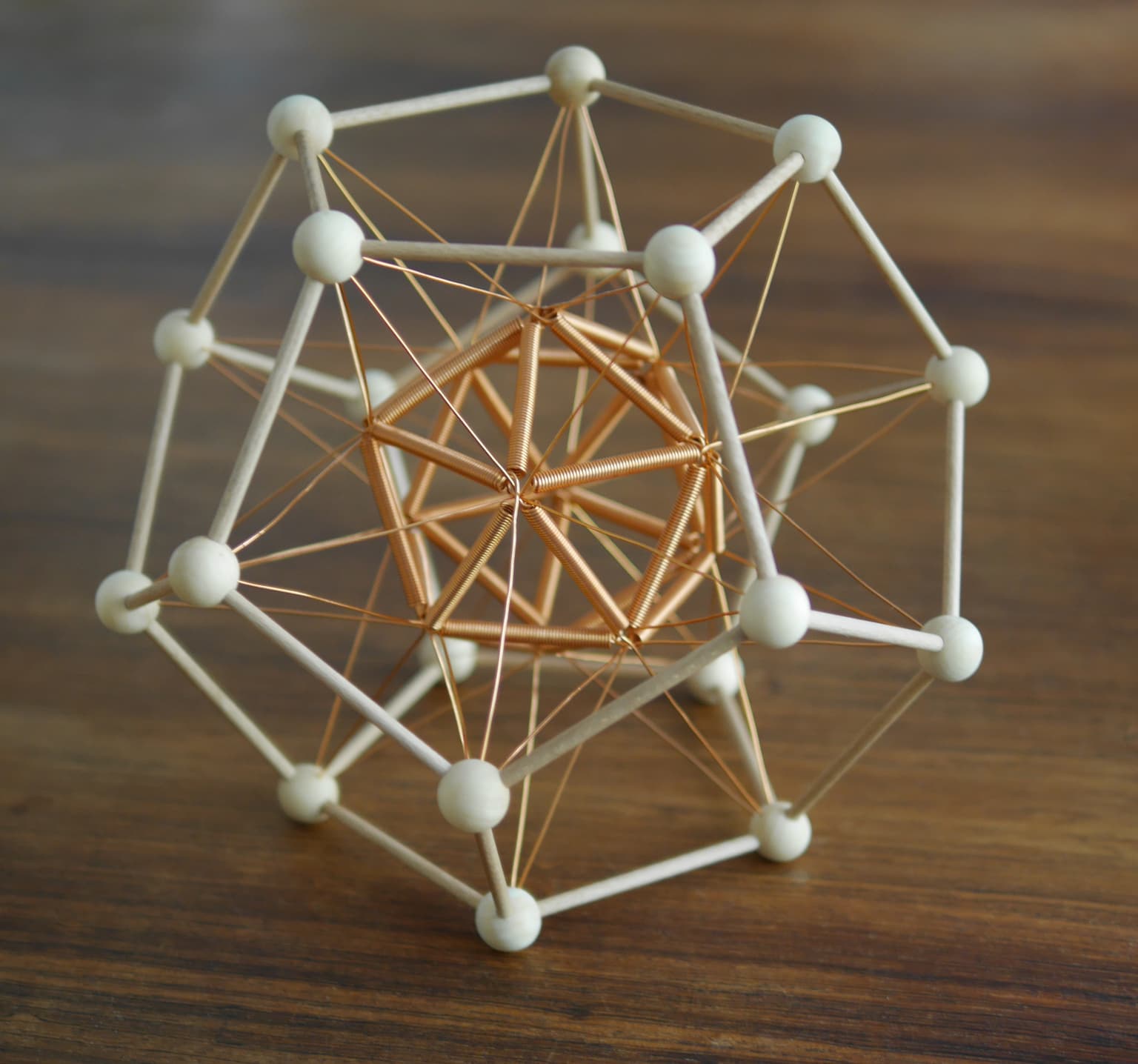
Crossing Space 10
23 x 23 x 23 cm
Wood and Aluminum Wire
2022
This complementary piece highlights an icosahedron suspended in a dodecahedron, with the complete wire structure outlining a great stellated dodecahedron. The wire edges are identical in both works: they have the same length, and an identical coiled middle section. This puts the lengths of the wood edges in the two works in the golden ratio. As an edge model, this piece may be interpreted as a proper subset of the 10-dimensional cross-polytope under the projection mapping the ten coordinate axes to the ten 3-fold symmetry axes of the dodecahedron. Here there are only three wood edges and three wire edges at each vertex, so each vertex in this model has only six of the 18 edges of the full 10-dimensional cross-polytope.