2023 Joint Mathematics Meetings
Eve Torrence
Artists
Eve Torrence
Professor Emeritus of Mathematics
Randolph-Macon College
Ashland, Virginia, USA
Statement
I enjoy using paper to create models to enhance my understanding of geometric objects. Through the process of creating a 3-dimensional form I am able to truly understand its structure. The mental challenge of designing a model and the physical challenge of building it are particularly satisfying when combined. These pieces were inspired by map models created by Susan Goldstine in ceramics, crochet and bead crochet and Moira Chas's crocheted yarn models.
Artworks
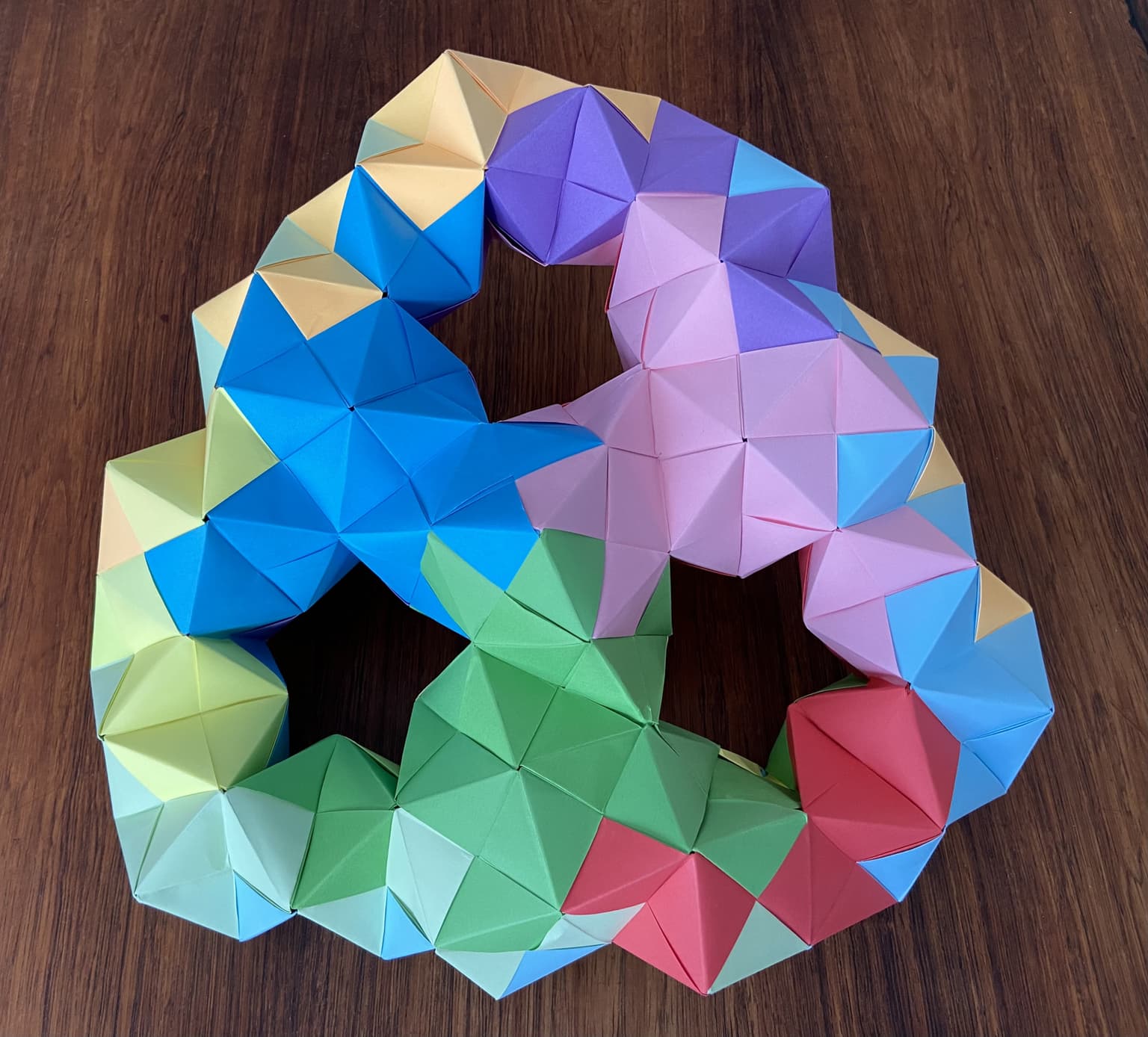
Cloud Nine
39 x 39 x 19 cm
paper
2022
In 1968 Ringel and Youngs showed that maps requiring $n = \lfloor{{7 + \sqrt{1 + 48 k} \over 2}}\rfloor$ colors exist for all $k$-holed tori. But their proof does not provide examples of maps we can see. Few physical examples of maps on higher genus surfaces exist. In 2022 Carlo Sequin designed a highly symmetric map on a 3-holed torus that requires $\lfloor{{7 + \sqrt{1 + 48 * 3} \over 2}}\rfloor = 9$ colors. The title comes from the cloud like structure and the thrill of being able to build this 3-dimensional model of Sequin’s 3-holed torus map out of 129 Sonobe units.
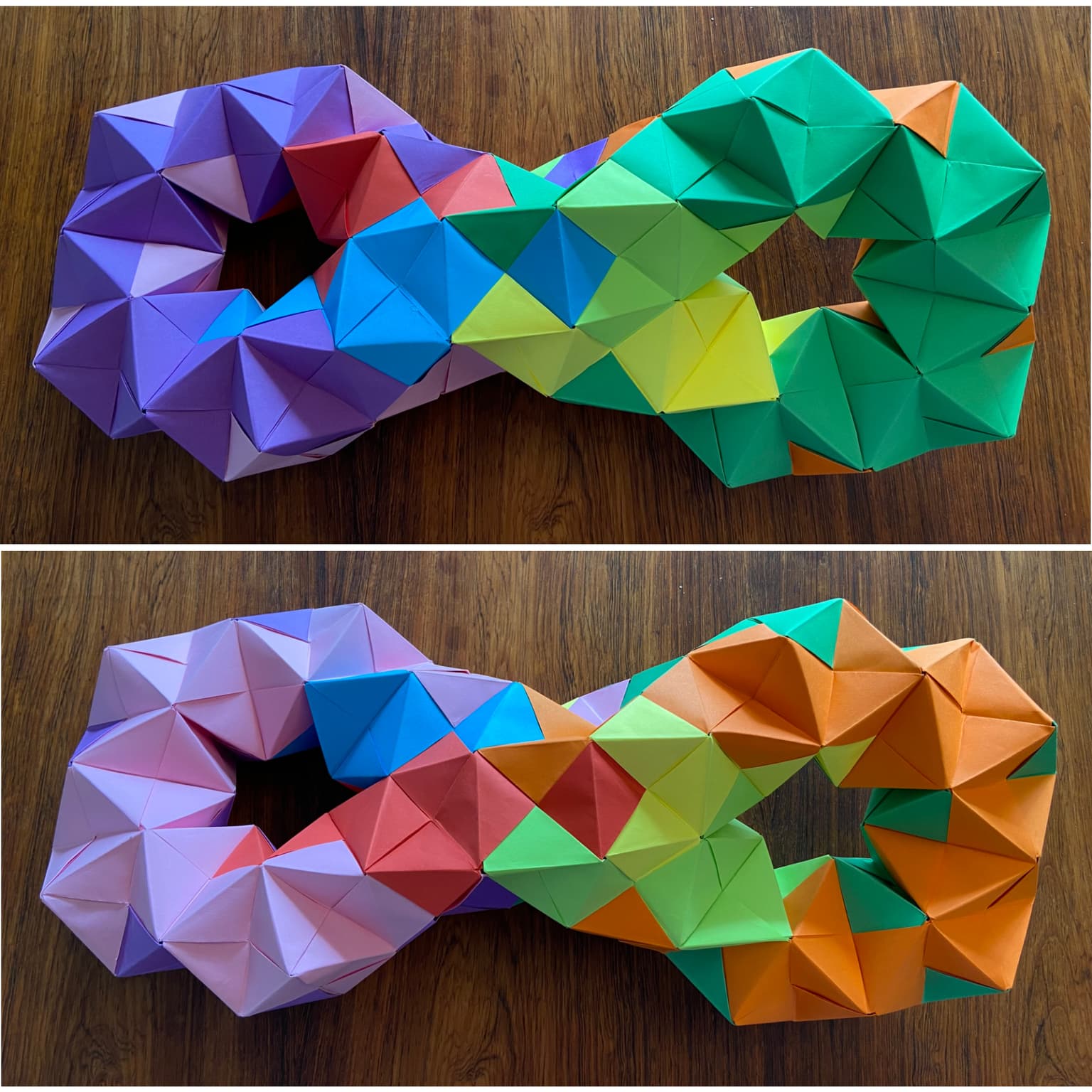
In 1890 Percy Heawood proved that any map on a k-holed torus can be properly colored with at most $n = \lfloor{{7 + \sqrt{1 + 48 k} \over 2}}\rfloor$ colors. Heawood published a map on a torus that required $\lfloor{{7 + \sqrt{1 + 48 * 1} \over 2}}\rfloor = 7$ colors but did not show that a map requiring $n$ colors exists on a k-holed torus for $k > 1$. This modular origami model shows a map on a 2-holed torus requiring $\lfloor{{7 + \sqrt{1 + 48 * 2} \over 2}}\rfloor = 8$ colors. The creases give the sculpture a frenzied look and the slight twist lends an off-balance feel to the structure. Directions for building this model, as well as two distinct seven-color torus maps, can be found in my 2022 Bridges workshop paper, in the link above.