2023 Joint Mathematics Meetings
Steve Trettel
Artists
Stephen J Trettel
Assistant Professor of Mathematics
University of San Francisco
San Francisco California, USA
Statement
When illustrating mathematics, I focus on illustrating low-dimensional geometric objects which embody both visual and mathematical beauty. My primary medium consists of 3-dimensional computer graphics, and I typically write a new program for each piece. I aim to capture each mathematical object in its natural environment, and provide a view of what it 'would really look like' were it possible to visit the abstract world that it calls home.
Artworks
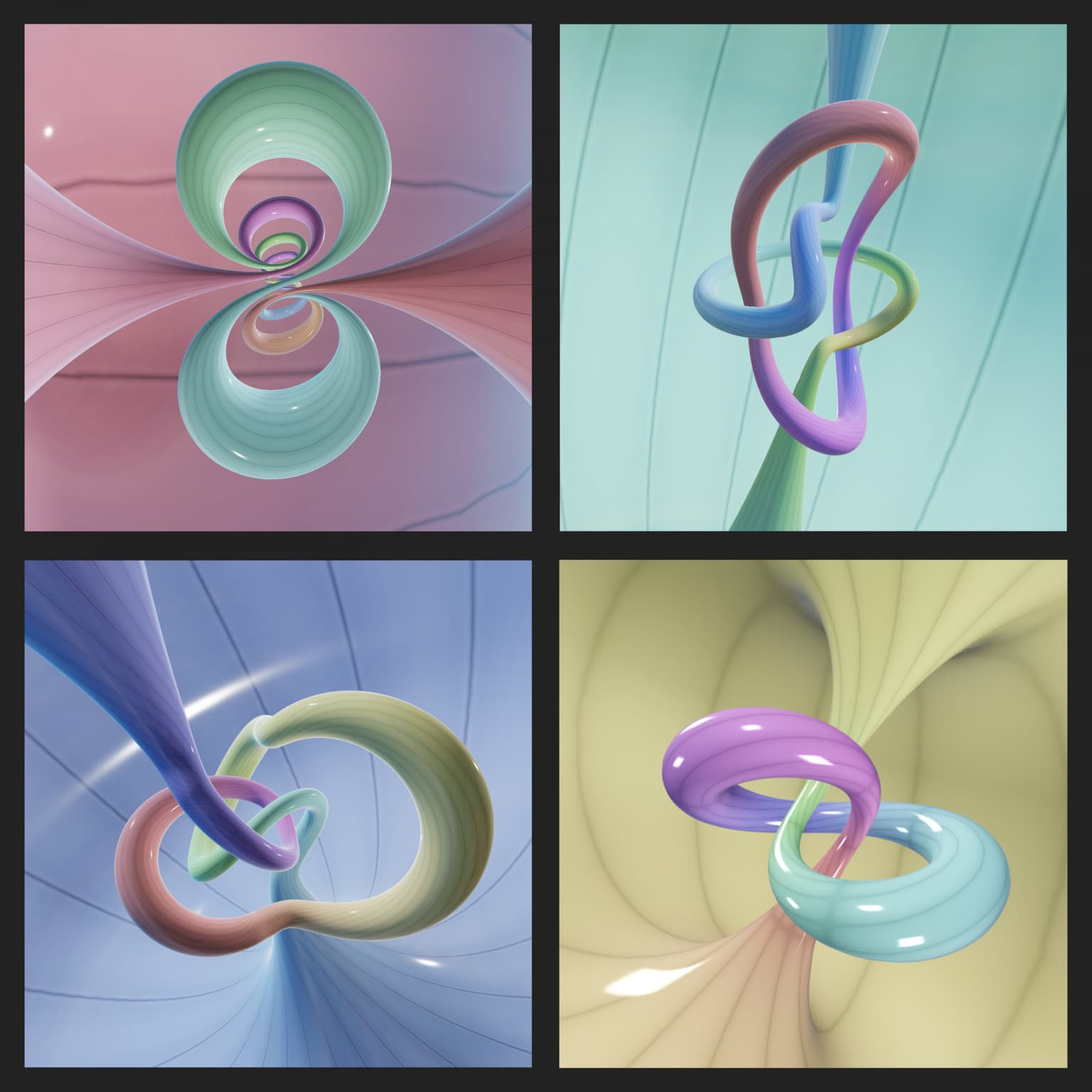
Knot Complements
50 x 50 x 1 cm
Javascript and GLSL (graphics shading language for the GPU).
2022
Knots are often studied via their complements: the space left behind upon removing a tubular neighborhood of the knot from the 3-dimensional sphere. This work provides an insider's view of four such complements: the (2,11) torus, knot, the whitehead link, the trefoil, and the figure-8.
The details: an embedding of each knot/link was computed, and used to parameterize the boundary of a regular $\varepsilon$-tube about the knot/link, with respect to the round metric on $\mathbb{S}^3$. The resulting surface was projected into $\mathbb{R}^3$ via stereographic projection from a point on the knot, ensuring the region visible in the images homeomorphic to the complement itself.