2024 Joint Mathematics Meetings
Benjamin David Grimmer
Artists
Benjamin David Grimmer
Assistant Professor of Applied Mathematics and Statistics
Applied Mathematics and Statistics, Johns Hopkins University
Baltimore, MD, USA
Statement
I am an optimizer. I do research in optimization, searching over every point in a shape to find the best one (by some definition and rigor). Several mathematical insights just can't be shared with chalk in 2D in teaching or research talks. This piece shows a surprising connection between different geometries: Inside the 4-norm ball in 3D lives the 2-norm ball in 2D! (This case is just the start of a delightful hierarchy of relationships between norms across dimensions.)
Artworks
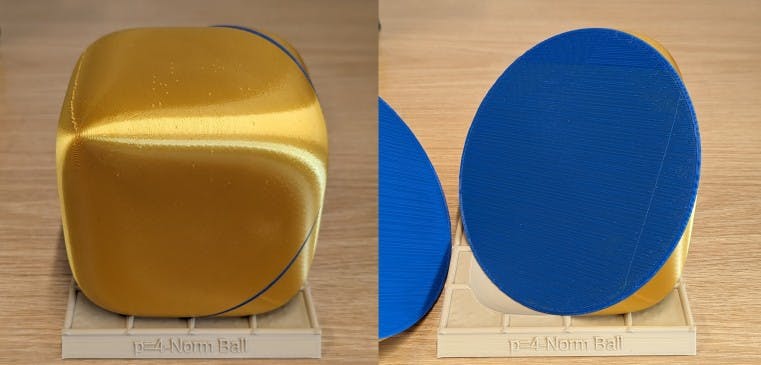
P-norms provide a range of different ways to measure distance. The 2-norm is our classic Euclidean idea. The 1-norm is often called the taxicab or Manhattan distance since it captures how we move within a fixed grid. There are infinitely more to explore for any p>=1.
This work shows a surprising connection between these different notions of distance that is only clear as we look up into higher dimensions. Inside the 4-norm ball (along a certain subspace) is the 2-norm ball. Hence, after some changes of variables (and lifting to a higher dimension), everything classic and Euclidean is a special case of 4-norms! This can be done more generally with even values of p by going beyond dimension 3!!