2024 Joint Mathematics Meetings
Carlo H. Séquin
Artists
Carlo H. Séquin
Prof. Emeritus
EECS Department, University of California, Berkeley
Berkeley, California, USA
Statement
Mathematical knots are closed-loop curves embedded in Euclidean 3-space. I am turning such knots into geometrical sculpture models by sweeping a suitable cross-sectional profile along that curve; possibly letting the profile grow and shrink and twist as it moves along the knotted sweep curve. A quick way to increase the complexity of the knot is to follow the knot-curve with a “cable” comprised of multiple parallel strands. This set of cable strands can be connected into a single mathematical knot, if the cable is given some overall twist, so that each strand connects with one of the other strands in a cyclic manner. Knot complexity can be further enhanced by weaving the strands into a braid.
Artworks
"Braided Trefoil"
6.0 x 15.0 x 15.0 cm
PLA, 3D Print
2023
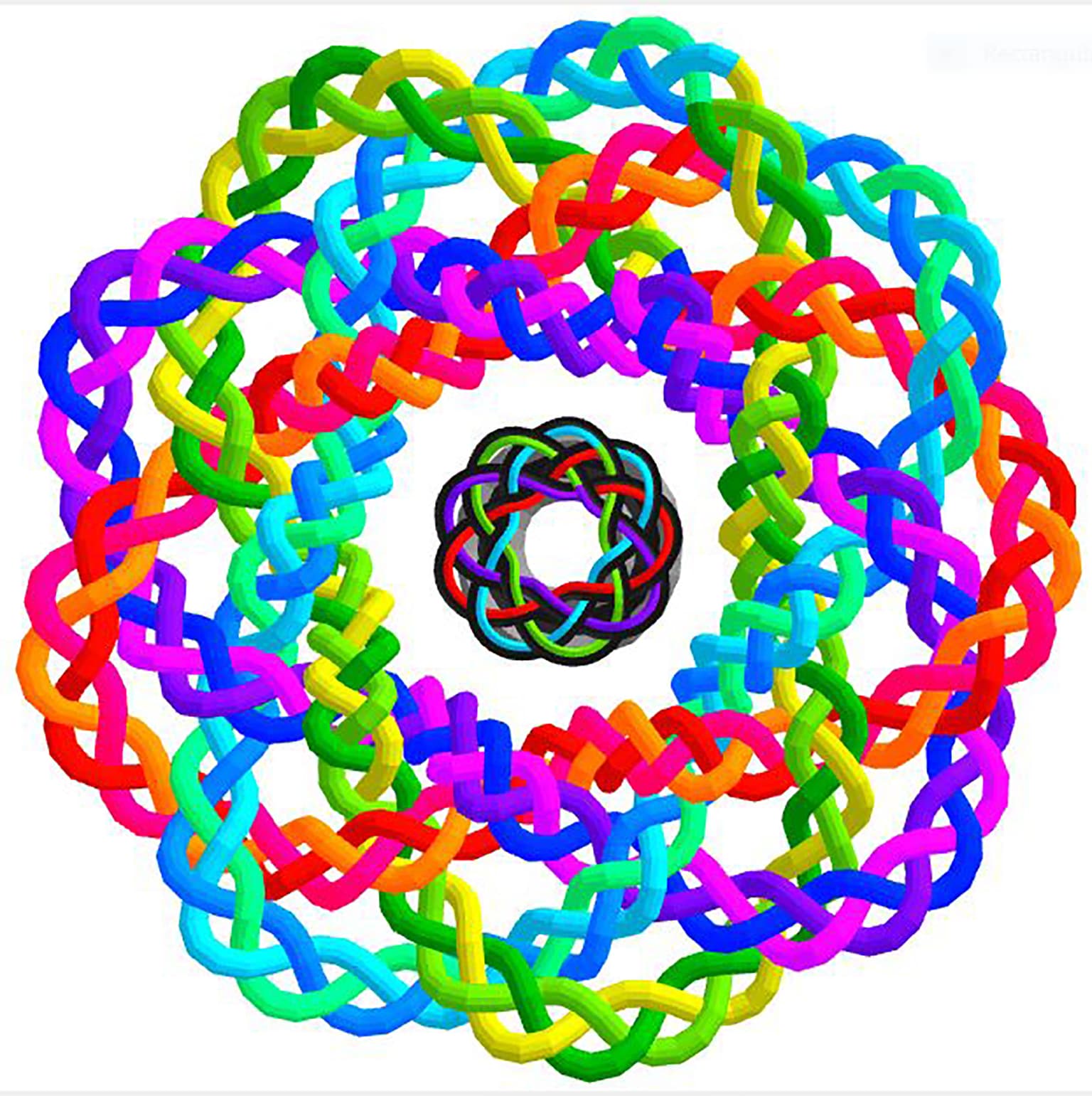
“Braided Braid”
24.0 x 24.0 x 0.0 cm
Computer Graphics
2023