2024 Joint Mathematics Meetings
Elliot Kienzle
Artists
Elliot Kienzle
Mathematics graduate student
Mathematics department, University of California, Berkeley
Berkeley, USA
Statement
I believe in the pedagogical power of a pretty picture. It can get people interested, keep them engaged, and sometimes make the uncomprehended click. I use art to aid and explain math. Even when direct representation is impossible, art can convey how mathematical ideas fit together and some aspects of their flavor. I hope this can aid students and make high-level math more accessible. It takes years of dedicated study to appreciate the mathematics I want to convey, but anyone with eyes can appreciate the art. I try to take the beauty we mathematicians see in symbols and put it on the page for the world to see.
Artworks
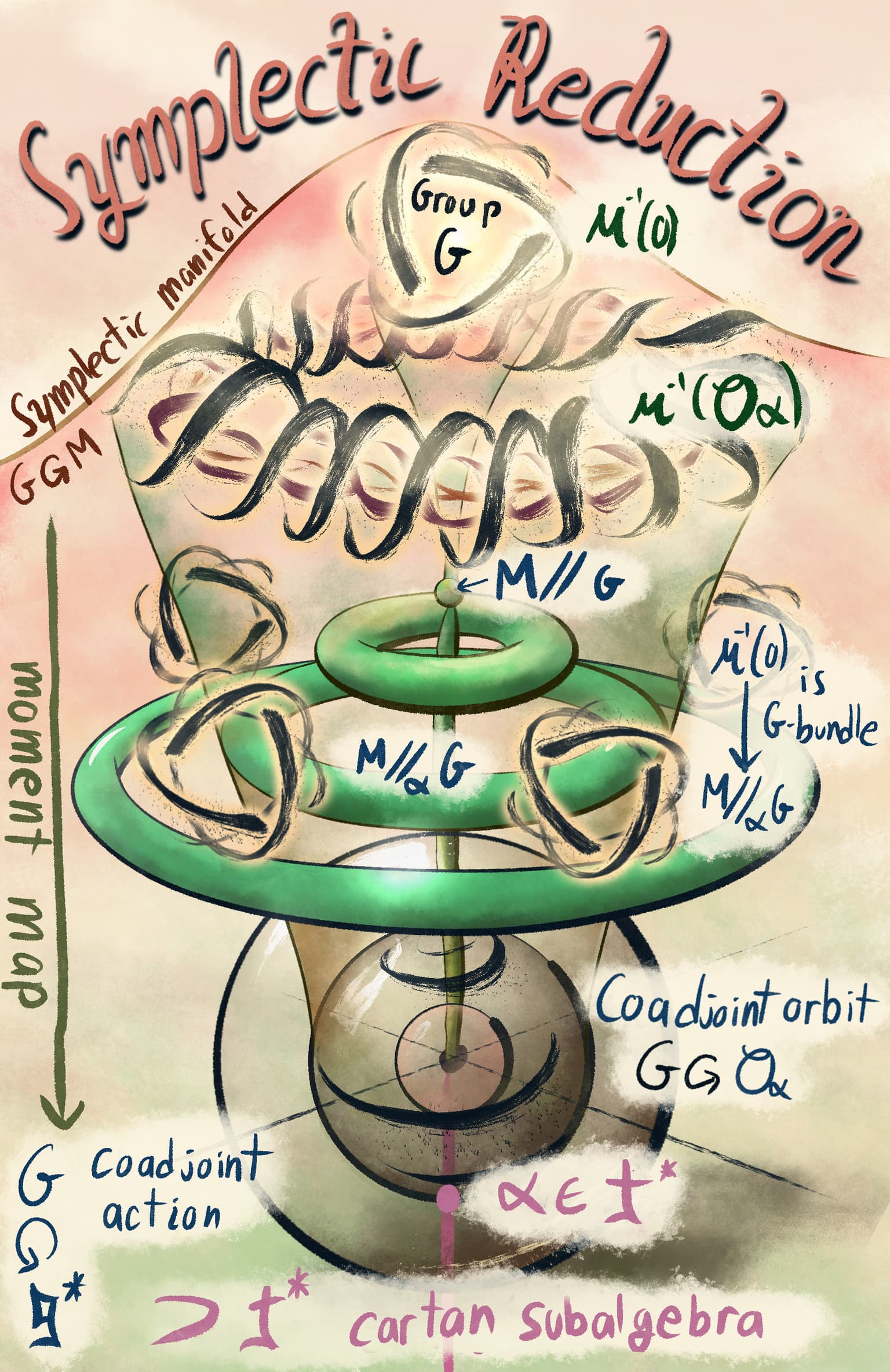
Anatomy of a symplectic manifold
45.0 x 40.0 cm
Digital illistration
2023