2024 Joint Mathematics Meetings
Henry Segerman, Saul Schleimer, Will Segerman
Artists
Henry Segerman
Associate Professor of Mathematics
Oklahoma State University
Stillwater, Oklahoma, USA
Saul Schleimer
Reader in Mathematics
Mathematics Institute, University of Warwick
Coventry, United Kingdom
Statement
Saul is a geometric topologist, working at the University of Warwick. His other interests include combinatorial group theory and computation. He is especially interested in the interplay between these fields and additionally in visualisation of ideas from these fields. Henry is an associate professor in the department of mathematics at Oklahoma State University. His research interests are in three-dimensional geometry and topology, and in mathematical art and visualization. He wrote the book "Visualizing Mathematics with 3D Printing". Will is a self-employed sculptor in analogue and digital media. He works from his home/office/workshop in Manchester, UK. His current main source of income is as an escape room puzzle designer.
Artworks
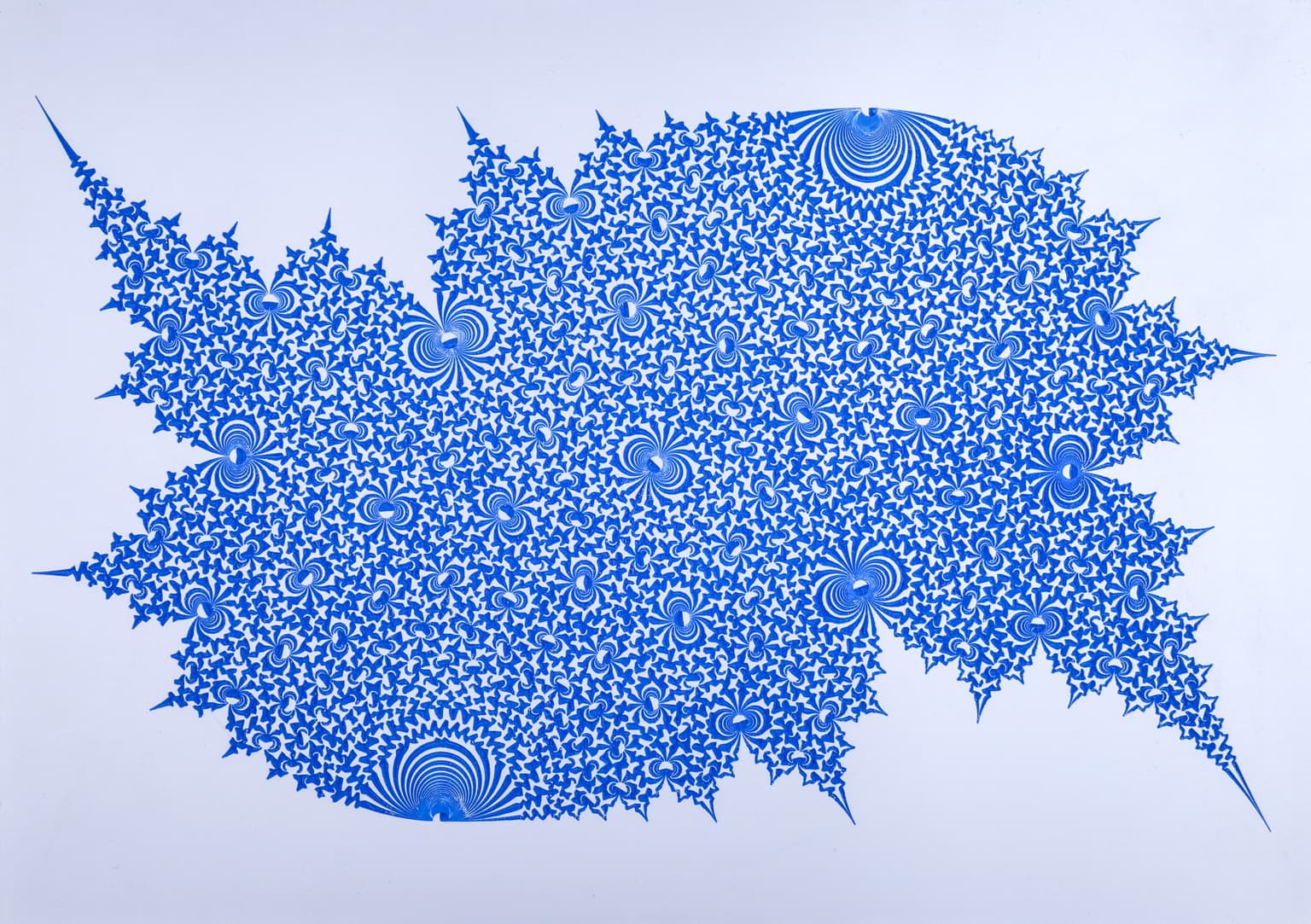
Cannon-Thurston map for the complement of the figure-eight knot
30.0 x 42.0 cm
Three-axis CNC milling (with a 60 degree v bit) of multilayered coloured PVC plastic
2022
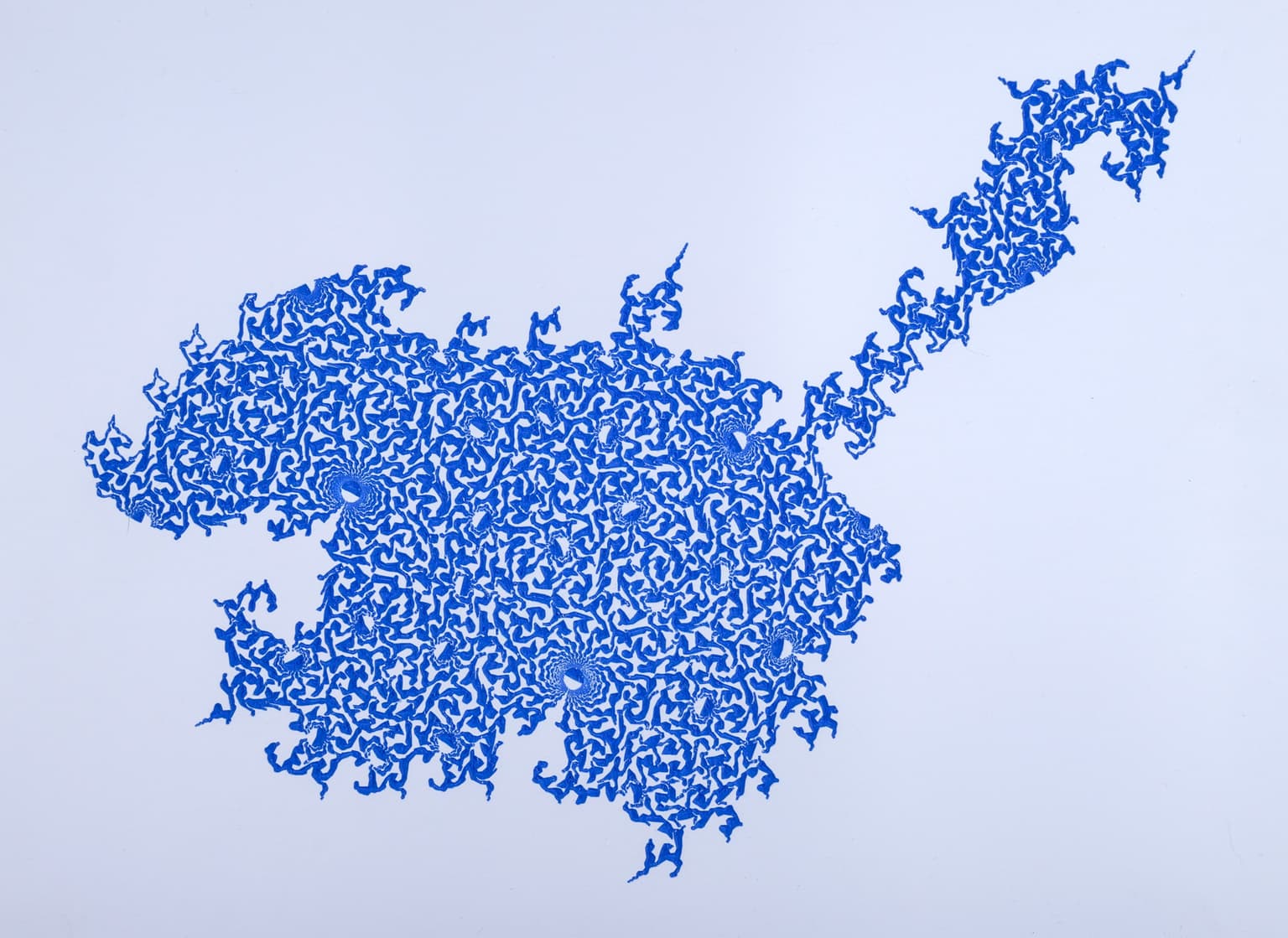
Cannon-Thurston map for the SnapPy manifold s227
30.0 x 42.0 cm
Three-axis CNC milling (with a 60 degree v bit) of multilayered coloured PVC plastic
2022