Frank A. Farris

Statement

My hope is to help others to see mathematics in new ways, sometimes inspired by technology, sometimes by the mathematics itself. For this work, I discovered that Geogebra, a wonderful tool for exploring geometry, can export images in postscript code that allows me to play with vector graphics, not just bitmaps. This year, the Problem of Apollonius came up in another project and I decided to offer some examples of what it looks like to find the eight (or fewer) circles that are tangent to three given circles, using conic sections as a tool. Artists and number theorists have made amazing works with Apollonian circles packings, where the starting point is three mutually tangent circles; I enjoyed illustrating some of the other possible cases.

Artworks

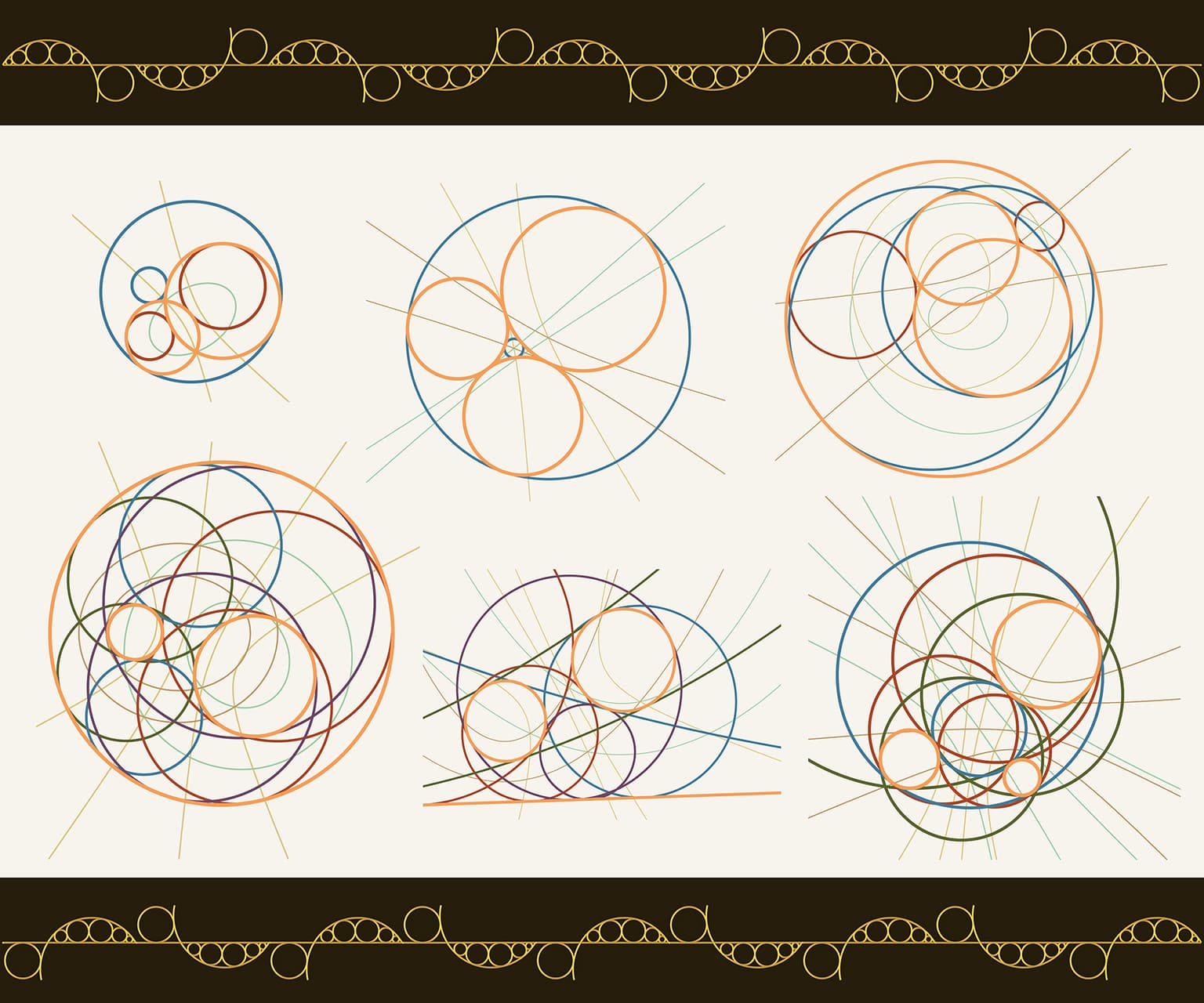
The Problem of Apollonius starts with three given circles and asks how many circles can be constructed to be tangent to all three. This piece illustrates the role of conic sections in finding as many as 8 tangent circles. The given circles are always in orange, with heavier line weights. At the top left is a warm-up: With just two given intersecting circles, an ellipse and a hyperbola hold all the centers of all the circles tangent to both; I picked four points on the conics and made the circles. In the remaining figures, find the given circles and enjoy how the centers of the bold constructed circles are triple intersections of the fainter conics.