2025 Joint Mathematics Meetings
John W Snow
Artists
John W Snow
Professor of Mathematics
University of Mary Hardin-Baylor
Belton, Texas, USA
Statement
Each of these images is a mathematical reflection of the 1923 painting "Circles in a Circle" by Vasily Kandinsky. Random compositions of trig functions, maximum, minimum, and multiplication are used to generate functions from the canvas to Kandinsky's painting. Each of these images is an inverse image of Kandinsky's painting under such a function. The images inherit symmetry and rhythm from the generated functions. They inherit color and texture from the painting.
Artworks
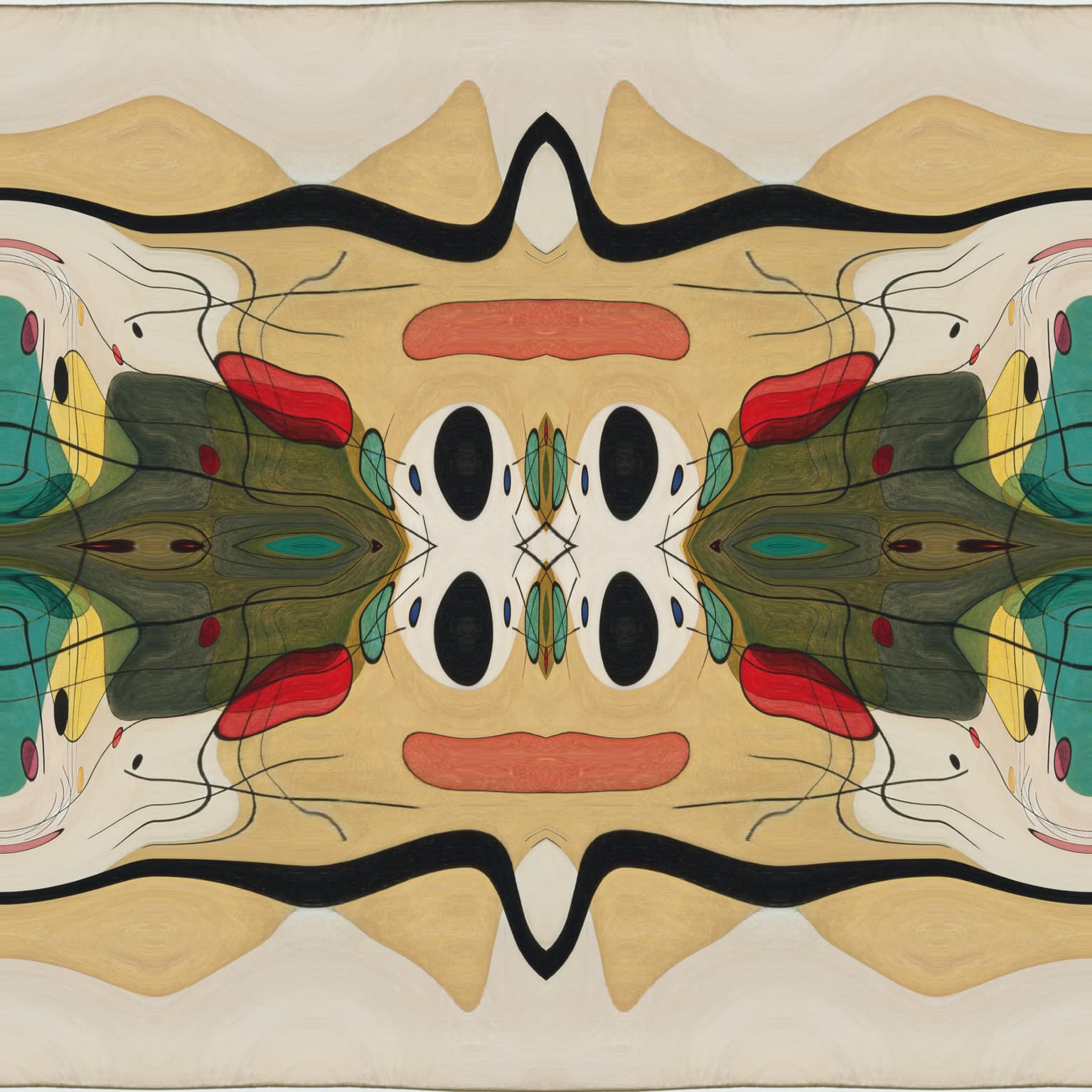
Inverted Circles 1
28.0 x 28.0 cm
Digital print
2024
The mathematical reflection procedure converts Kandinsky's simple combination of geometric figures into a more organic form in this image. Kandinsky's vibrant colors are still present, but lines and circles have been converted into smooth flowing curves.

Inverted Circles 2
28.0 x 28.0 cm
Digital print
2024
This piece is another mathematical reflection of Kandinsky's "Circles in a Circle". The reflection function in this image preserves much of Kandinsky's color palette but contributes a sense of movement and a regular rhythm to the resulting image.