2025 Joint Mathematics Meetings
Margaret Kepner
Artists
Margaret Kepner
Independent Artist
Washington, District of Columbia, USA
Statement
I like to explore the possibilities for conveying ideas in new ways, primarily visually. I have a background in mathematics, which provides me with a never-ending supply of subject matter. My lifelong interest in art gives me a vocabulary and references to utilize in my work. I enjoy expressing mathematical concepts through attributes such as color, geometric forms, and patterns. A topic I have explored recently is the Bell Numbers from Number Theory. The nth Bell Number indicates the number of different ways to group n elements into sets.
Artworks
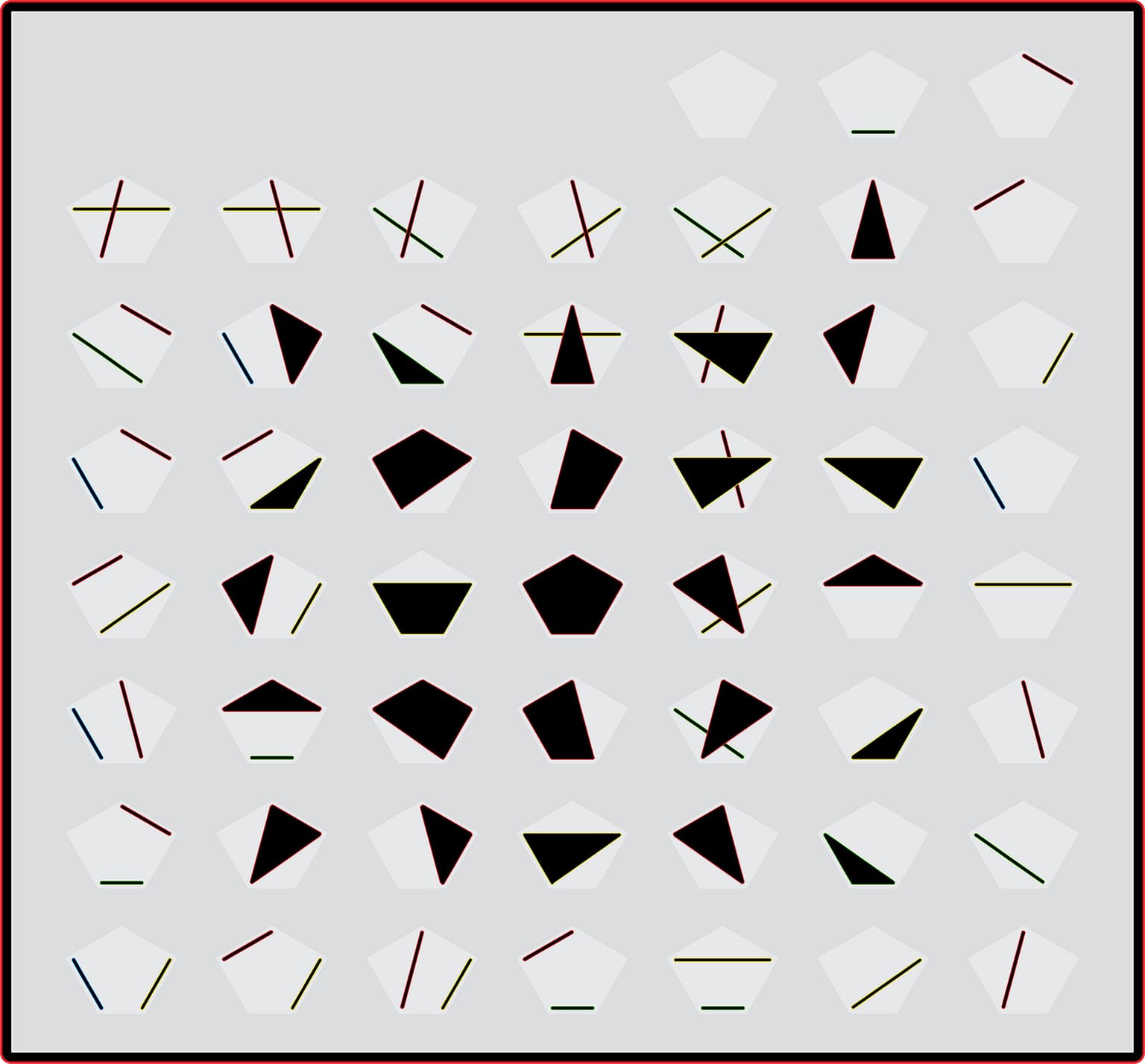
A Geometric View of the Fifth Bell Number
50.0 x 40.0 cm
Archival Inkjet Print
2024
The fifth Bell Number is 52, since there are 52 different ways that five elements can be partitioned into discrete, non-empty sets. In this piece, the solutions are considered geometrically, such that elements act as end-points or vertices. Each solution has a corresponding geometric figure. For example, if the five elements are denoted: a, b, c, d, e, then [(a, b, e), (c, d)] is one of the 52 solutions, with 3 elements in one subset and 2 in the other. Distributing the elements clockwise around a pentagon (top vertex labeled “a”) and then connecting sequentially within the subsets, generates a figure composed of a triangle and a line segment, as seen at row 6, col 2. Subtle color effects illustrate other properties of the figures.