2025 Joint Mathematics Meetings

Rebeca Regina Bittencourt Campusano

Artists

Regina Bittencourt

Mathematical Artist

MuArt Mujeres en el Arte; APECh; ESMA

Santiago, Chile

Art.RBittencourt@gmail.com

Statement

Fascinated by mathematics and technology, Chilean artist Regina Bittencourt has developed her career in the field of information technology. After exploring many materials, formats and media, Regina got interested in abstract art that builds on lines, curves, algorithms, surfaces, structures, polynomials and other entities, in order to develop a Mathematical Art Work as an artistic expression. She imposes herself the difficult challenge of making art based solely on mathematical concepts to show the beauty of Math Art. This concept has taking her art to more than 20 countries: The Netherlands, South Korea, Finland, France, USA, Bangladesh and China, among other places.

Artworks

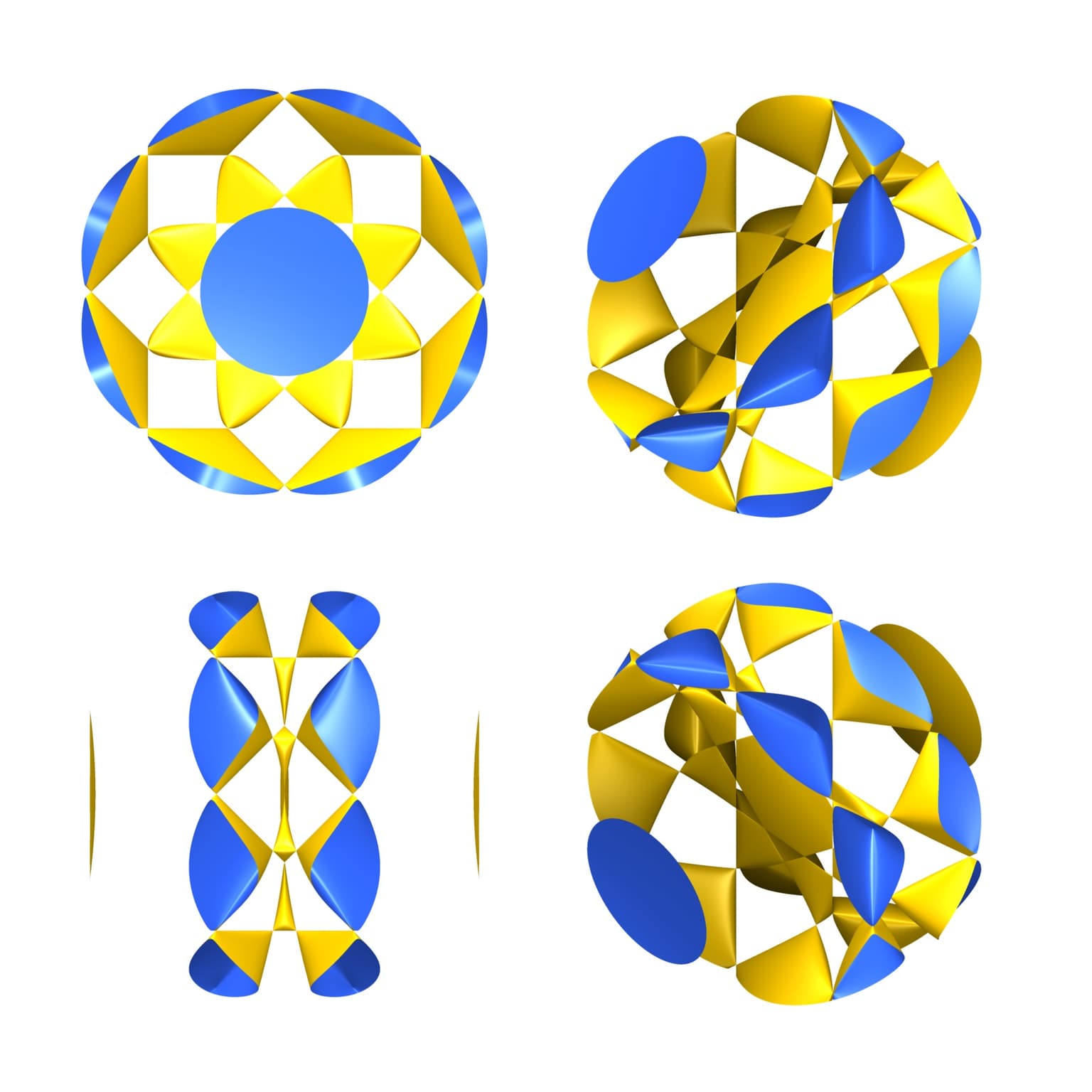
Image for entry 'Look at the Endrass Flower!'

Look at the Endrass Flower!

60.0 x 60.0 cm

Giclée on Canvas

2024

I use the octic Endrass surface polynomial equation: $$ 2a(-1/4(1-\sqrt(2)) (x^2+y^2)^2 +(x^2+y^2) ((1-1/\sqrt(2))z^2 +1/8(2-7\sqrt(2))) -z^4$$ $$+(0.5+\sqrt(2)) z^2-(1)/(16)(1-12\sqrt(2)))^2 $$ $$ -(cos(0\pi /4)x+sin(0\pi /4) y-1) (cos(\pi /4)x+sin(\pi /4)y-1) (cos(2\pi /4)x+sin(2\pi /4)y-1) $$ $$ (cos(3\pi /4)x+sin(3\pi /4)y-1) (cos(4\pi /4)x+sin(4\pi /4)y-1) (cos(5\pi /4)x+sin(5\pi /4)y-1) $$ $$ (cos(6\pi /4)x+sin(6\pi /4)y-1) (cos(7\pi /4)x+sin(7\pi /4)y-1) $$ I started from the point of origin (0, 0, 0) of the Cartesian coordinates and rotated the surface 45° around its axes until returning to the original point. The image shows the Endrass surface at its origin, then rotated 45, 90 and 135°