2025 Joint Mathematics Meetings
Stephen J Trettel
Artists
Stephen J Trettel
Assistant Professor of Mathematics
University of San Francisco
San Francisco California, USA
Statement
When illustrating mathematics, I focus on illustrating low-dimensional geometric objects which embody both visual and mathematical beauty. My primary medium consists of 3-dimensional computer graphics, and I typically write a new program for each piece. I aim to capture each mathematical object in its natural environment, and provide a view of what it 'would really look like' were it possible to visit the abstract world that it calls home.
Artworks
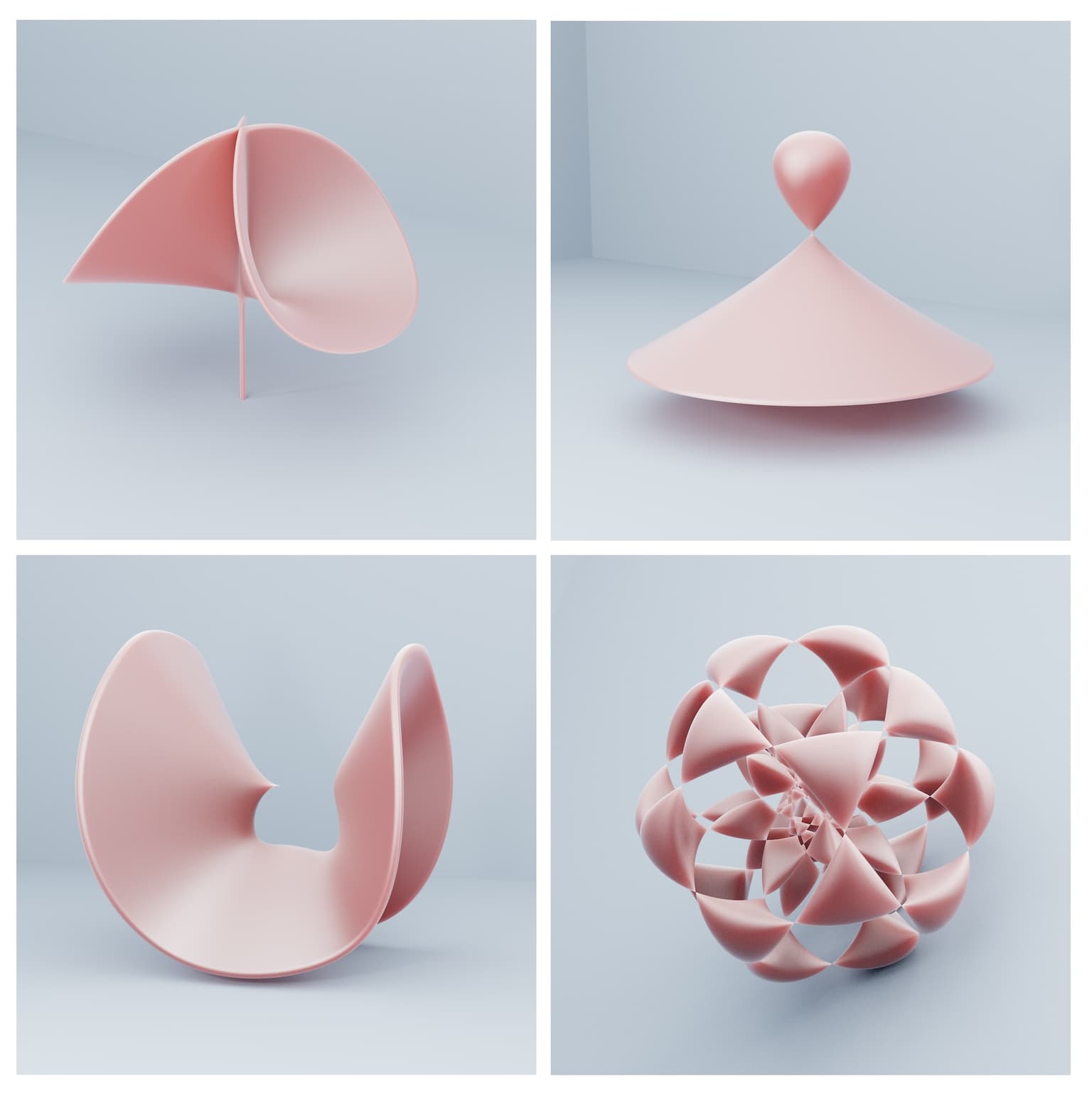
Singularity
20.0 x 50.0 cm
Digital simulation with custom software, printed on four square panels.
2024
Algebraic geometry emerges from the study varieties - curves, surfaces and higher dimensional analogs defined by polynomial equations. Some varieties are smooth (like the circle, $x^2+y^2=1$) providing a fruitful overlap with the differential geometric world. But general varieties also contain singularities - points near which they cannot be well approximated by a plane. This collection provides a window into the singular world, exhibiting three types of 2-dimensional singularities (a node, a cross-cap, and a cusp), as well as a variety containing many individual singularities (the double cover of the Barth Sextic surface, in the 3-sphere).