2025 Joint Mathematics Meetings
Susan Schmoyer
Artists
Susan Schmoyer
Lecturer
Mathematics and Statistics Department, The College of New Jersey
Ewing, New Jersey, USA
Statement
Like most mathematicians, I see math almost everywhere. When my kids got their first Spirograph sets and asked me what combination of gears would make a 5-pointed star, it was only inevitable that I would dig deeper into the mathematics of epicycloids and hypocycloids (the mathematical name for Spirograph curves). Math has found its way into other parts of my creative life, too. I enjoy knitting Moebius bowls, embroidering temari balls, and it’s only a matter of time before I start sewing math-inspired quilts.
Artworks
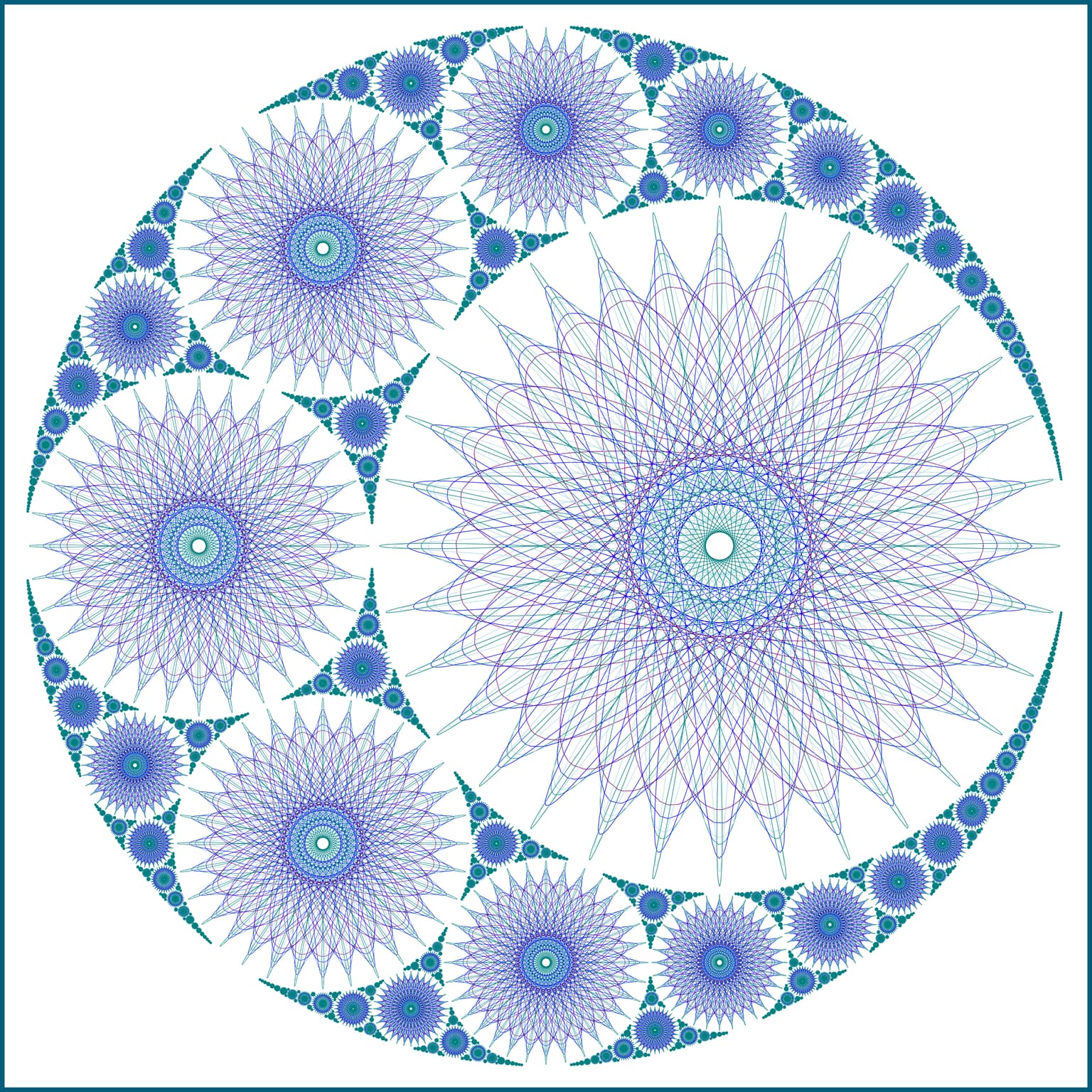
Blue Zinnia Apollonian Gasket
30.0 x 30.0 x 0.0 cm
framed print
2024
What do you get when you cross a hypocycloid with an Apollonian gasket? An Apollonian gasket is a fractal that begins with three circles, each one tangent to the other two. The spaces between the circles are filled in with new circles, each one tangent to three existing circles. The process is repeated ad infinitum. Hypocycloids are “Spirograph” curves that are created by tracing a point that is a fixed distance from the center of one circle, and rolling that circle around the inside of another fixed circle. They can be described using parametric equations. By overlapping hypocycloids you can create intricate designs. I used Sage to replace the circles of an Apollonian gasket with Spirograph designs.
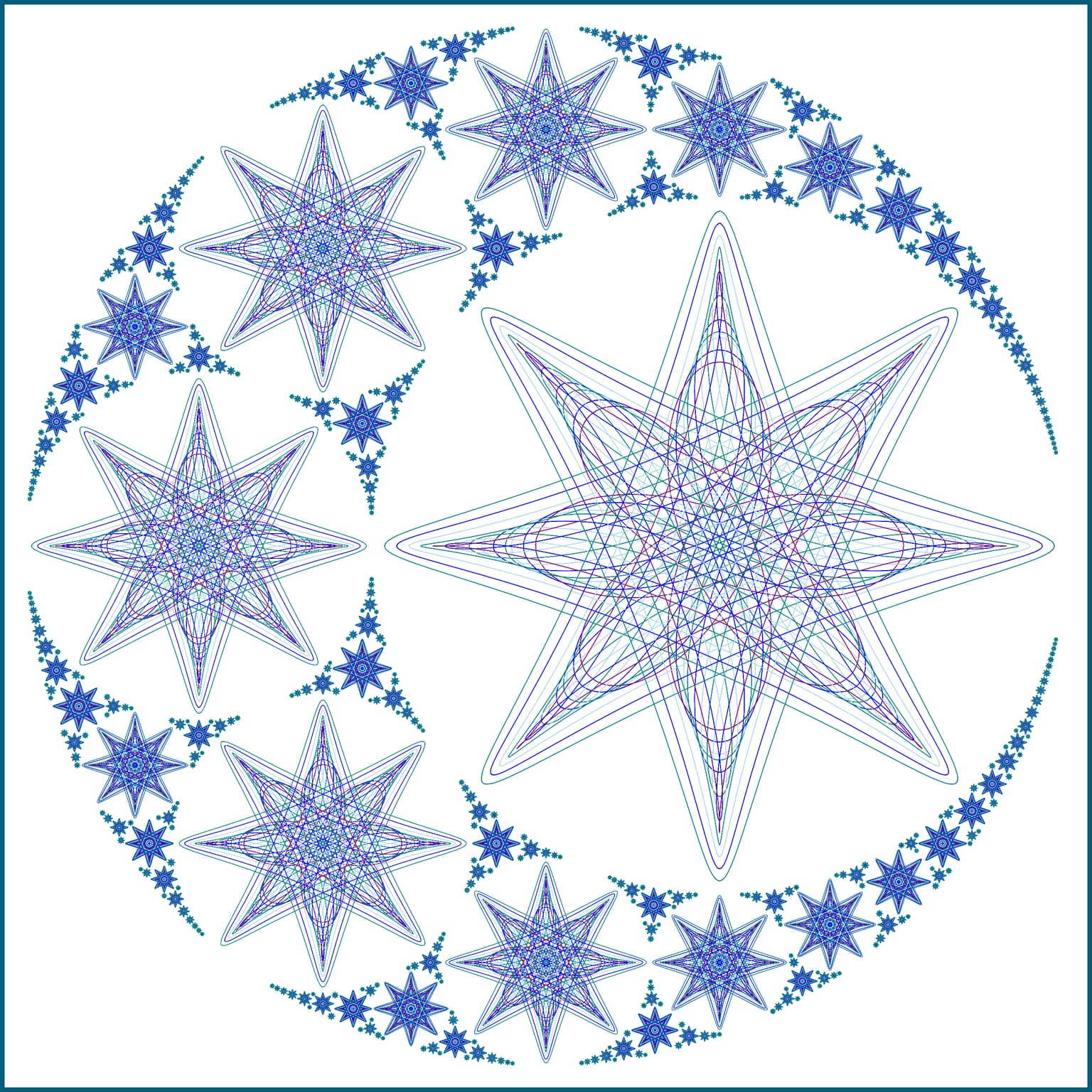
8-Pointed Stars Apollonian Gasket
30.0 x 30.0 x 0.0 cm
framed print
2024
What do you get when you cross a hypocycloid with an Apollonian gasket? An Apollonian gasket is a fractal that begins with three circles, each one tangent to the other two. The spaces between the circles are filled in with new circles, each one tangent to three existing circles. The process is repeated ad infinitum. Hypocycloids are “Spirograph” curves that are created by tracing a point that is a fixed distance from the center of one circle, and rolling that circle around the inside of another fixed circle. They can be described using parametric equations. By overlapping hypocycloids you can create intricate designs. I used Sage to replace the circles of an Apollonian gasket with Spirograph designs.