Bridges 2024 Exhibition of Mathematical Art, Craft, and Design
Benjamin G. Thompson
Artists
Benjamin G. Thompson
Ph.D. Student
Department of Mathematics, Cornell University
Ithaca, New York, USA
Statement
I've always loved mathematics, patterns, and bold colors. While I predominantly do research, I regularly think about how to bring advanced mathematics to wider audiences too. Making art out of mathematical tools and ideas is a part of this practice, as I do below with some recently developed objects from algebraic geometry. Most people in the world have no idea what "bumpless pipe dreams" are (nor do they need to!), but I hope my work is able to provide a wordless introduction to them nonetheless. I had a lot of fun doing the mathematics required to make this artwork, as well as the creative choices that followed in assembling it. Working with bumpless pipe dreams in this way was something new for me, hopefully it will be for viewers too.
Artworks
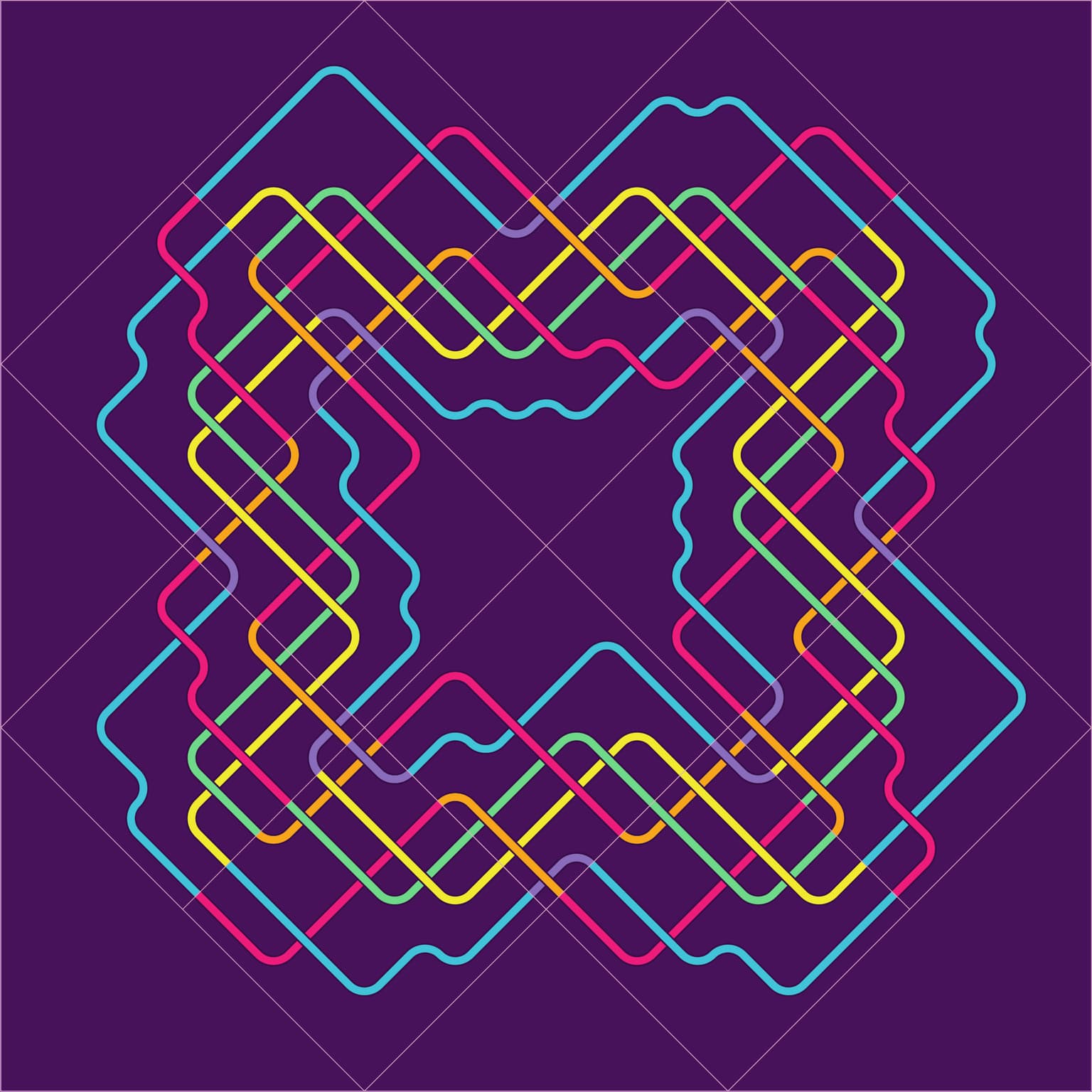
(125634) Dreams
32.5 x 32.5 cm
Digital print
2024