Artists
Logan Apple
Software Engineer
Seattle, Washington, USA
Statement
I'm Logan Apple, a Caltech graduate who loves creating fractal art. My artwork focuses on composing mathematical transformations in iterated function systems, painting them with colorful gradients, and rendering infinitely scalable designs. I've been exploring the intersection of math and art for over 10 years, tying together themes of nature, space, and geometric patterns. I love bringing math to life in vibrant images with elements that tie into the real world! I often address pressing environmental issues and make them the foci of my pieces. I've also previously hosted presentations on dynamical systems and created tutorials to teach others how to make their own fractal art.
Artworks
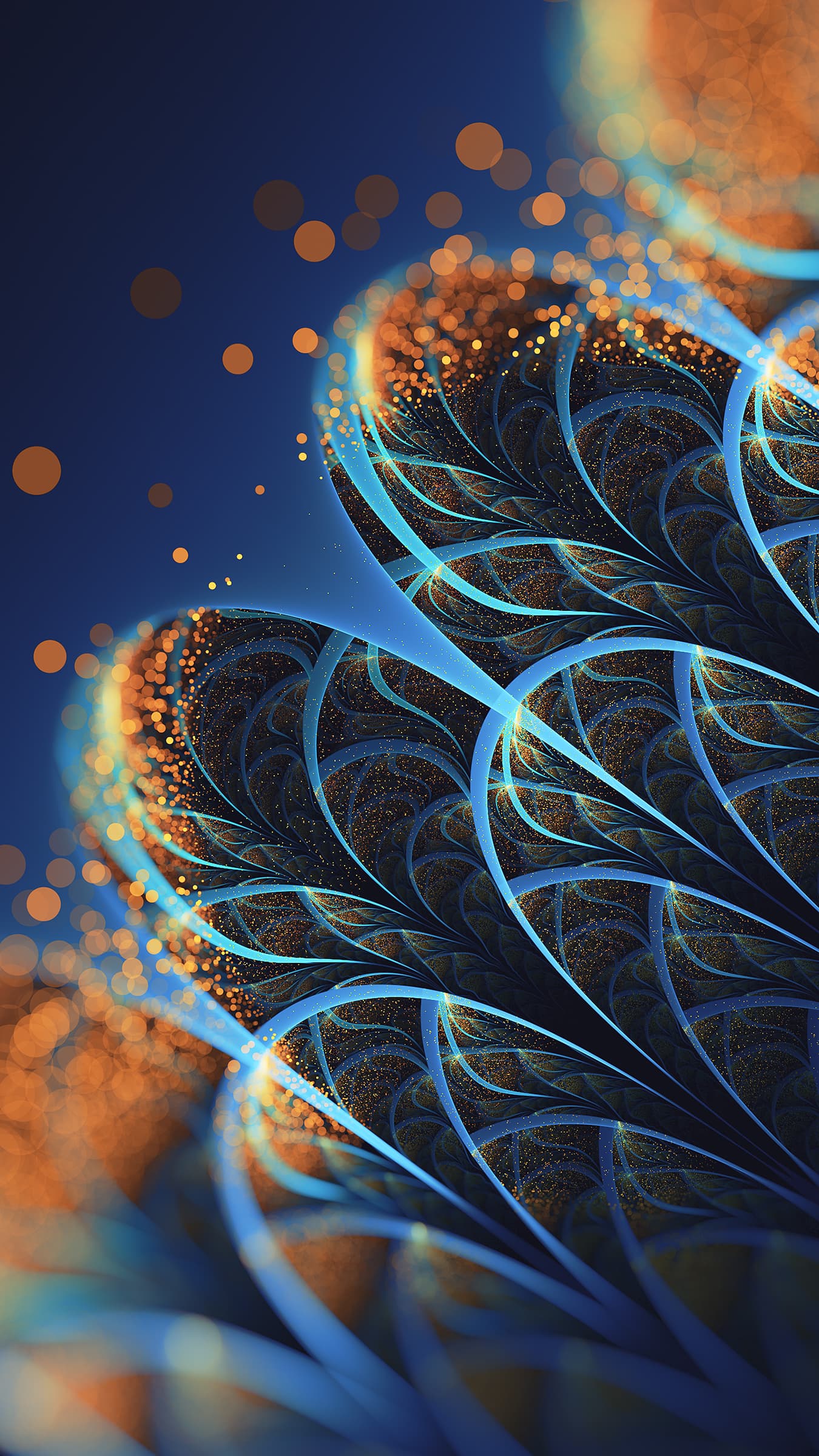
"Alluring Pollen" is an iterated function system (IFS) fractal. Iterated function systems are finite sets of contraction mappings on a complete metric space. Thanks to Banach's Contraction Mapping Theorem, there exists a fixed attractor which we'll converge to in any such set of functions. By playing the chaos game, we can render an image of this attractor over time.
The main body of the piece is an elliptic split, with an additional x-split and a cylinder transformation. The canvas itself is logarithmic with a Julia pre-transformation which gives the piece its distinct rounded curves descending in a neat row. Additional detailing comes from crackle and depth blur transformations, creating "pollen" and giving the piece its title.