Artists
Statement
I have been designing various origami works for several decades. A project delights me the most when it involves math and puzzles. I love the challenges of purism in origami, which means no cutting, gluing, nor painting. Here I present two types of my recent origami puzzles. First, a completed set resembles an existing puzzle in the world, yet in a paper-folded form. A good example is “$ Origami Einstein Hat Tiling.” Secondly, a model is presented with edges shown on the surface, instead of drawn lines, as the solution of a puzzle. “13 Convex Tangram Solutions” exemplifies the attempt. I gain much satisfaction in creating these works. After all, coming up with a design was a puzzle solved!
Artworks

This 13-sided, hat-shaped polygon is the first known example of an “einstein”, discovered by David Smith in November 2022. An “einstein”, meaning “one stone” in German, is a single tile that can be assembled on a flat surface endlessly without gaps, overlaps, and most amazingly, without repetitions. In this work, each tile here is a folded US dollar bill, without cuts. Among them, 45 tiles show the green side of the bills, with words “In God” visible. The other mirrored six show the black side of the bills, with President Washington peeking. Can you identify them?
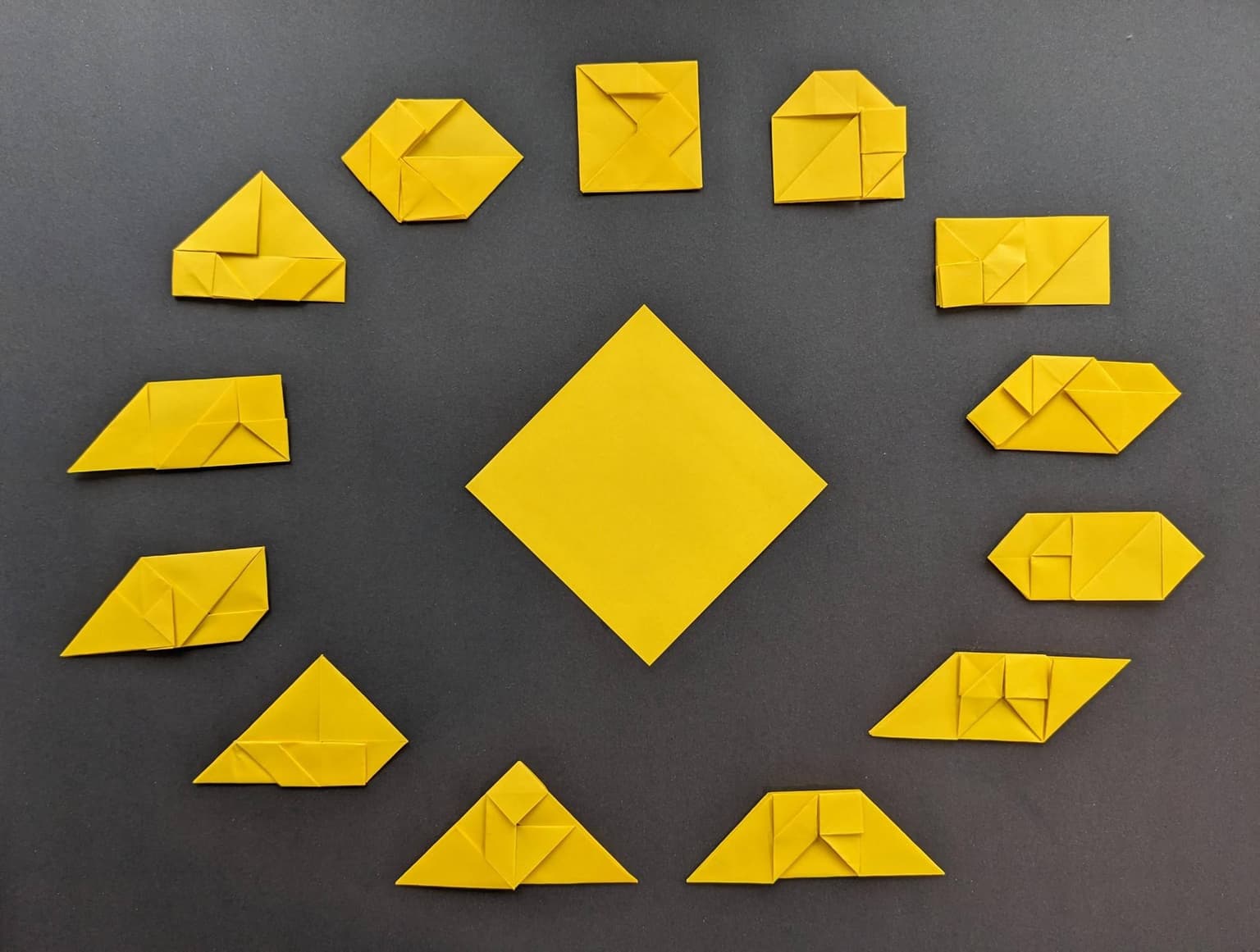
The seven-piece tangram tiling is a combination of 2-D geometric pieces when the edges of the adjacent polygons align with each other. There are only 13 convex polygonal shapes among all possible tiling arrangements. All of them are presented here. Each of the 13 pieces was folded from a single sheet of square paper, as shown at the center of the framed work, with no cutting. All visible edges, including folded ones and those from the original piece of paper, give out the solution of the tiling puzzle, without exceptions. This is the first origami design series that tackles the puzzle from this unique approach.