Anne Burns
Artists
Anne Burns
Professor Emerita (Mathematics)
Long Island University
Huntington, New York, USA
Statement
I have always been fascinated by the connections between mathematics, art and nature. I began my studies as an art major, but later switched to mathematics. After I bought my first computer in the late eighties I spent all my spare time programming, trying to use mathematical ideas to imitate art and nature.
Artworks
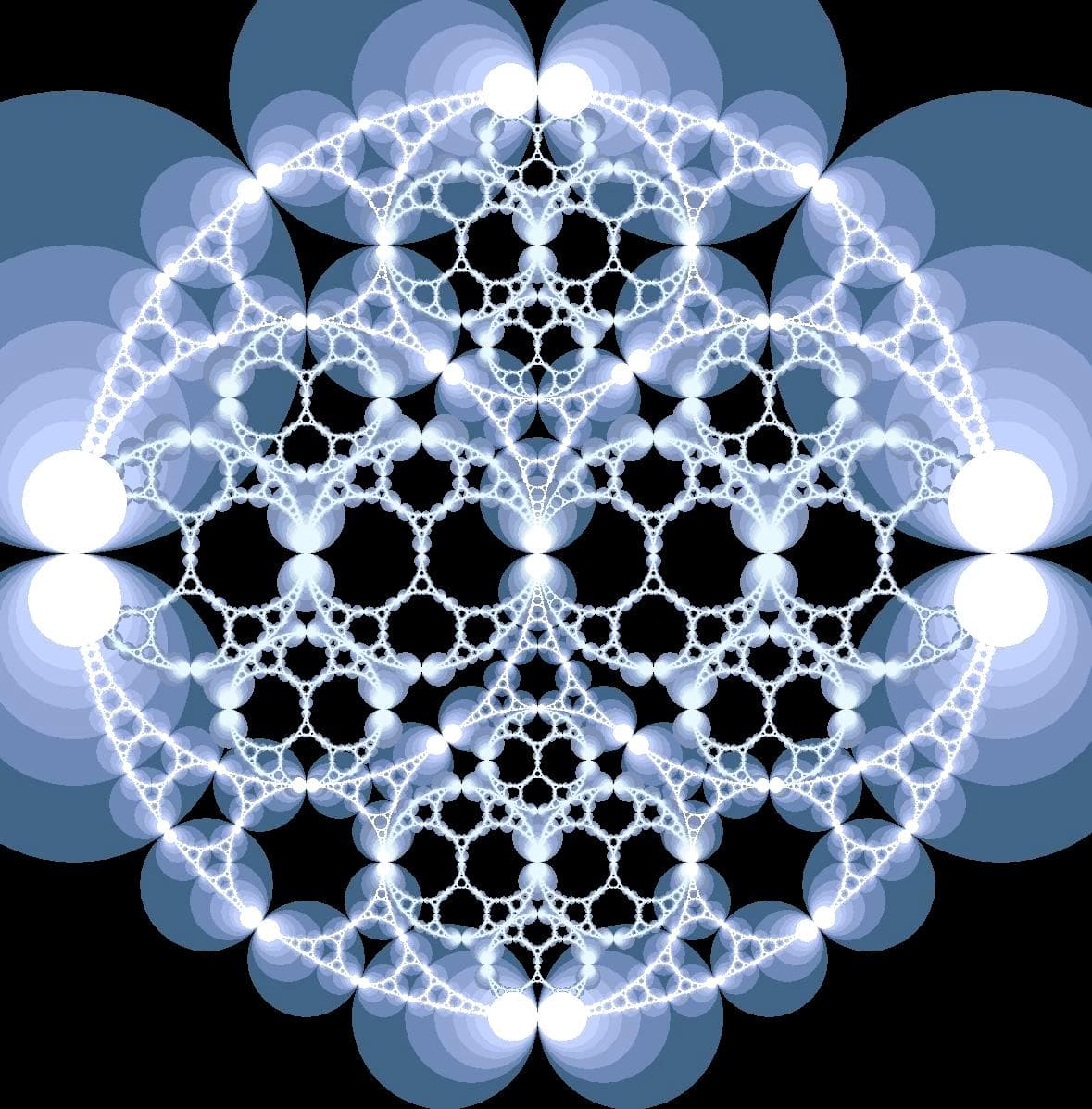
Appolonian Gasket
12"X 12"
Digital print
2012
An Appolonian Gasket is constructed as follows: starting with any three mutually tangent circles the two circles that are tangent to the original three are added.. At the next stage 6 new circles are added, each one tangent to three of the circles from the previous stage.. Continuing in this pattern; at each stage, for every triple of circles, new circles tangent to each of the three are constructed.

Organic Forms
16"X12"
Digital print
2012
In my 2011 Bridges paper I described a method of creating interesting designs by attaching vectors to curves in the plane. In this picture a vector f(z), for a function f of a complex number is attached to the points on the graph of an eight-leaved rose. The vectors are semi-transparent and rendered over a background of computer generated vines. The color and value of a vector is a function of its slope.

Imaginary Garden
14" X 11"
Digital print
2007
The clouds are the result of a midpoint algorithm. The flowers and leaves are generated using string rewriting sysems. The trees are fractals with controlled random lengths and angles designed to avoid regularity. A blade of grass is a sequence of very short lines whose slopes and lengths vary randomly.