Rodney Fulton
Artists
Statement
Geometric shapes intrigue me, especially overlapping ones. As do things composed of just a few elements. I don’t know why. I can only speculate. I am also interested in things that are asymmetrical yet balanced. It’s all very intuitive to me, and I’m comfortable with that. I think that we’re aware of many things that we don’t know we’re aware of. For instance, I’m not a mathematician yet my work demonstrates many mathematical concepts. Upon reflection, this really isn’t surprising. Math is everywhere and it’s part of everything, including us.
Artworks
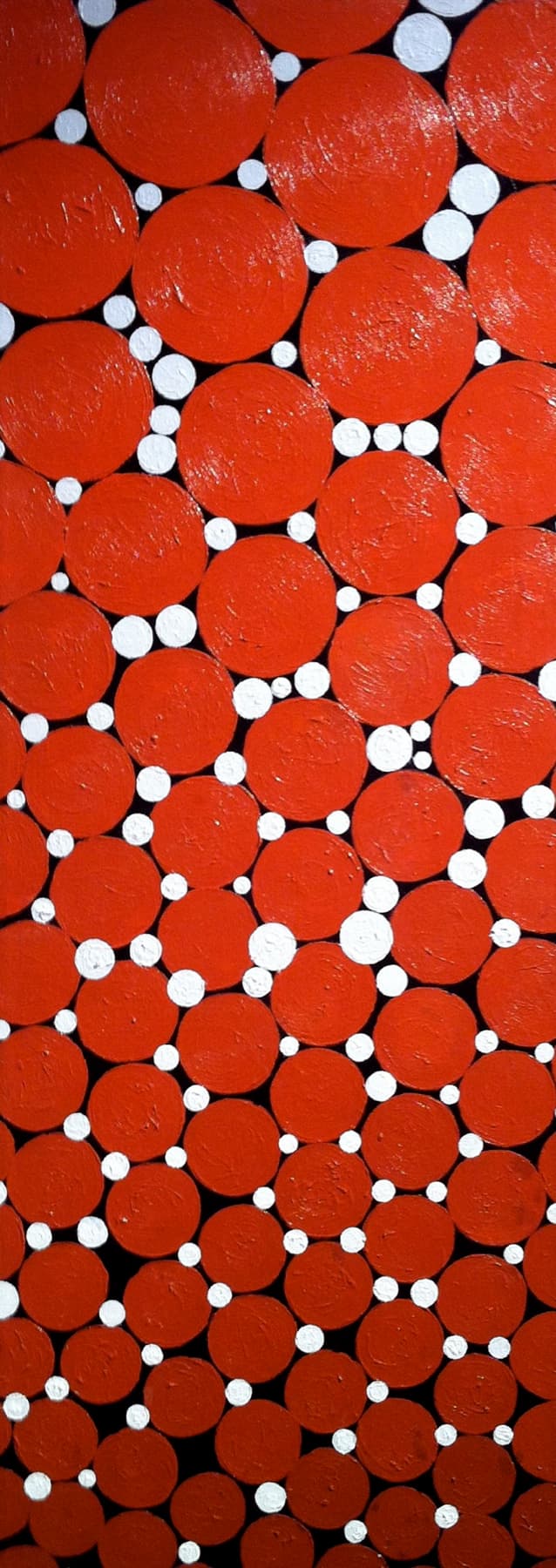
Circles
8" W x 24" H
Acrylic on canvas
2008
[ROD FULTON] Movement created with a simple repeating shape. The orange cirlces get bigger as they rise, like bubbles. The white circles were added afterwards because the painting wanted them. [MATHEMATICAL ANALYSIS BY DR. TATYANA SOROKINA] Classical circle packing problem: given a container (e.g. a rectangle) find the best arrangement of arbitrarily sized circles inside of the container. The number of circles might be fixed, in which case a measure of quality of packing needs to be introduced. Alternatively, one could aim for an arrangement that would accommodate the most number of circles. This piece is a variation of the traditional circle packing problem where we are given circles of different radii, however, larger circles have higher weights in the final count. In general, this is an unsolved problem. Adding colors takes us to the three-dimensional analog of this problem called sphere packing . It is used in granular structure modeling, where colors reflect different grains.

Cityscape
30" W x 40" H
Acrylic on canvas
2011
[ROD FULTON] This is an example of interlocking geometric shapes and asymmetry and balance. The buildings are one of the things that overlapping rectangles suggest to me. When the buildings are taken as a whole, the whole piece has only three pieces: the skyline, the moon/sun and the sky. Nothing is centered, yet the whole thing feels right. [MATHEMATICAL ANALYSIS BY DR. TATYANA SOROKINA] Cityscape is an example of the approximation of a skyline of a city. It is a non-trivial problem since the skyline is discontinuous. Most numerical analysts agree that L1 norm should be used. The solution is given by piecewise linear discontinuous function, known as a discontinuous spline. Cityscape attempts to show a possible solution to a more general problem – approximation of a cityscape. It uses the idea of “multiple skylines”, adding colors to bring out features of the cityscape. The non-centered source of light helps to “smooth” the corners of the cityscape.

Garden
48" W x 36" H
Acrylic on canvas
2005
[ROD FULTON] This is an example of overlapping geometric shapes, simplicity, and asymmetry and balance. There are only four “thingss” in the piece: the sun the tree, the land and the sky. They all seem to be in the right place. [MATHEMATICAL ANALYSIS BY DR. TATYANA SOROKINA] This is is an example of multicolored Voronoi diagrams of higher order. Traditional multicolored Voronoi diagrams consist of polygonal regions of different color. The boundary of each cell is a piecewise linear curve. Voronoi diagrams of higher orders as well as Voronoi diagrams based on a non-Euclidian metric allow using curves of higher order. Second order curves have been used, along with the coloring of each Voronoi cell to reflect sophisticated shapes and color modes of nature.