Teresa Downard

I was motivated to make this piece after I found a mentor through the Association of Women in Mathematics and in response to some of the math and gender discussions in the last couple years. It is likely that each female mathematician inspires atleast two young women, in my opinion having these role models will attract women with aptitude to the field in a natural way.

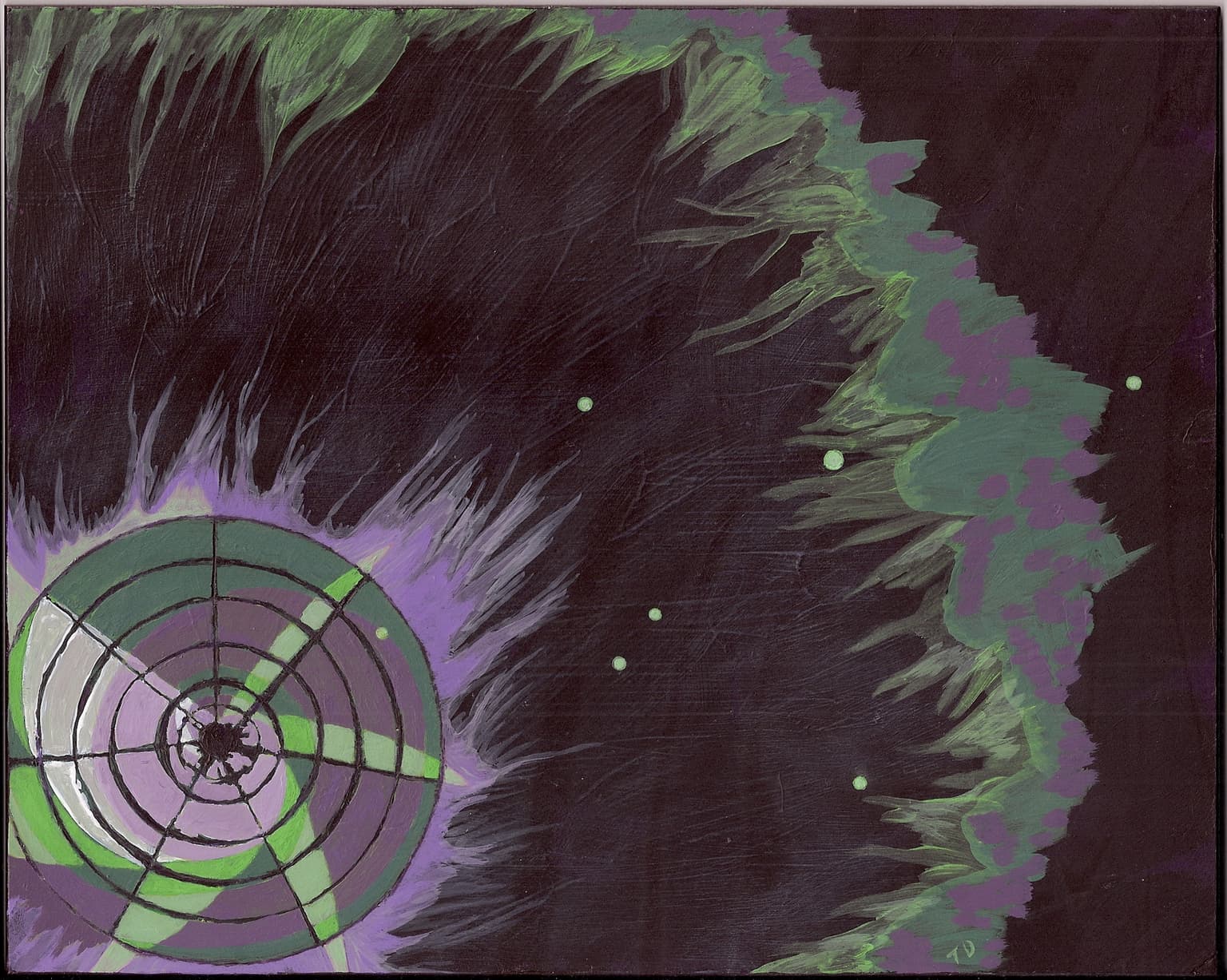
A depiction of the cyclic group Z_7 with addition modulo 7. The figure on the lower left can be seen as a coordinate grid. The zero map, identity map, and others are shown with colors that intersect the grid lines. This work was inspired by study of finite groups, and was created with the intention of using symbolism to communicate mathematical ideas.

The set of all neighborhoods using the distance function d(A,B)=|AunionB - AintersectB| where A and B are finite sets. Here we are defining a distance between sets by the number of unshared elements. If we think of the typical drawing of intersecting sets, we can redraw this with the 'outside' set as an annulus. They are arranged like a stack of coins that has slid over, where width of the border corresponds to the number of elements. When studying introductory analysis, a classmate came up with this interesting way to define distance. For my visualization I employed wet mixing to mimic natural texture, and hoped to imbue it with some of what I feel makes abstract art compelling: simplicity that emphasizes the beauty of relationships between color, form, and texture.